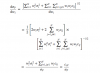Difference between Marginal and incremental VAR

[CYLoh]

Hi

I'm somewhat confused between Marginal and Incremental VAR. Am i right to say that the only difference between the two is that Incremental VAR is calculated precisely from a total revaluation of the portfolio and that Marginal VAR is just an estimation of the change in VAR?

Regards

 

[ShaktiRathore]

Yes
Incremental Var for a position gives u the Var change of portfolio resulting from removing the position a from the portfolio.Simply ,Var(P+a)-Var(P) =Incremental Var, value Var of portfolio including position a, now remove position a from from portfolio and revalue portfolio P Var the resulting change in Var is incremental Var.
While Marginal Var for a position is derivative of portfolio w.r.t the per unit $ change in value of the position is dP/da. It answers the question how much portfolio Var changes w.r.t every $ change in value of position. If position value change by small value da then resulting change in portfolio Var is marginal Var. If position changes by infinitsimal da $ then resulting change in Var$ of portfolio dP is the marginal Var.
Thanks

 

[dkmonline]

Hi

Incremental costs are closely related to the concept of marginal cost but with a relatively wider connotation. While marginal cost refers to the change in total cost resulting from producing an additional unit of output.

 

[Dipuo]

Thanks @ShaktiRathore - That explanation helped me because I was struggling with this.

 

[David Harper CFA FRM]

Just to add, how i think of it: Incremental VaR is the exact (fully simulated) answer to the change in VaR resulting from removal of the position. Marginal VaR, as a partial derivative, informs an linear approximation to removal--or just a change--in the position; i.e., marginal VaR gives us Component VaR which is an approximation. Marginal VaR is the convenience approximation; incremental VaR is an actual answer. A weak analogy would be: using duration to approximate a price change in bond; duration is the linear approximation (analogous to marginal VaR), whereas re-pricing would give us the accurate, exact answer (analogous to incremental VaR). Thanks,

 

[Stuti]

I have a question about Marginal VaR. Is it possible if someone could guide me how did we arrive at the derivation

Marginal VaR = Zc * cov ( Ri , Rp)/standard dev of Rp?

I am unable to arrive at this result. It would be great if someone could help me.

 

[Arka Bose]

Hi Stuti,

Cov(Ri,Rp)/sigma Rp is actually the beta between the portfolio and the asset i.
Since beta is the slop, i.e first derivative, that is why u have that formula

 

[vijayaraghavan sundararajan]

Hi Stuti,

Here goes the derivation;

Let;

p = Portfolio Value
standard deviation of portfolio (Rp) = s.d

Marginal Var = Var(p) /p * cov(i,p)/variance of Portfolio
= z* s.d * p/p * cov(i,p)/(s.d)^2

striking off the common terms;

the final formula gets to = z*cov(i,p)/s.d


Hope this helps.

Rg,
Vijay.

 

[afterworkguinness]

@ShaktiRathore , great answer. Helped me a lot. Thanks.

 

[Stuti]

Hi , I understood all the steps in the derivation of marginal VaR. However, how do we conclude that the numerator is covariance(asset (i) , portfolio)? @arkabose and @vijayaraghavan sundararajan - Thank you for your explanations. I can relate with beta which is a next step after arriving at the numerator & denominator as indicated in the file i have attached.

 

[vijayaraghavan sundararajan]

@Stuti I believe that we are trying to calculate the % of portfolio Variance that's been contributed by the asset, hence the covariance (asset, portfolio) is divided by the portfolio variance.

 

[ShaktiRathore]

Hi
If you can find this derivation helpful:
Marginal Var=d(Varp)/d(wi*Vp) is the as per definition where Varp is the Var of the portfolio and the wi*Vp is the $ invested in the ith position.
=>Marginal Var=d(z*sigma(p)*Vp)/d(wi*Vp)=(z*Vp/Vp)*d(sigma(p))/dwi (as z and V are constants per se so take them out)
=>Marginal Var=(z)*d(sigma(p))/dwi ....E1)

Also we know that, standard deviation of portfolio^2
=sigma(p)^2=w1^2*sigma(1)^2+....+wi^2*sigma(i)^2+....+wn^2*sigma(n)^2 +2*wi*(w1*Cov(i,1)+w2*Cov(i,2)+....+wn*Cov(i,n))+Other Covariance terms (considering that portfolio has n positions)
differentiate w.r.t wi both sides of above equation to get:
d(sigma(p)^2)/d(wi)=2 wi*sigma(i)^2+2*(w1*Cov(i,1)+w2*Cov(i,2)+....+wn*Cov(i,n))
2*sigma(p)* d(sigma(p))/d(wi)=2 (w1*Cov(i,1)+w2*Cov(i,2)+....+wi*sigma(i)^2+....+wn*Cov(i,n))
sigma(p)* d(sigma(p))/d(wi)=(w1*Cov(i,1)+w2*Cov(i,2)+....+wi*Cov(i,i)+....+wn*Cov(i,n))... E2) (as sigma(i)^2=Cov(i,i))

Also we know that portfoio return=Rp=w1*R1+w2*R2+....+wi*Ri+...+wn*Rn
=>Cov(Ri,Rp)=w1*Cov(R1,Ri)+w2*Cov(R2,Ri)+....+wi*Cov(Ri,Ri)+...+wn*Cov(Rn,Ri) (the (Ri,Rp) is same as(i,p) notation used above)
=>Cov(i,p)=w1*Cov(1,i)+w2*Cov(2,i)+....+wi*Cov(i,i)+...+wn*Cov(n,i)

Since from equation 2) w1*Cov(1,i)+w2*Cov(2,i)+....+wi*Cov(i,i)+...+wn*Cov(n,i)=sigma(p)* d(sigma(p))/d(wi)
=> sigma(p)* d(sigma(p))/d(wi)=Cov(i,p)

=>d(sigma(p))/d(wi)=Cov(i,p)/sigma(p) ...3)
Put value of d(sigma(p))/d(wi) in Equation 1) to get
=> Marginal Var=z*Cov(i,p)/sigma(p)
Thus Marginal Var=z*Cov(i,p)/sigma(p)
thanks

 

[Stuti]

Hi
If you can find this derivation helpful:
Marginal Var=d(Varp)/d(wi*Vp) is the as per definition where Varp is the Var of the portfolio and the wi*Vp is the $ invested in the ith position.
=>Marginal Var=d(z*sigma(p)*Vp)/d(wi*Vp)=(z*Vp/Vp)*d(sigma(p))/dwi (as z and V are constants per se so take them out)
=>Marginal Var=(z)*d(sigma(p))/dwi ....E1)

Also we know that, standard deviation of portfolio^2
=sigma(p)^2=w1^2*sigma(1)^2+....+wi^2*sigma(i)^2+....+wn^2*sigma(n)^2 +2*wi*(w1*Cov(i,1)+w2*Cov(i,2)+....+wn*Cov(i,n))+Other Covariance terms (considering that portfolio has n positions)
differentiate w.r.t wi both sides of above equation to get:
d(sigma(p)^2)/d(wi)=2 wi*sigma(i)^2+2*(w1*Cov(i,1)+w2*Cov(i,2)+....+wn*Cov(i,n))
2*sigma(p)* d(sigma(p))/d(wi)=2 (w1*Cov(i,1)+w2*Cov(i,2)+....+wi*sigma(i)^2+....+wn*Cov(i,n))
sigma(p)* d(sigma(p))/d(wi)=(w1*Cov(i,1)+w2*Cov(i,2)+....+wi*Cov(i,i)+....+wn*Cov(i,n))... E2) (as sigma(i)^2=Cov(i,i))

Also we know that portfoio return=Rp=w1*R1+w2*R2+....+wi*Ri+...+wn*Rn
=>Cov(Ri,Rp)=w1*Cov(R1,Ri)+w2*Cov(R2,Ri)+....+wi*Cov(Ri,Ri)+...+wn*Cov(Rn,Ri) (the (Ri,Rp) is same as(i,p) notation used above)
=>Cov(i,p)=w1*Cov(1,i)+w2*Cov(2,i)+....+wi*Cov(i,i)+...+wn*Cov(n,i)

Since from equation 2) w1*Cov(1,i)+w2*Cov(2,i)+....+wi*Cov(i,i)+...+wn*Cov(n,i)=sigma(p)* d(sigma(p))/d(wi)
=> sigma(p)* d(sigma(p))/d(wi)=Cov(i,p)

=>d(sigma(p))/d(wi)=Cov(i,p)/sigma(p) ...3)
Put value of d(sigma(p))/d(wi) in Equation 1) to get
=> Marginal Var=z*Cov(i,p)/sigma(p)
Thus Marginal Var=z*Cov(i,p)/sigma(p)
thanks

Thank you so much. This makes it clear!

 

[FrmL2_Aspirant]

Hi,
Someone asked this question on watsapp group, how to solve it ? @emilioalzamora1 any insight from you will be very helpful
tagging @bpdulog for notification

 

[emilioalzamora1]

Hi,

weight(HIJ): (140/300) = 0.466 which means Wealth(HIJ): 140
weight (KLM) (160/300) = 0.533 which means Wealth(KLM): 160
weight (portfolio) = 0.466 + 0.533 which means Wealth(portfolio): 140 + 160 = 300

std. dev. (HIJ): 20%
std. dev. (KLM): 12%
std. dev. (portfolio): 13.7%

beta (HIJ): 1.6
beta (KLM): 0.8
beta (portfolio): 1.2

1.) marginal VAR (HIJ) = alpha*ß(HIJ)*sigma(p) = 1.645*1.6*0.137 = 0.360584

2.) marginal VAR (HIJ) = [ VAR(portfolio)/Wealth(portoflio) ] * ß(HIJ) = [ (1.645*300*0.137)/300 ]* 1.6 = 0.360584

 

[Ali Ehsan Abbas]

Whay would be the result to portfolio diversified var? Shouldnt it be the product of Portfolio wealth, portfolio st. Dev., and the 1.645 deviate?

 

[FrmL2_Aspirant]

thanks a lot @emilioalzamora1 .. !

 

[emilioalzamora1]

Honestly speaking the diversified VaR question is not clear.

contribution of KLM:

VaR(portfolio) = 300*1.645*0.137 = 67.6095

Component VaR(KLM) = marginal VaR(KLM) *Wealth(KLM)

Marginal VaR(KLM) = 1.645*0.8*0.137 = 0.180292

Component VaR(KLM) = 0.180292*160 = 28.84672 >> in percent out of the total VaR(portfolio) = 28.84672/67.6095 = 42.67%

Diversified VaR (assuming rho = 0):

[ VaR1^2 + VaR2^2 ]^1/2

But this does not yield the given answer. Have no idea how to get 10M here. I think it is necessary to have @David Harper CFA FRM take on this.

 

[Ali Ehsan Abbas]

I have a feeling the inputs are incorrect as they (in the case of HIJ and portfolio) lead to correlation parameter estimate > 1. @David Harper CFA FRM will come to the rescue hopefully soon.

 

[David Harper CFA FRM]

Outstanding @emilioalzamora1 ! I agree with 100% of your calculations above. I would add:

• Given the portfolio volatility of 13.7%, we can infer the correlation param, ρ(HIJ, KLM), is 0.50
• The betas are probably not internally consistent (this is a common bug in these questions if they are not constructed from the bottom up). Related, our feedback to GARP is that betas should be labeled because in this application these are not CAPM betas. The HIJ beta is β(HIJ, Portfolio); ie, the beta of the position with respect to the portfolio. As such the Portfolio beta should be 1.0. And, by my quick calculations the internally consistent betas would be β(HIJ) = 1.33 and β(KLM) = 0.71.
• Nevertheless, using the given betas, I fully agree with @emilioalzamora1 's marginal VaRs; e.g., there are four ways to get there including σ(portfolio)*1.645*β, in this case 13.704%*1.645*[1.6 | 0.8] = [0.3606, 0.1803]
• Also agree the portfolio diversified VaR should be $30.0 * 13.7% * 1.645 = $67.6 million
• If we correct for the portfolio VaR, then the contribution of KLM (i.e., which is KLM's component VaR divided by portfolio VaR) becomes (0.1803*$160)/$67.6 = 42.67%, where KLM's component VaR = 0.1803*$160 = $28.85. So I would say that the only problems with the question are the internally inconsistent betas, and I don't think any of the portfolio VaRs are possible (consider that the highest choice of $21.5 mm is a VaR% of only 21.5/300.0 = 7.2%. Doesn't makes sense given the other facts). I hope that helps, thanks!