Difference between Marginal and incremental VAR

[GARP2015]

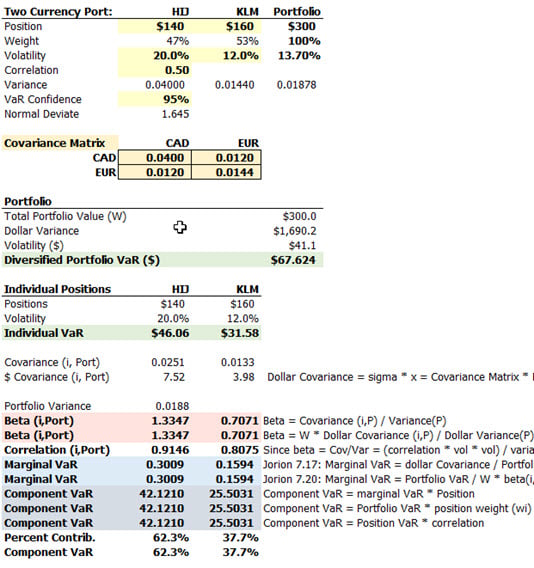
Thank you @Nicole Seaman that is exactly the relevant email: we only received it this week. We've been driving this point for years actually, so I am very happy to report that GARP finally understands the importance of a correctly specified portfolio VaR. Jamal (@GARP2015 ) you are exactly correct about an easy test for the betas; below is the specification that I sent GARP for this question. Notice that indeed (140/300) * 1.3347 + (160/1300) * 0.7071 = 1.00. Thank you!

Thanks David, I appreciate your response.
Actually, I have found the same inconsistency in 2010 practice exam and maybe more in other years, so since 2010 GARP are publishing wrong questions.
How many candidates failed FRM because of these questions? Remember that FRM practice questions are old real exams !!!
Finally, one more way to calculate component VaR contribution=(Beta*weight * Portfolio VaR), then you can calculate Marginal VaR by dividing CVaR over position value. In the example above and using correct Betas, Component Contribution of HIJ = 1.337 * 140/300 = 62.3%, the CVaR = 62.3%* 67.62 = 42.19, MVaR = 42.18/140 = 0.30.

It is easier to start with Beta ...

Jamal

 

[David Harper CFA FRM]

Hi Jamal (@GARP2015 ) Yes, exactly, believe you me that I am very unhappy about the low standard that has historically been employed on the portfolio VaR questions; I have supplied GARP with the correct approach to internal consistency many many times. It is very disappointing to me personally, especially given that it takes hard work to master these concepts. Candidates, who invest significantly their time and money, deserve correctly specified questions.

In regard to your alternative approach, yes, I do agree! You will note that my spreadsheet contains three approaches to Component VaR, the first approach is "Component VaR = marginal VaR * Position" and the second approach is "Component VaR = Portfolio VaR * position weight (wi) * beta (i,P)." So that's consistent with your approach above. Thank you!

 

[Biju]

Hi David

For the GARP FRM Part-2 2017 Qstn#60

Asset position Value (USD million) Return Standard Deviation (%) Beta(To Portfolio)
HIJ 200 30 1.30
KLM 300 25 0.55
Portfolio 500 20 1.0


----
For the above question

For the Diversification benefit can't I calculate without Marginal VaR ?

ie
Portfolio Var = 1.645*.0.2*500*=1645.5

VaR HIJ=1.645*.25*200=82.25
VaR KLM=1.645*.30*300=148.05
Total StandAlone VaR=82.25+148.05 =230.9

Benefit Of Diversification =230.9-1645.5=66.4

Why should we use Component VaR and calculate accordingly ?

Thanks in Advance
Biju

 

[Nicole Seaman]

Hi David

For the GARP FRM Part-2 2017 Qstn#60

Asset position Value (USD million) Return Standard Deviation (%) Beta(To Portfolio)
HIJ 200 30 1.30
KLM 300 25 0.55
Portfolio 500 20 1.0


----
For the above question

For the Diversification benefit can't I calculate without Marginal VaR ?

ie
Portfolio Var = 1.645*.0.2*500*=1645.5

VaR HIJ=1.645*.25*200=82.25
VaR KLM=1.645*.30*300=148.05
Total StandAlone VaR=82.25+148.05 =230.9

Benefit Of Diversification =230.9-1645.5=66.4

Why should we use Component VaR and calculate accordingly ?

Thanks in Advance
Biju

@Biju

Please note that I moved your question to this thread, where this practice question has been discussed in depth already. Note at the top that we use tags (i.e. garp17-p2-60) to tag the GARP practice questions. If you search by the tags, many times you will find that these questions have been discussed. This specific question has also been discussed in these two threads, which can also be found using the regular search function in the forum:

• https://forum.bionicturtle.com/threads/garp-2017-p2-60-garp17-p2-60.10491/
  
• https://forum.bionicturtle.com/threads/typos-in-2017-frm-part-2-practice-exam.10290/

Thank you,

Nicole

 

[[email protected]]

Hi David,
A quick maths/ covariance properties question on how covariance(x,p) is derived.
I get confused with how we square weights in the portfolio variance formula, but do not do this in the covariance (i,p) term i.e. weight(x)*covar(x,x) + weight(y)*covar(x,y).
Can you point me to the right derivation/ explanation please?
Thanks

 

[emilioalzamora1]

Hi @[email protected],

you can have a look at David's and my post here about the derivation of the covariance:

https://forum.bionicturtle.com/threads/p1-t2-705-correlation-hull.10172/#post-48565

proof of the covriance between two assets:

cov(i,j) = [cov(i,m)/variance(m)] * [cov(j,m)/variance(m)] * variance(m)

cov(i,j) = [std(i)*std(m)*corr(i,m)/variance(m)] * [std(j)*std(m)*corr(j,m)/variance(m)] * variance(m)

cov(i,j) = [std(i)*corr(i,m)/std(m)] * [std(j)*corr(j,m)/std(m)] * variance(m)

cov(i,j) = [std(i)*corr(i,m)*std(j)*corr(j,m)/variance(m)] * variance(m)

cov(i,j) = std(i)*corr(i,m)*std(j)*corr(j,m)

cov(i,j) = std(i)*std(j)*corr(i,j)

On top of this, remember this statement made by David:

while cov(i,j) = cov(j.i) but β(i,j) ≠ β(j,i) so it's important to specify the order for beta ("one variable with respect to another")

 

[[email protected]]

Thanks. I have a mental block about that one... found a simple example as well in the Jorion qs.
This is the property where the multiplier (weight) jumps out.

Answers:

2.1. C. 1.25
Beta (USD, Portfolio) = Covariance(USD, Portfolio)/Variance(Portfolio).

Covariance (USD, 0.5*USD + 0.5*EUR) =
= Covariance (USD, 0.5*USD) + Covariance (USD, 0.5*EUR)
= 0.5 * Variance (USD) + 0.5 * Covariance(USD,EUR) = 0.5 * 30%^2 + 0.5 * 20% * 30% * 0.6 = 0.063;
Variance (Portfolio) = 0.5^2 * 20%^2 + 0.5^2 * 30%^2 + 2 * 0.5 * 0.5 * 20% * 30% * 0.6
= 0.0505

Therefore, Beta (USD, Portfolio) = Covariance(USD, Portfolio)/Variance(Portfolio) = 0.063/0.0505 = 1.247525

 

[Flashback]

The question for @David Harper CFA FRM

In calculating SaR, I found that (z)(σ)(Surplus amount) is noted as a relative SaR while - ΔSurplus - (z)(σ) is noted as an absolute SaR. Shouldn't be opposite?
ΔSurplus may be noted in % rather than in absolute value.

 

[Karim_B]

The question for @David Harper CFA FRM

In calculating SaR, I found that (z)(σ)(Surplus amount) is noted as a relative SaR while - ΔSurplus - (z)(σ) is noted as an absolute SaR. Shouldn't be opposite?
ΔSurplus may be noted in % rather than in absolute value.

Hi @Flashback
I think there are a couple of distinctions between the Surplus at Risk questions you found.

1) Absolute vs Relative SaR
Here the difference is whether or not you use the expected change in surplus, similarly as to whether you use the mean return or not in absolute vs relative VaR.

For example see 710.3 here https://forum.bionicturtle.com/thre...ue-at-risk-var-and-surplus-at-risk-sar.10741/

Absolute SaR takes into consideration the expected change in Surplus, whereas Relative SaR doesn't take the expected change in surplus into account and therefore is "relative" to whatever the change in surplus ends up being in the future.

Screenshot where you can see the difference between Absolute & Relative SaR = Expected change in surplus:


Here's more detail on Abs vs Rel VaR:
https://forum.bionicturtle.com/thre...ersus-relative-var-versus-ul.6020/#post-41655

2) Percent vs Currency Amount SaR
I can't remember seeing Percent SaR questions, but conceptually I think it's like having % VaR or Currency Amount VaR. For the Percent versions of Absolute or Relative SaR you wouldn't multiply by the ExpectedΔSurplus & Surplus amounts in whatever currency they're given.

So Absolute SaR in % & USD:
-ExpectedΔSurplus % + (z)(σ) = Absolute SaR in %
-ExpectedΔSurplus in USD + (z)(σ)(Surplus in USD) = Absolute SaR in USD

Relative SaR in % & USD:
(z)(σ) = Relative SaR in %
(z)(σ)(Surplus in USD) = Relative SaR in USD

I think @David Harper CFA FRM is taking some time to work on the content plan until next Monday based on his status update.

Best
Karim

 

[Flashback]

Great! I had this example on my mind when I asked to clarify.
Thus, an Absolute SaR is with Delta Surplus (Change between an initial A/L position and the compounded A/L) while the Relative SaR is an ordinary VaR with Surplus as an asset value.
What if there is a deficit instead of Surplus? How to interpret such SaR?

 

[Karim_B]

Great! I had this example on my mind when I asked to clarify.
Thus, an Absolute SaR is with Delta Surplus (Change between an initial A/L position and the compounded A/L) while the Relative SaR is an ordinary VaR with Surplus as an asset value.
What if there is a deficit instead of Surplus? How to interpret such SaR?

Hi @Flashback
Yeah so Absolute SaR includes the expected Delta Surplus, and the Relative SaR doesn't include it (both would use the Surplus value).

My understanding is that SaR measures funding risk (i.e. does a pension plan sponsor need to fund an excess of liabilities compared to its assets). From study note R72-P2-T8 Jorion:
"This funding risk represents the true long-term risk to the owner of the fund. If the surplus turns negative, it will have to provide additional contributions to the fund. This is called surplus at risk (SAR)."

One other thing to keep in mind in terms of the info given, is that on GARP practice exam questions they actually make you calculate the standard deviation of the surplus, and the SaR is then calculated from that.

You can see that in the "GARP's SaR" portion of 710.3 which I linked above too https://forum.bionicturtle.com/...ue-at-risk-var-and-surplus-at-risk-sar.10741/

When you're ready for the GARP 2018 part II practice exam check out question 24 on SaR.

Best
Karim

 

[Flashback]

Thanks Karim at first for Your great explanation and the tip about GARP P II Q24. I will practice these for sure.
About negative surplus, contributions may be a solution. IMO, another way is taking immediate action to reduce A/L gap and thus eliminate deficit. A combination of both, additional cash contributions and reducing a duration mismatch would be a preferable course of action.