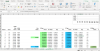
The key rate shift technique overcomes the key limitation of duration and DV01 which is that they must assume a parallel shift in the yield curve because they are single-factor risk measures. The key rate shift technique, on the other hand, is multi-factor: the term structure is carved into a limited number of "key rate regions;" in this illustration, four key rates are selected, 2-year, 5-year, 10-year, and 30-year. You can find Tuckman's Fixed Income Securities book here: https://amzn.to/2SOMGzv

Last edited: