P1.T4.318. Key rates exposures (Tuckman 3rd edition)

[Miriam]

AIMs: Describe and assess the major weakness attributable to single-factor approaches when hedging portfolios or implementing asset liability techniques. Describe key-rate shift analysis. Define, calculate, and interpret key rate ‘01 and key rate duration.

Questions

318.1. The exhibit below shows the results of a key-rate shift calculation for 30-year bond with face value of $1,000 that pays a semi-annual coupon with coupon rate of 2.0%. If the current par yield curve is flat at 4.0%, the initial price of the bond is approximately $652.39. The exhibit omits the four bond values that result from re-pricing the bond under a key rate shift but does show the resulting key-rate '01 (KR01s) and key-rate durations:

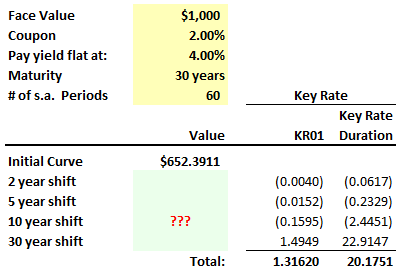
How does the 10-year shift value (red "???") compare to the initial value of $652.39?

a. Less by $0.1595
b. Less by $2.4451
c. Greater $0.1595
d. Greater by $2.4451

318.2. The following exhibit displays key rate durations (final column) for a par $1,000 zero-coupon bond with 30 years to maturity. The bond has an initial value of $304.78 because the current par yield curve is flat at 4.0%:

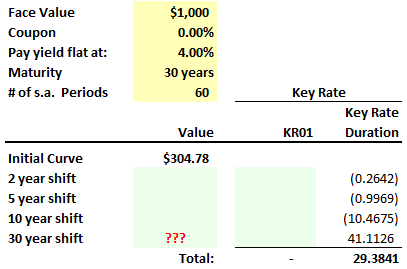
Which is nearest the the value of the bond under a 30-year shift (red "???")?

a. $303.53
b. $304.29
c. $306.03
d. $307.42

318.3. Sally manages a bond portfolio that is benchmarked against an index with the following key rate profile:

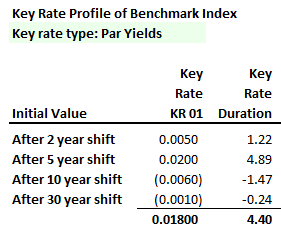
Sally's single-factor portfolio duration exactly matches the index's duration. However, compared to the benchmark which is concentrated in shorter maturities, Sally's portfolio has a higher concentration in bonds with higher maturities; i.e., 10 and 30 year maturities. Which of the following statements is true?

a. If rates steepen (i.e., a non-parallel shift), Sally's portfolio is likely to underperform the benchmark
b. If rates steepen (i.e., a non-parallel shift), Sally's portfolio is likely to overperform the benchmark
c. Key rate shifts is still a single-factor technique because convexity is not included, such that Sally's portfolio is likely to match the benchmark's portfolio even under a non-parallel shift
d. Sally should expect that the benchmark index's yield-based DV01 is equal to exactly 0.01800 because that is the sum of the KR01

Answers:

• Here in forum

 

[[email protected]]

Good afternoon - please could you be so kind to prompt me - it is FRM Part 1 program or FRM Part 2? Cause I don`t quite remember anything like this from FRM Part 1 program topics? Thank you beforehand for your answer...
Regards

 

[noalv4]

Hi,

The link to the answers does not work...

Thanks,
Noa

 

[Suzanne Evans]

Noa,

Can you please try now. I didn't test the link before I changed it, but it is working now.

Thanks,
Suzanne

 

[Suzanne Evans]

monte,

This is a question for Part 1, Topic 4: Valuation & Risk Models.

It is from Tuckman, Chapter 5: Multi-Factor Risk Metrics & Hedges.

Thanks,
Suzanne

 

[[email protected]]

Well then the answers are:

318.1 c
318.2 a
318.3 I am dubious either d or b (sorry not quite understand the crux of this question)

 

[David Harper CFA FRM]

correct re 1 and 2!

re .3, it's based on the intro to Tuckman Chapter 5 and meant to highlight the very purpose of key rates. If the rate shift is parallel, then a portfolio that matches the duration of the index implies approximately similar performance (fund vs index).

But a non-parallel shift will exploit differences in key rate exposures. Consider, for example, a non-parallel shift where both long/short rates are increasing (not the scenario in the question) under a curve "flattening": this is more painful for the index above, as is has positive duration exposure to the short key rates and actually negative duration exposure to the long rates. Under increasing-rates-and-flattening (flattening = short rate increase > long rate increase), the index above is adversely impacted by the increasing short rates rate where its exposure is concentrated.

 

[[email protected]]

Then the answer is probably c?

 

[David Harper CFA FRM]

Key rate shift is a multi-factor approach, each of the key rates is a factor which can independently impact price. Unlike duration/DV01 which are single factor where the single factor is yield to maturity ("yield"), which incorporates the entire term structure all at once (at by shocking, implicitly assumes a parallel shift).

Adding convexity does not, per se, change the "single factor" aspect of duration/DV01: it's just a second-order term operating on the same single factor.

 

[[email protected]]

Thank you very much David for virtually chewing it over! Now I understand that key rate shifts are of course multifactor due to adverse changes and to the effect of one key rate affecting the others that are for longer maturities. But what I still don`t understand is how it can be the KR01 are permanently negative, because when we talk about DV01 - it is positive in a sense that everubody understands that after yield goes up - price goes down and vice versa. Or it is just an indication that after a negative shift of key rate (direction) the price inhanced...

Thank you one more time for your help, you are very helpful!

I would like very much to pass FRM but it`s not that easy... especially when you are sifting through these mazy aspects of fixed income securities

 

[orit]

Hi Suzanne,
where can I see the link to the excel sheet?
thanks

 

[macy]

HI David,

Could you please explain more about question 1. I dont get whey the 10year shift value in the question is greater than -KR01 while the 10year shift value in the example on P.131 of your notes does not ?

Thanks

 

[David Harper CFA FRM]

Hi macy, am i missing something, they are directionally similar as far as i can tell:

• the notes mimic Tuckman's Chapter 5 example: -(26.25763 - 26.22311 initial value) = -0.0345
• this question implies -(652.5506 - 652.3911 initial value) = -0.1595

As discussed in the answer to question 318.1 (here) and Tuckman, I think it is counter-intuitive that an increase in the 10-year par rate (in both cases) implies an increase in the bond price. But both examples use Tuckman (5.2) for KR01 = -1/10,000*[(new value) - (initial value)]/0.01% = -[new value - initial value]. Thanks,

 

[[email protected]]

318.2 C isnt it?

please confirm Many Thanks

 

[David Harper CFA FRM]

riskgeek if the key rate duration is positive, should the shocked price go up or down?

 

[[email protected]]

Got it I was getting c. $306.03 since i was not using "-"

After substituting i got a. $303.53

Thanks

 

[David Harper CFA FRM]

Awesome, chatting about it helps me to keep sharp, it's rarely natural

 

[cash king]

I think question 318.2 is confusing
1. the zero coupon bond has only one future cashflow, indicating it should be exposed to 30-year rate shiftonly. But why in your question your have non-zero key rates duration for year 2,5,10
2. why the key rate duration for year 2,5,10 is negative? I thought only when you had negative future cashflows (such as in a IRS) you could possibly have negative key rate duration. Any explanation?

 

[David Harper CFA FRM]

Hi @cash king

They are both counter-intuitive indeed, but they are both explained in Tuckman's Chapter 5, as he introduces key rates, in fact. I considered using spot rates (which would not produce the seemingly awkward outcomes) but Tuckman's entire introduction utilizes par yields as the key (interest) rates rather than spot rates.

Consequently, for example, similar to Tuckman's own Table 5-2, in my 318.2 above a shock to the 10-year par yield key rate, of plus one basis point, from 4.00% to 4.01%, while it has no impact on the 30-year par yield, does decrease (ever so slightly) the 30-year spot rate, which in turn, slightly (and counter-intuitively) increases the price of the 30-year zero coupon bond. Hence, the answer to both of your questions: the 10-year key rate duration is negative because the re-priced bond increases in price due to the +1 bps shock to the 10-year par yield (and neighboring par yields). This nuance, in my opinion (with little doubt), is (way) above-beyond the level of the testable P2 exam; but Tuckman (from 2nd to 3rd Edition) introduced this outcome and spends some paragraphs explaining it, and so I did not want to run from the complexity (if I did, then eventually somebody would ask why my examples are different than his).

I am going to upload the underlying spreadsheets (behind these questions) to the paid-member Q&A, in case someone wants to further explore (or verify, for that matter) there. It's much easier (I think) to see in action, but again, the counter-intuitive outcome of "par yield as key rate" (versus spot rates as key rate) as a concept is also extra-curricular to the FRM. Thanks,

 

[Arka Bose]

Are the spreadsheets available?