Hi @David Harper CFA FRM
I was watching the chapter two video this morning while getting ready for work. I came across this and had a question for you.
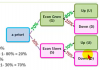
What is the probability of just an "up" situation?
Is it (0.7) x (0.8) + (0.3) x (0.3) ? = 0.65?
I was watching the chapter two video this morning while getting ready for work. I came across this and had a question for you.

What is the probability of just an "up" situation?
Is it (0.7) x (0.8) + (0.3) x (0.3) ? = 0.65?
