Hi David,
Pls elaborate more on the answer provided to below question.To my understanding, we should have selected the answer c), since it exceeds the value (5) for 99% confidence. Text highlighted in red is not clear.
207.1. A bank's VaR, calibrated with 99% confidence and a one-day horizon, is $10.0 million. The bank conducted a backtest over the previous 500 trading days in order to ascertain, with 95% confidence, whether the 99% VaR model is good or bad ("faulty"). It is assumed losses are i.i.d. Which backtest observation would MOST LIKELY implicate the VaR model as bad (faulty) with 95% confidence?
a) Never (on no single day) did the daily loss exceed the $10.0 million VaR
b) Only once was the daily loss exactly $10.0 million
c) On exactly eight days the daily loss exceeded the $10.0 million VaR
d) The largest daily loss was over $50.0 million, which is more than 5x the daily VaR
207.1. A. Never (on no single day) did the daily loss exceed the $10.0 million VaR
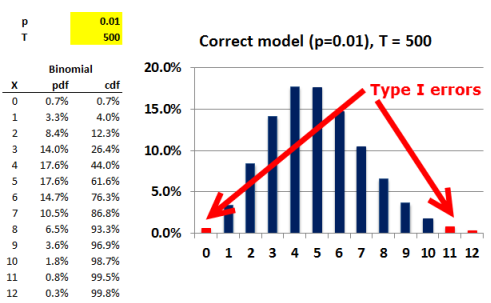
Under i.i.d., a good 99% VaR model would expect 1% * 500 = 5 daily exceptions (exceedences).
The probability of zero exceptions = 99%^500 = 0.657%, which is less than 2.5% (i.e., outside the 5% two-tailed acceptance region).
So, zero exceptions would lead to a "reject null" conclusion.
In regard to (B), (C), and (D), none of these observations implicate the model as faulty: 8 exceptions occurs with 6.5% probability (93.3% cumulatively, which is inside the acceptance region); a single large outlier does not contradict VaR, which says nothing about magnitude of losses in excess of VaR.
Pls elaborate more on the answer provided to below question.To my understanding, we should have selected the answer c), since it exceeds the value (5) for 99% confidence. Text highlighted in red is not clear.
207.1. A bank's VaR, calibrated with 99% confidence and a one-day horizon, is $10.0 million. The bank conducted a backtest over the previous 500 trading days in order to ascertain, with 95% confidence, whether the 99% VaR model is good or bad ("faulty"). It is assumed losses are i.i.d. Which backtest observation would MOST LIKELY implicate the VaR model as bad (faulty) with 95% confidence?
a) Never (on no single day) did the daily loss exceed the $10.0 million VaR
b) Only once was the daily loss exactly $10.0 million
c) On exactly eight days the daily loss exceeded the $10.0 million VaR
d) The largest daily loss was over $50.0 million, which is more than 5x the daily VaR
207.1. A. Never (on no single day) did the daily loss exceed the $10.0 million VaR
Under i.i.d., a good 99% VaR model would expect 1% * 500 = 5 daily exceptions (exceedences).
The probability of zero exceptions = 99%^500 = 0.657%, which is less than 2.5% (i.e., outside the 5% two-tailed acceptance region).
So, zero exceptions would lead to a "reject null" conclusion.
In regard to (B), (C), and (D), none of these observations implicate the model as faulty: 8 exceptions occurs with 6.5% probability (93.3% cumulatively, which is inside the acceptance region); a single large outlier does not contradict VaR, which says nothing about magnitude of losses in excess of VaR.