chatty06
New Member
Hi All, I'm completing question 2.4 in the GARP book quantitative analysis.
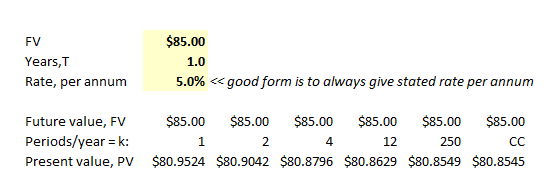
The question is just asking to get the present value of a bond that is expected to be $85 after 1 year at a continuous interest rate of 5%.
In the answer it says it should be $80.85 but when I run my PV calcs I get 80.95. Any thoughts? I tried both on my calculator and in excel and I get the same price. This seems like a simple calculation.
Thanks for the help.
The question is just asking to get the present value of a bond that is expected to be $85 after 1 year at a continuous interest rate of 5%.
In the answer it says it should be $80.85 but when I run my PV calcs I get 80.95. Any thoughts? I tried both on my calculator and in excel and I get the same price. This seems like a simple calculation.
Thanks for the help.

 Let me just show you the quicker way. To compound PV at stated rate of (r) continuously over T years, we use FV=PV*exp(rT). I think of discounting as compounding but backwards, such that to discount the FV to the present value at a stated rate of (r) over T years with continuous discounting, we us PV = FV*exp(-rt) which is the same as PV = FV/exp(rt) because x^(-a) = x*1/a = x/a. So with respect to continuous discounting, the most efficient formula is PV = FV*exp(-rt) and so you can get the solution on the calculator with the following (where "##" indicates my comment):
Let me just show you the quicker way. To compound PV at stated rate of (r) continuously over T years, we use FV=PV*exp(rT). I think of discounting as compounding but backwards, such that to discount the FV to the present value at a stated rate of (r) over T years with continuous discounting, we us PV = FV*exp(-rt) which is the same as PV = FV/exp(rt) because x^(-a) = x*1/a = x/a. So with respect to continuous discounting, the most efficient formula is PV = FV*exp(-rt) and so you can get the solution on the calculator with the following (where "##" indicates my comment):