afterworkguinness
Active Member
Hi David,
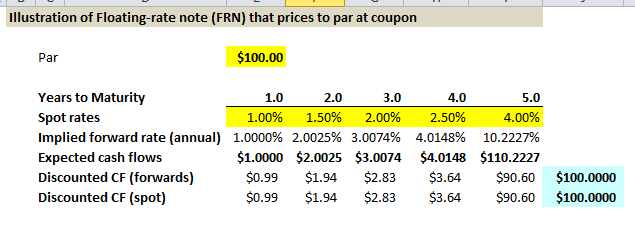
I don't fully understand how to value a plain vanilla swap as a fix rate and floating rate bond. In your Youtube video, for the floating rate bond you price it as PV (1st coupon + notional). Why don't you take into account the other floating rate coupons that take place during the life of the swap ?
Thanks in advance.
ps: Your Youtube videos are awesome.
I don't fully understand how to value a plain vanilla swap as a fix rate and floating rate bond. In your Youtube video, for the floating rate bond you price it as PV (1st coupon + notional). Why don't you take into account the other floating rate coupons that take place during the life of the swap ?
Thanks in advance.
ps: Your Youtube videos are awesome.