You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
GARP.FRM.PQ.P1 Unexpected loss formula decipher
- Thread starter aimjo
- Start date
Hi @aimjo If you are referring to formula 5.8 in Schroeck, then I agree with you: not only is it too complicated, but we had to explain to GARP why it's actually wrong  (see https://forum.bionicturtle.com/threads/november-2017-part-1-exam-feedback.12896/page-5#post-56335, it actually appeared as a mistake on the Nov 2017 exam)
(see https://forum.bionicturtle.com/threads/november-2017-part-1-exam-feedback.12896/page-5#post-56335, it actually appeared as a mistake on the Nov 2017 exam)
The correct portfolio UL is given in our notes by the following (and was presented in the previously assigned Ong (https://forum.bionicturtle.com/resources/internal-credit-risk-models-by-michael-ong.131/):
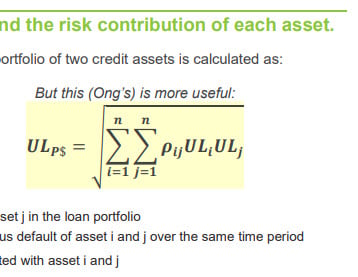
This is correct and hopefully familiar: portfolio UL is a essentially similar to portfolio standard deviation (e.g., https://en.wikipedia.org/wiki/Modern_portfolio_theory). It's actually the same formula.
 (see https://forum.bionicturtle.com/threads/november-2017-part-1-exam-feedback.12896/page-5#post-56335, it actually appeared as a mistake on the Nov 2017 exam)
(see https://forum.bionicturtle.com/threads/november-2017-part-1-exam-feedback.12896/page-5#post-56335, it actually appeared as a mistake on the Nov 2017 exam)The correct portfolio UL is given in our notes by the following (and was presented in the previously assigned Ong (https://forum.bionicturtle.com/resources/internal-credit-risk-models-by-michael-ong.131/):

This is correct and hopefully familiar: portfolio UL is a essentially similar to portfolio standard deviation (e.g., https://en.wikipedia.org/wiki/Modern_portfolio_theory). It's actually the same formula.
- In the standard mean-variance 2-asset portfolio variance, we have σ$(1)^2 + σ$(2)^2 + 2*σ$(1)*σ$(2)*ρ(1,2),
- but 2-asset portfolio UL^2 = UL$(1)^2 + UL$(2)^2 + 2*UL$(1)*UL$(2)*ρ(1,2), which is the same. This is just a reduction of the above formula under the square root: portfolio UL^2 = UL$(1)*UL$(1)*ρ(1,1) + UL$(2)*UL$(2)*ρ(2,2) + 2*UL$(1)*UL$(2)*ρ(1,2). I hope that's helpful,
bollengc
Member
hi @David Harper CFA FRM ,Hi @aimjo If you are referring to formula 5.8 in Schroeck, then I agree with you: not only is it too complicated, but we had to explain to GARP why it's actually wrong(see https://forum.bionicturtle.com/threads/november-2017-part-1-exam-feedback.12896/page-5#post-56335, it actually appeared as a mistake on the Nov 2017 exam)
The correct portfolio UL is given in our notes by the following (and was presented in the previously assigned Ong (https://forum.bionicturtle.com/resources/internal-credit-risk-models-by-michael-ong.131/):

This is correct and hopefully familiar: portfolio UL is a essentially similar to portfolio standard deviation (e.g., https://en.wikipedia.org/wiki/Modern_portfolio_theory). It's actually the same formula.
- In the standard mean-variance 2-asset portfolio variance, we have σ$(1)^2 + σ$(2)^2 + 2*σ$(1)*σ$(2)*ρ(1,2),
- but 2-asset portfolio UL^2 = UL$(1)^2 + UL$(2)^2 + 2*UL$(1)*UL$(2)*ρ(1,2), which is the same. This is just a reduction of the above formula under the square root: portfolio UL^2 = UL$(1)*UL$(1)*ρ(1,1) + UL$(2)*UL$(2)*ρ(2,2) + 2*UL$(1)*UL$(2)*ρ(1,2). I hope that's helpful,
I am currently reviewing T6-R9 and from your comment above, I understand formula 5.9 is wrong?

If we consider a portfolio with 50% of asset i and 50% of asset j, the 2 formulas in the screenshot are not matching.
or at least the notations UL_i and UL_j should refer to different definitions in both formulas.
I thought I saw a similar formula in part 1, but might have been when browsing some practice questions from older versions.
any explanation on the above would be great

(and if the formula is not correct, maybe better to remove that part from the notes to prevent losing more people
 )
)edit:
I just found an example showing the 2 formulas:

how would the UL_i and UL_j be expressed in % mode?
thanks,
Camille
Last edited:
Hi Camille (@bollengc ) I hear you re: removing the formula but it's in GARP's notes so I've tagged the note for some improvement explanation (the extra work GARP creates for us, yay!). So both formulas are okay, if we understand how to use the first one in percentage terms, to your point. Using my question 506.3 as an example ....
where my solution uses the easier dollar-based solution ...506.3. A bank has extended two loans to customers in the same industry. Both loans are have an exposure amount (EA) of $50.0 million, default probability (PD) of 2.0%, loss rate (LR) of 50.0%, and standard deviation of loss rate of 60.0% such that each loan has an expected loss of $500,000 and an unexpected loss of $5.5 million. In this way, the bank's credit portfolio consists of these two credit assets; and the default correlation between the two loans is 28.0%. Which is nearest to the risk contribution of each asset to the portfolio's unexpected loss?
a. $3.33 million
b. $4.40 million
c. $5.37 million
d. $5.50 million
If we want to use the percentage (%) based, the key is to realize that UL(1) and UL(2) are given by $5.5 mm / $50.0 mm = 11.0%. Now we can use SQRT(50%^2 * 11.0%^2 + 50%^2 * 11.0%^2 + 2*50%*50%*11.0%*11.0%*28.0%) = 8.80% which is that same $100.0 * 8.80% = $8.80 UL. I hope that explains it!in this case, the portfolio unexpected loss reduces to sqrt[2*UL(1)^2 + 2*ρ(1,2)*UL(1)^2] = sqrt[2*5.5^2 + 2*0.28*5.5^2] = $8.80 million.
bollengc
Member
Thanks David (@David Harper CFA FRM )Hi Camille (@bollengc ) I hear you re: removing the formula but it's in GARP's notes so I've tagged the note for some improvement explanation (the extra work GARP creates for us, yay!). So both formulas are okay, if we understand how to use the first one in percentage terms, to your point. Using my question 506.3 as an example ....
where my solution uses the easier dollar-based solution ...
If we want to use the percentage (%) based, the key is to realize that UL(1) and UL(2) are given by $5.5 mm / $50.0 mm = 11.0%. Now we can use SQRT(50%^2 * 11.0%^2 + 50%^2 * 11.0%^2 + 2*50%*50%*11.0%*11.0%*28.0%) = 8.80% which is that same $100.0 * 8.80% = $8.80 UL. I hope that explains it!
crystal clear with this numerical example

Similar threads
- Replies
- 2
- Views
- 462
- Replies
- 1
- Views
- 284
- Replies
- 0
- Views
- 244
- Replies
- 0
- Views
- 129
