Hi @gargi.adhikari It's a good question, I am using Amenc's formula below: IR = t-stat/sqrt(T), such that t-stat = IR*sqrt(T); in the example above, the active IR = 4.49% (displayed as 0.045) such that t-stat = 4.49*5.0 years, where 5.0 years is a simple input. So the 4.49% is just the information ratio although I made a mistake to show it formatted a percentage (%), which probably throws you off. because I do think it's unitless (%/%); i.e., I think its correct to say that IR is a (unitless) percent not a percentage, so it's 0.045 not really 4.5%.
I could instead have asked for confidence as an input; e.g., like Amenc, let 95% determine a 1.96 2-tailed deviate. Then the number of years implied would be (1.96/0.045)^2 = 1,902 years of this sort of performance in order to be deemed statistically significant at 95%, wow! This is always confusing to folks, the key idea is that the information ratio scales with the square root of time (sound familiar?) because IR = α/σ(α) scales with Δt per [α*Δt]/[σ(α)*sqrt(Δt)]; i.e., alpha is linear but standard deviation scales with sqrt(T), such that sustained/scaled IR = [α*Δt]/[σ(α)*sqrt(Δt)] = [α/σ(α)]*[Δt/sqrt(Δt)] = [α/σ(α)]*sqrt(Δt). But IR is itself a t-stat (coefficient divided by its own standard error!), so this is t-stat = IR*sqrt(T); i.e., scaling the annual IR over the number of sustained periods renders it its own t-stat. Please note this notation doesn't distinguish between which alpha; the XLS happens to use the active IR. I hope that explains!
This is always confusing to folks, the key idea is that the information ratio scales with the square root of time (sound familiar?) because IR = α/σ(α) scales with Δt per [α*Δt]/[σ(α)*sqrt(Δt)]; i.e., alpha is linear but standard deviation scales with sqrt(T), such that sustained/scaled IR = [α*Δt]/[σ(α)*sqrt(Δt)] = [α/σ(α)]*[Δt/sqrt(Δt)] = [α/σ(α)]*sqrt(Δt). But IR is itself a t-stat (coefficient divided by its own standard error!), so this is t-stat = IR*sqrt(T); i.e., scaling the annual IR over the number of sustained periods renders it its own t-stat. Please note this notation doesn't distinguish between which alpha; the XLS happens to use the active IR. I hope that explains!
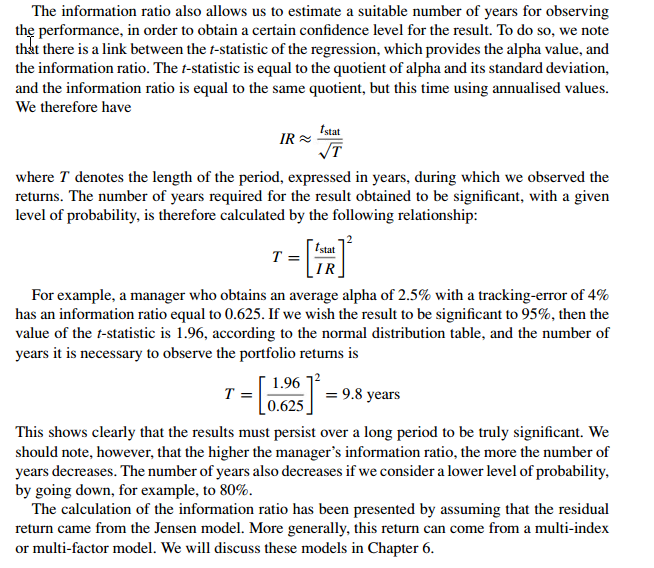
I could instead have asked for confidence as an input; e.g., like Amenc, let 95% determine a 1.96 2-tailed deviate. Then the number of years implied would be (1.96/0.045)^2 = 1,902 years of this sort of performance in order to be deemed statistically significant at 95%, wow!
 This is always confusing to folks, the key idea is that the information ratio scales with the square root of time (sound familiar?) because IR = α/σ(α) scales with Δt per [α*Δt]/[σ(α)*sqrt(Δt)]; i.e., alpha is linear but standard deviation scales with sqrt(T), such that sustained/scaled IR = [α*Δt]/[σ(α)*sqrt(Δt)] = [α/σ(α)]*[Δt/sqrt(Δt)] = [α/σ(α)]*sqrt(Δt). But IR is itself a t-stat (coefficient divided by its own standard error!), so this is t-stat = IR*sqrt(T); i.e., scaling the annual IR over the number of sustained periods renders it its own t-stat. Please note this notation doesn't distinguish between which alpha; the XLS happens to use the active IR. I hope that explains!
This is always confusing to folks, the key idea is that the information ratio scales with the square root of time (sound familiar?) because IR = α/σ(α) scales with Δt per [α*Δt]/[σ(α)*sqrt(Δt)]; i.e., alpha is linear but standard deviation scales with sqrt(T), such that sustained/scaled IR = [α*Δt]/[σ(α)*sqrt(Δt)] = [α/σ(α)]*[Δt/sqrt(Δt)] = [α/σ(α)]*sqrt(Δt). But IR is itself a t-stat (coefficient divided by its own standard error!), so this is t-stat = IR*sqrt(T); i.e., scaling the annual IR over the number of sustained periods renders it its own t-stat. Please note this notation doesn't distinguish between which alpha; the XLS happens to use the active IR. I hope that explains!

 Thanks a ZILLION !
Thanks a ZILLION !