gargi.adhikari
Active Member
In reference to R8.P1.T1.Amenc_Ch4_RISK_MGMT_Topic:INFORMATION_RATIO_RESIDUAL vs_ACTIVE:
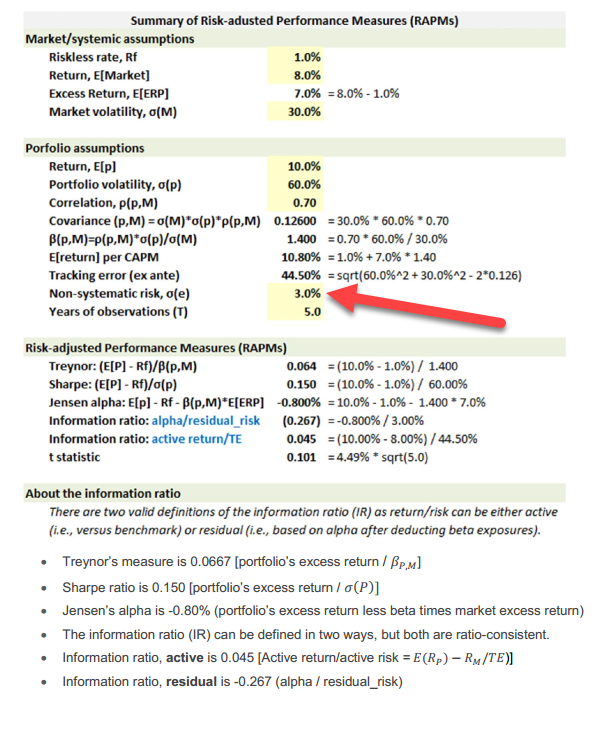
The Residual Risk is the idiosyncratic Risk = any risk which is not the Systemic Risk
and that Total Risk = Systemic Risk + Residual Risk.
Having said that though, I am trying to get the standardized formula to use for calculating Info-Ratio when we use:-
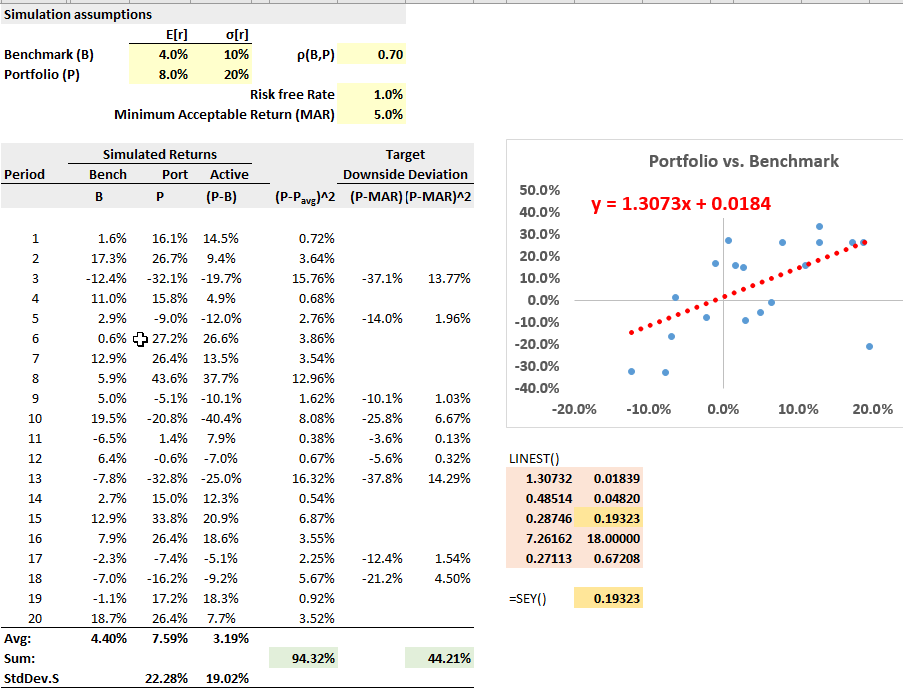
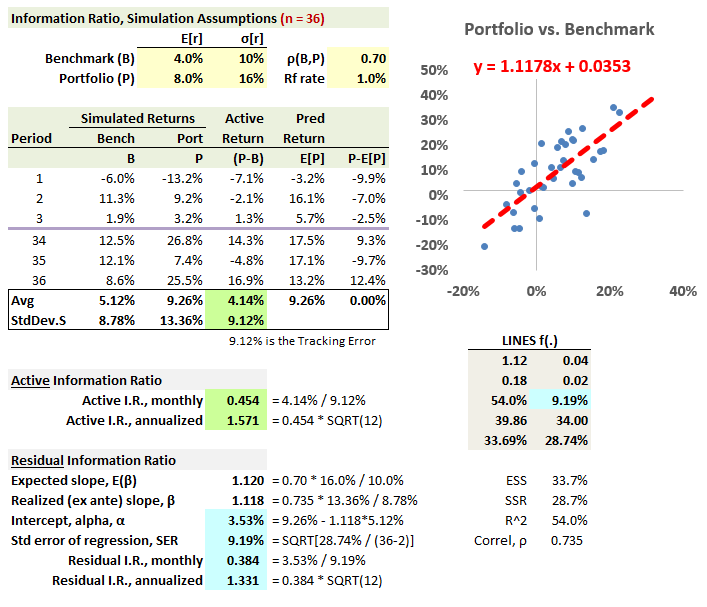
The study materials indicate just one formula for the Info-Ratio IR = E(Rp) - E(Rb) / Std-Dev( Rp - Rb)
Want to understand is whether the above stated formula is a) Residual Return/ Residual Risk or b) Active Return/ Active Risk... :-( ...?
Thanks much for all the help and insight on this topic ..
The Residual Risk is the idiosyncratic Risk = any risk which is not the Systemic Risk
and that Total Risk = Systemic Risk + Residual Risk.
Having said that though, I am trying to get the standardized formula to use for calculating Info-Ratio when we use:-
a) Residual Return/ Residual Risk vs.
b) Active Return/ Active Risk
b) Active Return/ Active Risk
The study materials indicate just one formula for the Info-Ratio IR = E(Rp) - E(Rb) / Std-Dev( Rp - Rb)
Want to understand is whether the above stated formula is a) Residual Return/ Residual Risk or b) Active Return/ Active Risk... :-( ...?
Thanks much for all the help and insight on this topic ..
Last edited: