gargi.adhikari
Active Member
In reference to R27.P1.T4.Hull_Ch13_15_19:Topic: BINOMIAL_TREE_OPTION_PRICING :-
Hi,
I just wanted to clarify something on this topic in the example illustrated below:-
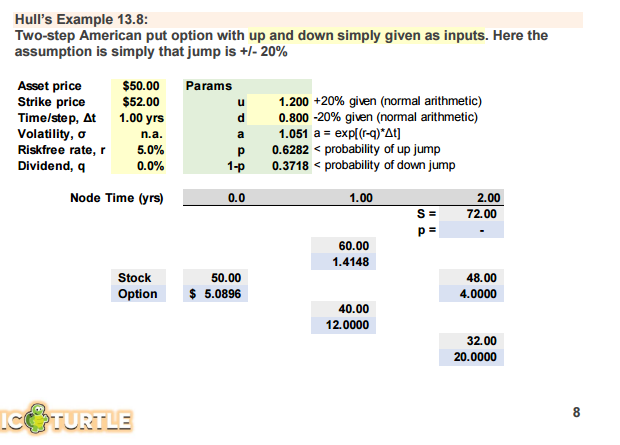
Here , the Volatility is a given input = 30 %
Time = 1 yr
So, then the Magnitude of Up Jump , u should be solved to be = e ^ [ Volatility * SQRT( Time) ] = e^ [ .3 * 1] = 1.345
But, instead the Magnitude of Up Jump , u: seems to be a Given input = 1.2 instead of 1.345..
Note : I do see in the new/latest notes for this example that Volatility is not given and so the Magnitude of Up Jump , u is a given input = 1.2
Much gratitude for a clarification on this.

Hi,
I just wanted to clarify something on this topic in the example illustrated below:-
Here , the Volatility is a given input = 30 %
Time = 1 yr
So, then the Magnitude of Up Jump , u should be solved to be = e ^ [ Volatility * SQRT( Time) ] = e^ [ .3 * 1] = 1.345
But, instead the Magnitude of Up Jump , u: seems to be a Given input = 1.2 instead of 1.345..
Note : I do see in the new/latest notes for this example that Volatility is not given and so the Magnitude of Up Jump , u is a given input = 1.2
Much gratitude for a clarification on this.