gargi.adhikari
Active Member
In reference to R26.P1.T4.DOWD_Topic: EXPECTED_SHORTFALL :-
For finding the ES, we find the Total area under the Tail and then divide by (1-alpha) to get the average amount of Loss under the Tail ...Shouldn't we dividing the Total area under the Tail by Alpha instead of ( 1-Alpha). Am probably missing a point here..Grateful for any insights into this...
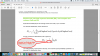
For finding the ES, we find the Total area under the Tail and then divide by (1-alpha) to get the average amount of Loss under the Tail ...Shouldn't we dividing the Total area under the Tail by Alpha instead of ( 1-Alpha). Am probably missing a point here..Grateful for any insights into this...


