Suzanne Evans
Well-Known Member
AIMs: Calculate the short-term rate change and standard deviation of the change of the rate using a model with normally distributed rates and no drift. Describe methods for handling negative short-term rates for term structure models
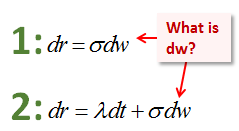
Questions:
303.1. Analyst Barry runs an short-term interest rate simulation using Tuckman's simple Model 1, which assumes no drift (zero drift) and is given by:
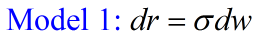
(Source: Bruce Tuckman, Fixed Income Securities, 3rd Edition (Hoboken, NJ: John Wiley & Sons, 2011))
The time step in his model is one month; i.e., dt = 1/12. His Model 1 also makes two assumptions. First, the initial or current (t0) short-term rate is equal to 4.00%. Second, the annual basis-point volatility is 200 basis points. In the first step of his first trial, the random uniform variable is 0.8925 such that, via inverse transformation, the associated random standard normal value is 1.240; i.e., =NORM.S.INV(89.25%) = 1.240. If r(0) is 4.00%, to what level does the rate evolve in the first month, r(1/12)?
a. 3.854%
b. 4.716%
c. 5.393%
d. 6.480%
303.2. Analyst Barry wants to improve his short-term interest rate simulation by employing Tuckman's Model 2, which adds a drift term to Model 1 and is given by:
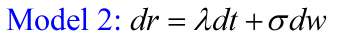
(Source: Bruce Tuckman, Fixed Income Securities, 3rd Edition (Hoboken, NJ: John Wiley & Sons, 2011))
The time step in his model is one month; i.e., dt = 1/12. His Model 2 also makes three assumptions. First, the initial or current (t0) short-term rate is equal to 4.00%. Second, the annual basis-point volatility is 250 basis points. Third, the annual drift is +100 basis points. In the first step of his first trial, the random uniform variable is 0.0793 such that, via inverse transformation, the associated random standard normal value is -1.410; i.e., =NORM.S.INV(7.93%) ~= -1.410. If r(0) is 4.00%, to what level does the rate evolve in the first month, r(1/12)?
a. 2.77%
b. 2.98%
c. 3.07%
d. 4.15%
303.3. Analyst Susan is evaluating various short-term interest rate models. Her primary criteria is to AVOID a model that will produce a negative short-term rate. Each of the following approaches can help Susan avoid a model that generates negative rate outcomes EXCEPT for:
a. She can increase the convexity effect input assumption
b. She can assume a non-normal distribution; e.g., lognormally distributed rates
c. She can employ shadow rates
d. She can assume a Cox-Ingersoll-Ross (CIR) model
Answers:
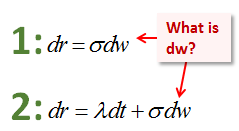
Questions:
303.1. Analyst Barry runs an short-term interest rate simulation using Tuckman's simple Model 1, which assumes no drift (zero drift) and is given by:
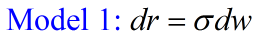
(Source: Bruce Tuckman, Fixed Income Securities, 3rd Edition (Hoboken, NJ: John Wiley & Sons, 2011))
The time step in his model is one month; i.e., dt = 1/12. His Model 1 also makes two assumptions. First, the initial or current (t0) short-term rate is equal to 4.00%. Second, the annual basis-point volatility is 200 basis points. In the first step of his first trial, the random uniform variable is 0.8925 such that, via inverse transformation, the associated random standard normal value is 1.240; i.e., =NORM.S.INV(89.25%) = 1.240. If r(0) is 4.00%, to what level does the rate evolve in the first month, r(1/12)?
a. 3.854%
b. 4.716%
c. 5.393%
d. 6.480%
303.2. Analyst Barry wants to improve his short-term interest rate simulation by employing Tuckman's Model 2, which adds a drift term to Model 1 and is given by:
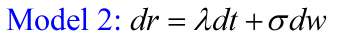
(Source: Bruce Tuckman, Fixed Income Securities, 3rd Edition (Hoboken, NJ: John Wiley & Sons, 2011))
The time step in his model is one month; i.e., dt = 1/12. His Model 2 also makes three assumptions. First, the initial or current (t0) short-term rate is equal to 4.00%. Second, the annual basis-point volatility is 250 basis points. Third, the annual drift is +100 basis points. In the first step of his first trial, the random uniform variable is 0.0793 such that, via inverse transformation, the associated random standard normal value is -1.410; i.e., =NORM.S.INV(7.93%) ~= -1.410. If r(0) is 4.00%, to what level does the rate evolve in the first month, r(1/12)?
a. 2.77%
b. 2.98%
c. 3.07%
d. 4.15%
303.3. Analyst Susan is evaluating various short-term interest rate models. Her primary criteria is to AVOID a model that will produce a negative short-term rate. Each of the following approaches can help Susan avoid a model that generates negative rate outcomes EXCEPT for:
a. She can increase the convexity effect input assumption
b. She can assume a non-normal distribution; e.g., lognormally distributed rates
c. She can employ shadow rates
d. She can assume a Cox-Ingersoll-Ross (CIR) model
Answers:
