P1.T4.309. Discount factors and law of one price

[Fran]

AIMs: Define discount factor and use a discount function to compute present and future values. Define the “law of one price,” explain it using an arbitrage argument, and describe how it can be applied to bond pricing.

Questions:

309.1. The table below gives coupon rates and mid-market prices for three U.S. Treasury bonds for settlement on (as of) May 31, 2013:

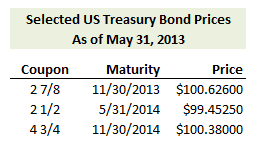
Which of the following is nearest to the implied discount function (set of discount factors) assuming semi-annual compounding?

a. d(0.5) = 0.9370, d(1.0) = 0.8667, d(1.5) = 0.9210
b. d(0.5) = 0.9920, d(1.0) = 0.9700, d(1.5) = 0.9350
c. d(0.5) = 0.9999, d(1.0) = 0.7455, d(1.5) = 0.8018
d. d(0.5) = 1.0350, d(1.0) = 1.1175, d(1.5) = 0.6487

309.2. The table below gives coupon rates and mid-market prices for two U.S. Treasury bonds, one that settles in six months and one that settles in 1.5 years. We are, however, given neither a price nor a discount factor for the 3.0% coupon bond that settles in one year:

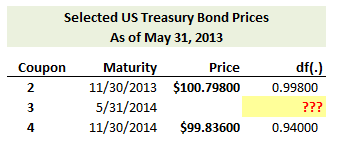
We do know that the six month discount factor, d(0.5) = 0.9980, and the 1.5 year discount factor, d(1.5) = 0.9400. According to the "law of one price," what must be the one-year discount factor, d(1.0), if we assume semi-annual compounding?:

a. 0.9157
b. 0.9631
c. 0.9800
d. 0.9947

309.3. The table below gives the prices of two out of three US Treasury notes for settlement on July 31, 2013. All three notes will mature exactly one year later on July 31, 2013. Assume annual coupon payments and that all three bonds have the same coupon payment date.

Which is nearest to the price of the 3.0% Treasury note (based on GARP's 2010 Practice Exam P1.18)?

a. $96.55
b. $99.37
c. $101.04
d. $103.28

Answers:

• Here in Forum

 

[David Harper CFA FRM]

In recent weeks, we've received several queries about Tuckman in Topic 4. This question starts a totally new series of questions devoted to Tuckman's Chapters in P1.T4. Tuckman (3rd edition). To remind, I write a new set (of three, like the above) Mon, Tue, Wed, Thur which alternative P1, P2, P1, P2. As we reach end-of-chapters, we collect into PDFs. T4 has assigned Chapters 1 to 6, so this new Tuckman series will occupy several weeks, you can see.

Question 309.3 reflects a tactic I use: while I write primarily to the AIMs, at the same time, we've collected a DB of prior GARP sample/exam questions, so my "secondary triangulation" is to mimic or approximate an actual prior exam question (that is also congruent with AIM), if we can find a match. And 309.3 is a good example, imo: it's a nice test of law of one price (I suppose) but I don't think it would be naturally suggested by the text, rather it's been employed by GARP.

We hope you enjoy them! Thanks,

 

[sm@23]

Hi @David Harper CFA FRM ref above: 309.3 as per as the price given the 1st n 3rd bond's spot rate is different. How is it possible as per as law of one price? Shouldn't the spot rate same.
PS: I know it can be solved using the cash flow equation. But trying to understand from other aspect

 

[David Harper CFA FRM]

Hi sm@23 if you click on the link to the answer (https://forum.bionicturtle.com/threads/p1-t4-309-discount-factors-and-law-of-one-price.6848/), you'll see a discussion thread about this. (Please note, in my defense, I based this on GARP's 2010 Practice Exam P1.18; I just didn't think to check it against the Law ). I think we all agreed that it is an unjustified violation of the law of one price (and I believe I did feedback to GARP); many have come to the same conclusion as you. The reason it can't be justified is that there are no confounding factors, specifically really, these are virtually risk-free instruments. Tuckman says the "law of one price [is]: absent confounding factors (e.g., liquidity, financing, taxes, credit risk), identical sets of cash flows should sell for the same price." That is, the law of one price is really applied (in Tuckman's scenarios anyway) to the risk-free spot rate curve. There are all US Treasuries maturing in one year, so the Law should apply and this question is flawed. (I think the question would work with a slight tweak: if these were credit risky bonds, where the solved-for $3.00 coupon bond was simply in-between the other two. Riskier than the $2.25 so yielding more, safer than the $4.13 so yielding less. And without the need to honor the law of one price due to credit risk variation). Thanks.

 

[sm@23]

Thanks a ton @David Harper CFA FRM

 

[juldam]

Hi David, why for 309.1 we cannot simply make -> PRICE / (1+Yield)^n, e.g. 100.62 / (1 + 0.02875)^0.5 for the first case ?
Alternatively, could you specify the valid formula to compute respective years?

 

[Rblc]

Hi David, why for 309.1 we cannot simply make -> PRICE / (1+Yield)^n, e.g. 100.62 / (1 + 0.02875)^0.5 for the first case ?
Alternatively, could you specify the valid formula to compute respective years?

Hi @juidam -
I think you know that but the coupon rate is not necessarily eq yield.By discounting by the coupon rate you are implying that the yield is equal to the coupon rate. That means that you are assuming the bond is selling at par which is isn't (see below the relationship on the wiki). You can try it on your calculator for the first bond : N= 1, I/Y =1.4375% , Coupon = $ 1.4375, FV = 100 will return a PV of 100 --> Price at Par

https://en.wikipedia.org/wiki/Yield_to_maturity#Coupon_rate_vs._YTM_and_parity

• if a bond's coupon rate is less than its YTM, then the bond is selling at a discount.
• If a bond's coupon rate is more than its YTM, then the bond is selling at a premium.
• If a bond's coupon rate is equal to its YTM, then the bond is selling at par.

it took me a long time to understand Tuckman law of 1 price because i was so used in financial math classes to discount using the yield and I feel that you might be having some of the same confusion as I am.
The way I understand it is as follow (perhaps its not entirely correct)

The law of 1 price in the FRM's 2020 reading are based on Tuckman who states that:
This reasoning is an application of the law of one price: absent confounding factors (e.g., liquidity, financing, taxes, credit risk), identical sets of cash flows should sell for the same price.

What this means is that $1 in 6 months should have the same value today for all securities . The fact that we are assuming there are no "confounding factors" ensures that if this is not true for all bonds, then there is an arbitrage to be had.

Thus the observed prices and maturity inform the discount factor for the period and any bond of the same maturity should be discounted at the same rate. If not this would mean that $1 for a bond of say 6m maturity is costing more (or less) than $1 of another bond. Thus (if we assume no confounding factors) there is an arbitrage that can be done.

Edit : (Now that I wrote all that, I see that David had already answered a similar inquiry of mine some time ago here... :
https://forum.bionicturtle.com/threads/t4-21-fixed-income-law-of-one-price.22492/#post-82864)

 

[David Harper CFA FRM]

Thank you @RajivBoolell ! @juldam If you click-through to the solution at https://forum.bionicturtle.com/threads/p1-t4-309-discount-factors-and-law-of-one-price-tuckman.6848/, you'll see that the first discount factor, d(0.5), is indeed retrieved by d(0.5) = 100.626 / [100*(1+ 2.875%/2)] = 0.99200 which is the semi-annual-frequency analog to your annual-frequency formula. This solved-for d(0.5) then becomes an input into solving for the d(1.0) per the law of one price.