Dr. Jayanthi Sankaran
Well-Known Member
Hi David,
In question (2) referenced as above,
(2) At the start of the year, a stock price is $100. A twelve-step binomial model describes the stock price evolution such that each month the extreme volatility price with either jump from S(t) to S(t)*u with 60.0% probability or down to S(t)*d with 40.0% probability. The up jump (u) = 1.1 and the down jump (d) = 1/1.1; note these (u) and (d) parameters correspond to an annual volatility of about 33% as exp[33%*SQRT(1/12)] ~= 1.10. At the end of the year, which is nearest to the probability that the stock price will be exactly $121?
(a) 0.33%
(b) 3.49%
(c) 12.25%
(d) 22.70%
Answer: D = 22.7%
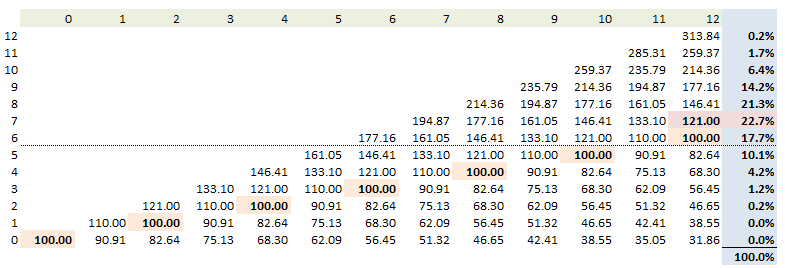
There are 13 outcomes at the end of the 12-step binomial, with $100 as the outcome that must correspond to six up jumps and six down jumps. Therefore, $121 must be the outcome due to seven up jumps and five down jumps: $100*1.1^7*(1/1.1)^5 = $121
Such that we want the binomial probability given by:
Binomial Prob [X = 7| n =12, p = 60%] = 22.70%
Clarification
Although, intuitively, 13 outcomes at the end of the 12-step binomial seems right (ie expected value of stock price at the end of 12 steps ??), how do you figure out six up jumps and six down jumps from the Binomial model to get $100 as the outcome? Also how do you get :
Binomial Prob [X = 7| n =12, p = 60%] = 22.70%
I guess I am missing something that I should understand.
Thanks!
Jayanthi
In question (2) referenced as above,
(2) At the start of the year, a stock price is $100. A twelve-step binomial model describes the stock price evolution such that each month the extreme volatility price with either jump from S(t) to S(t)*u with 60.0% probability or down to S(t)*d with 40.0% probability. The up jump (u) = 1.1 and the down jump (d) = 1/1.1; note these (u) and (d) parameters correspond to an annual volatility of about 33% as exp[33%*SQRT(1/12)] ~= 1.10. At the end of the year, which is nearest to the probability that the stock price will be exactly $121?
(a) 0.33%
(b) 3.49%
(c) 12.25%
(d) 22.70%
Answer: D = 22.7%
There are 13 outcomes at the end of the 12-step binomial, with $100 as the outcome that must correspond to six up jumps and six down jumps. Therefore, $121 must be the outcome due to seven up jumps and five down jumps: $100*1.1^7*(1/1.1)^5 = $121
Such that we want the binomial probability given by:
Binomial Prob [X = 7| n =12, p = 60%] = 22.70%
Clarification
Although, intuitively, 13 outcomes at the end of the 12-step binomial seems right (ie expected value of stock price at the end of 12 steps ??), how do you figure out six up jumps and six down jumps from the Binomial model to get $100 as the outcome? Also how do you get :
Binomial Prob [X = 7| n =12, p = 60%] = 22.70%
I guess I am missing something that I should understand.
Thanks!
Jayanthi


 and didn't worry about expressing every little detail. Whereas I am actually not so quick and i need to detail it all out. I hope that explains, thanks!
and didn't worry about expressing every little detail. Whereas I am actually not so quick and i need to detail it all out. I hope that explains, thanks!