Just a technical clarification on T5.c/Mapping a two-bond portfolio (Jorion 11-2)

[sleepybird]

Hi David,
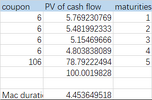
In your T5.c video there was an example on mapping a two-bond portfolio where you mapped the duration to 2.73 yrs. Can you clarify that the 2.73 yrs is the Macaulay duration (i.e., the column should be labeled Macaulay Duration)?

I am still very confused with which (Mac or Mod) duration is defined as the weighted average maturity of the bond? I think it is the Mac duration, that's why I suggest the 2.73 yrs is the Mac duration?

Thanks.

 

[David Harper CFA FRM]

Hi sleepybird,

Macaulay duration is (definitely) the weighted average maturity of the bond; Mac duration is computed as such, including in our learning XLS.

Re T5.c video, see label, that is Jorion's Table 11-2. Discussion is here at http://forum.bionicturtle.com/threads/l2-t5-63-fixed-income-mapping.3617/#post-9682
... i had assumed it is a modified duration
... Jorion does not appear to specify
... i have not had time to re-compute, will do when i get a chance
... however, @[email protected] claims that he replicated the 2.733 years as a Macaulay and he is quite expert (more expert than me, in many respects) so I do trust that. So, your intuition agrees with him. I'll post up when I've gotten a chance to compute both and see if i can replicates the 2.733 (when i tried before, i did not get 2.733 exactly under either.).

 

[sleepybird]

I did not get 2.733 either. I got 2.727.

https://www.dropbox.com/s/t6110d97nu2f6tt/VaR Mapping Jorion 11-2.xlsx

 

[FLIN1386]

Hi David,

I encountered the same issue where I also obtained a Macaulay duration of 2.727.
I watched the YouTube video provided by BT, titled "T5-05: Value (VaR) Mapping a Fixed-Income Portfolio". I believe the issue lies in the duration calculation for the 5-year 6% coupon bond, which I calculated to be 4.454.

Mac duration = (5.769*1 + 5.482*2 +5.155 * 3 + 4.804*4 + 78.792*5)/100 = 4.454
Cash flow calculation details attached.

I am unsure about the cause of the difference between 2.733 and 2.727. I hope it is only due to a precision issue in the calculations.

 

[Clay Carter]

Hi David,

I encountered the same issue where I also obtained a Macaulay duration of 2.727.
I watched the YouTube video provided by BT, titled "T5-05: Value (VaR) Mapping a Fixed-Income Portfolio". I believe the issue lies in the duration calculation for the 5-year 6% coupon bond, which I calculated to be 4.454.

Mac duration = (5.769*1 + 5.482*2 +5.155 * 3 + 4.804*4 + 78.792*5)/100 = 4.454
Cash flow calculation details attached.

I am unsure about the cause of the difference between 2.733 and 2.727. I hope it is only due to a precision issue in the calculations.

@FLIN1386 Do you mind sharing a link to your spreadsheet?