You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Jorion - Component Var : Matrix multiplication going wrong
- Thread starter rajeshtr
- Start date
HI @rajeshtr
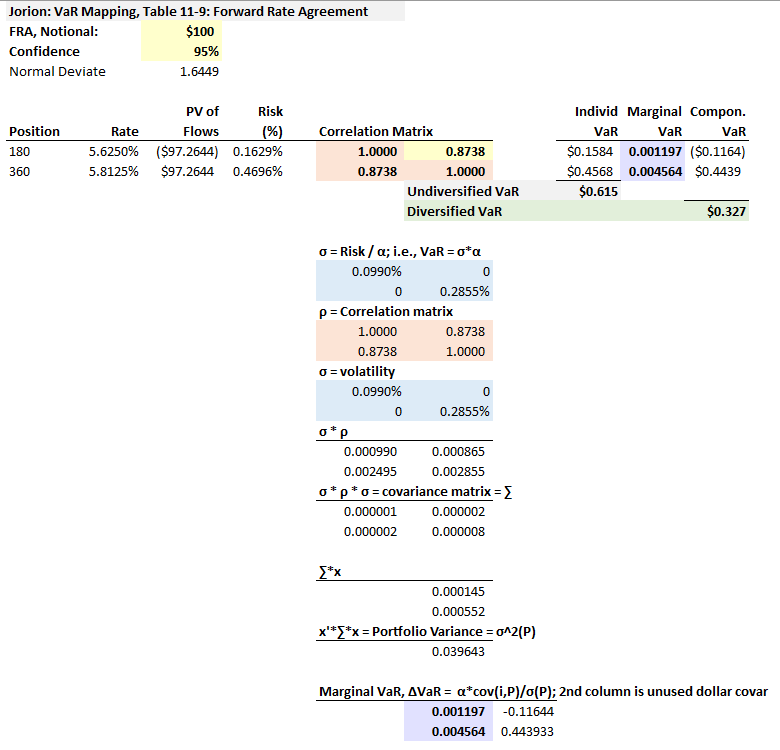
Please see this one sheet workbook that I just detached for you https://www.dropbox.com/s/srglvldxh0ymxuy/Jorion-table-11-9-FRA.xlsx?dl=0 (screenshot below)
This is my replication of Jorion's table. I added some labels and color to my derivation of the marginal VaR which is explicated in the vertical column and finally when solved colored in purple (at the bottom, then pulled up for use in the exhibit).
There is some matrix multiplication, first, to retrieve the covariance matrix from the correlations (themselves inferred from the risk%) and volatility; second to compute the portfolio variance.
Component VaR = marginal VaR * $position; there are three definitions of component VaR, this is the most intuitive (component VaR scales unitless marginal to the appropriate units of the position)
The only difficult calculation here, IMO, is the marginal VaR that you are asking about (purple cells H36 and H37). I used marginal VaR, ΔVaR = α*cov(i,P)/σ(P). I hope that helps, good luck, a deep dive on this takes a bit of time.
Please see this one sheet workbook that I just detached for you https://www.dropbox.com/s/srglvldxh0ymxuy/Jorion-table-11-9-FRA.xlsx?dl=0 (screenshot below)
This is my replication of Jorion's table. I added some labels and color to my derivation of the marginal VaR which is explicated in the vertical column and finally when solved colored in purple (at the bottom, then pulled up for use in the exhibit).
There is some matrix multiplication, first, to retrieve the covariance matrix from the correlations (themselves inferred from the risk%) and volatility; second to compute the portfolio variance.
Component VaR = marginal VaR * $position; there are three definitions of component VaR, this is the most intuitive (component VaR scales unitless marginal to the appropriate units of the position)
The only difficult calculation here, IMO, is the marginal VaR that you are asking about (purple cells H36 and H37). I used marginal VaR, ΔVaR = α*cov(i,P)/σ(P). I hope that helps, good luck, a deep dive on this takes a bit of time.

@zamz00 same as above which is merely the 2-asset instance of an n-asset portfolio. Portfolio variance given by matrix multiplication x(T)*Σ *x where x is column vector of (in this case) PV of Flows, Σ is covariance matrix (given by the matrix product σ*ρ*σ), and x(T) is transposed vector.
@zamz00 One solution to component VaR is Individual VaR * ρ(i, Portfolio); since correlation cannot exceed 1.0, the upper limit on component VaR should be individual VaR. Some discussion here https://forum.bionicturtle.com/threads/component-versus-incremental-value-at-risk-var-level-2.4961/ (it's a different issue w.r.t incremental VaR)
Also https://forum.bionicturtle.com/thre...nent-value-at-risk-var-jorion.4779/post-70165 i.e.,
Also https://forum.bionicturtle.com/thre...nent-value-at-risk-var-jorion.4779/post-70165 i.e.,
Hi @Marco.Musci Component VaR can be calculated three ways ( although, importantly, this are internally consistent: they always give the same answer as demonstrated by my worksheet in https://learn.bionicturtle.com/topic/learning-spreadsheet-jorion-chapter-17/ ), where CVaR = component VaR:
If you look at the last calculation above, the upper limit on the correlation between the position and the portfolio is 1.0; therefore the position's CVaR must be less than (or equal to) the position's individual VaR. It's possible that my statement is imprecise and should be "component VaRs must be LESS than (or equal to) individual VaR" but I'm not sure about that: as I experiment with the numbers, as long as I have another position, the correlation is less than 1.0. Even if (eg) my positions are $5,000 and $10 (99.80% and 0.20%), the correlation is 0.9999970, and the statement is strictly true! I hope that's helpful,
- CVaR = VaR_marginal * $Position
- CVaR = $VaR_portfolio* %position_weight * β(position, portfolio)
- CVaR = $VaR_individual * ρ(position, portfolio)
Similar threads
- Replies
- 0
- Views
- 419
- Replies
- 2
- Views
- 1K

