chatty06
New Member
Hi All,
Can someone confirm the correct Jensen's Alpha formula? I've seen two versions used throughout the material and I don't understand why. Maybe I'm just overlooking something. One version adds the Risk Free Rate to the Price of Risk while the other one subtracts it. Examples below
Version 1 = Expected Return (portfolio) - (Risk Free Rate + Beta (portfolio) [Expected Return (Market) - Risk Free Rate]
7:12 minutes into the Instructional Video: Amenc, Chatper 4.
Version 2 = Expected Return (portfolio) - (Risk Free Rate - Beta (portfolio) [Expected Return (Market) - Risk Free Rate]
PG 3 of R9.P1.T1.Amenc_v7_592b10f43df06.pdf
Can someone confirm the correct Jensen's Alpha formula? I've seen two versions used throughout the material and I don't understand why. Maybe I'm just overlooking something. One version adds the Risk Free Rate to the Price of Risk while the other one subtracts it. Examples below
Version 1 = Expected Return (portfolio) - (Risk Free Rate + Beta (portfolio) [Expected Return (Market) - Risk Free Rate]
7:12 minutes into the Instructional Video: Amenc, Chatper 4.
Version 2 = Expected Return (portfolio) - (Risk Free Rate - Beta (portfolio) [Expected Return (Market) - Risk Free Rate]
PG 3 of R9.P1.T1.Amenc_v7_592b10f43df06.pdf


 e.g., see below the explication of alpha in the new note:
e.g., see below the explication of alpha in the new note:

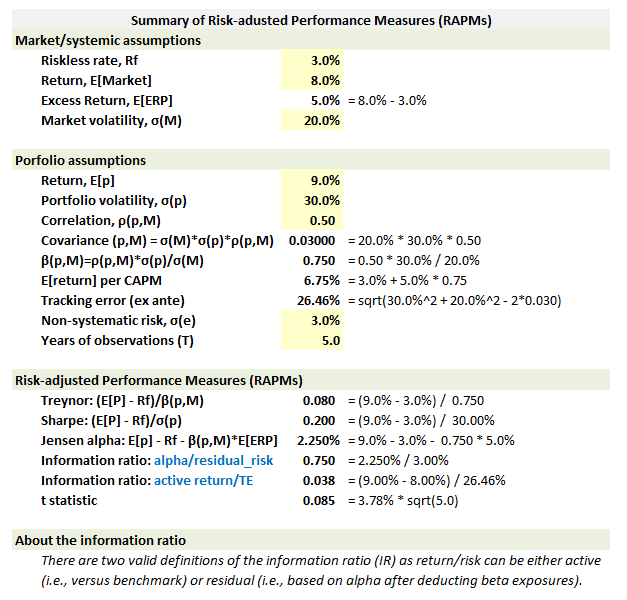
 ) however it earned 9.0%, which is therefore generally unexplained by CAPM's single-factor view and we will call this vertical distance from the SML alpha (aka, residual), which is here + 2.25%." In this way, CAPM is just exploited to help us measure (evaluate). I don't think it has any further claim on the interpretation of the +2.25%. It can be some combination of: luck (random sampling), skill, nonsystematic risk (that did not actually get diversified away), or very likely: non-market factor exposure (hidden beta), like a momentum or value factor. I hope that's helpful!
) however it earned 9.0%, which is therefore generally unexplained by CAPM's single-factor view and we will call this vertical distance from the SML alpha (aka, residual), which is here + 2.25%." In this way, CAPM is just exploited to help us measure (evaluate). I don't think it has any further claim on the interpretation of the +2.25%. It can be some combination of: luck (random sampling), skill, nonsystematic risk (that did not actually get diversified away), or very likely: non-market factor exposure (hidden beta), like a momentum or value factor. I hope that's helpful!