[email protected]
New Member
Hello:
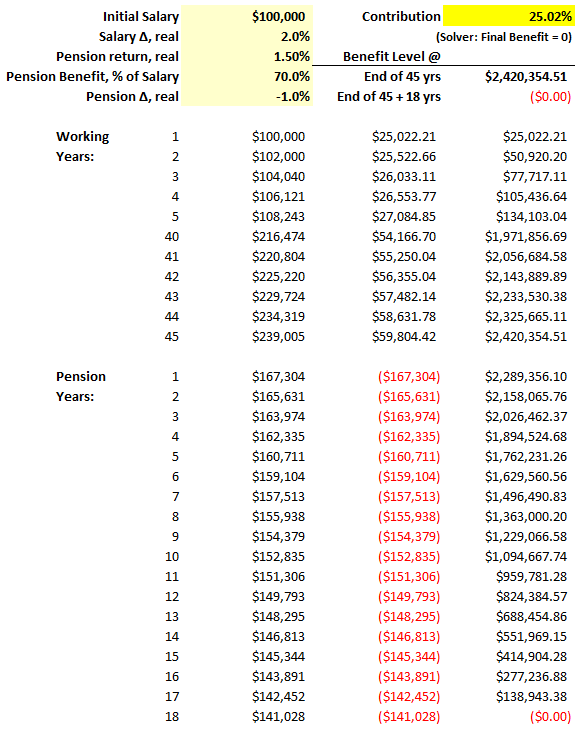
The answer to this question states: "The spreadsheet is used in conjunction with Solver..."; I can't find the Excel' spreadsheet with the Solver problem.
I could go ahead and do it...but, I do not want to re- invent the wheel...Please let me know if anyone has it, or if was it supposed to be included in the paid materials...or if I should go straight ahead and prepare the solution and posted here?
The answer to this question states: "The spreadsheet is used in conjunction with Solver..."; I can't find the Excel' spreadsheet with the Solver problem.
I could go ahead and do it...but, I do not want to re- invent the wheel...Please let me know if anyone has it, or if was it supposed to be included in the paid materials...or if I should go straight ahead and prepare the solution and posted here?