Hi!
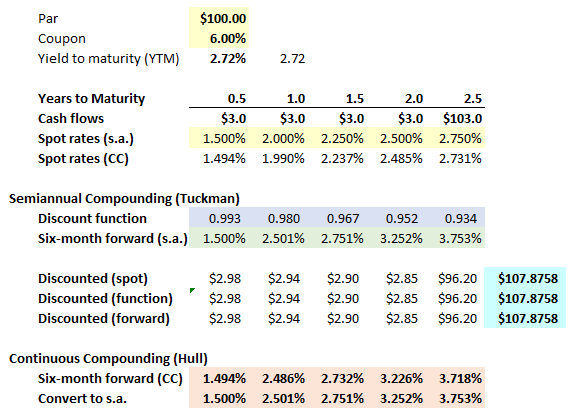
I'm confused about forward interest rate calculation, Hull (ch 4) uses RF=(R2T2-R1T1)/(T2-T1), Tuckman (ch 2) instead computes from formula (1+r(0,2)/2)^4=(1+r(0,1.5)/2)^3+(1+f(1.5,2.0)/2)^1. I'm sure the answer is just here but I can't see... Is it about compounding? Should I memorize both formulas or can I just be happy with Hull's formula for the exam?
Thank you!
PS David, sorry about personal alert. As I am a Firefox user, I could not post my message into forum. Later I found out that it is more allowed to an IE user So I'll post it for you.
So I'll post it for you.
I'm confused about forward interest rate calculation, Hull (ch 4) uses RF=(R2T2-R1T1)/(T2-T1), Tuckman (ch 2) instead computes from formula (1+r(0,2)/2)^4=(1+r(0,1.5)/2)^3+(1+f(1.5,2.0)/2)^1. I'm sure the answer is just here but I can't see... Is it about compounding? Should I memorize both formulas or can I just be happy with Hull's formula for the exam?
Thank you!
PS David, sorry about personal alert. As I am a Firefox user, I could not post my message into forum. Later I found out that it is more allowed to an IE user
 So I'll post it for you.
So I'll post it for you.