You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Explain Correlation-weighted HS example
- Thread starter frogs
- Start date
HI @frogs It is straight-up (literally) from Dowd's example in his Chapter 4. Matrix A is defined by Choleski's decomposition; in Dowd's Chapter 8 he more clearly defines it
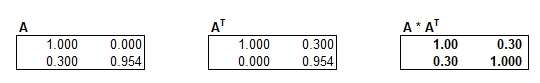
so if the historical correlation, rho = 0.30, then you can below (my super quick XLS below) how A*A^T = correlation matrix; i.e., my a(22) = sqrt(1- 0.30^2) = 0.954.
Then A^(-1) is is just using the desired rho = 0.90 as merely a given (arbitrary in this example, where the point is to "filter" the returns vector with a higher correlation). So he ends up with a matrix that is the product, A*A^(T); e.g., row 2, column 2 = [ 0.90 * 0 + sqrt(1 - 0.90^2) * 1 ] * [1/ (sqrt(1 - 0.30^2)] = 0.4569. This matrix multiplies by (aka, "filters") the actual historical return vector (from the 0.30 correlation) to a desired correlation of 0.90. If i get time next week, I'll share an XLS, there's just no way shortcut around the matrix math in Dowd if you really want to follow Cholesky's decomposition,. Thanks,
so if the historical correlation, rho = 0.30, then you can below (my super quick XLS below) how A*A^T = correlation matrix; i.e., my a(22) = sqrt(1- 0.30^2) = 0.954.

Then A^(-1) is is just using the desired rho = 0.90 as merely a given (arbitrary in this example, where the point is to "filter" the returns vector with a higher correlation). So he ends up with a matrix that is the product, A*A^(T); e.g., row 2, column 2 = [ 0.90 * 0 + sqrt(1 - 0.90^2) * 1 ] * [1/ (sqrt(1 - 0.30^2)] = 0.4569. This matrix multiplies by (aka, "filters") the actual historical return vector (from the 0.30 correlation) to a desired correlation of 0.90. If i get time next week, I'll share an XLS, there's just no way shortcut around the matrix math in Dowd if you really want to follow Cholesky's decomposition,. Thanks,
Similar threads
- Replies
- 0
- Views
- 1K
- Replies
- 0
- Views
- 2K
- Replies
- 0
- Views
- 2K
- Replies
- 0
- Views
- 1K
