Errors Found in Study Materials P1.T3. Financial Markets & Products (OLD thread)
- Thread starter Nicole Seaman
- Start date
-
- Tags
- errors
- Status
P1.T3. Financial Markets & Products, Reading 19 Hull, Page 79:
- “Normal backwardation” and “normal contango” refer to an unobserved relationship between the spot price and the expected future spot price.
Hi @juhsu Yes, you are absolutely correct on both, they are both typos. Thank you! (@Nicole Seaman per wrike, I fixed these in updated, unpublished version 7.3.2)
Hi @juhsu They are the same, see https://www.dropbox.com/s/ufxk7hndpdmib1s/0801-hull-3-16.xlsx?dl=0 and see below. He is looking for the unconditional survival probability and yours is (to me) the more intuitive approach: unconditional (aka, joint) survival to the end of year 2 = (year 1 conditional survival) * (year 2 conditional survival). But it's actually the same as his, because you can subtract unconditional probability (dying), or mathematically:
- (1 - 0.011046) × (1 - 0.011835) = (1 - 0.011046) - (0.011835 - 0.011835*0.011046) = 1 - 0.011046 - 0.0117043. I hope that helps!
Updated by Nicole to note that the reading in reference is Reading 18, John Hull, Chapter 4 (please make sure to note which reading you are referencing, as there are many readings in Topic 3 of the curriculum)
Consider an example where a fund of hedge funds divides its money equally between 10
hedge funds. All hedge funds charge 2 plus 20% and the fund of hedge funds charges 1 plus
10%. While it may seem that the investor pays 3 plus 30%, the actual fee can be even
higher. Consider a scenario where five of the hedge funds lose 40% before fees and the
other five make 40% before fees.
Each of the profitable hedge funds would collect an incentive fee of 20% of the profit of 38%:
0.2 × (40 – 2) = 7.6 %
The total incentive fee is (7.6% × 5)/10 = 3.8% of the funds invested.
Considering the 2% annual fee paid to the hedge funds and 1% annual fee paid to the fund
of funds, the net return of the investor is – (3+ 3.8) = –6.8% of the amount invested. This
means the return after fee is 6.8% less than the return on the underlying assets before fees.
Incentive fee from profitable hedge fund should be 7.6*5, why are we dividing it with 10? Why are we finding total incentive fee?
It should be Incentive fee of 7.6*5 =38%
service fee 3*5= 15
total 53%
total earned my investor 40*5=200%
remaining after all fees 200-53= 147%
even if my calculation seems to be wrong but I feel something is wrong in calculation done in notes. The calculation is present on Page 42 under the learning objective "Calculate the return on a hedge fund investment and explain the incentive fee structure of a hedge fund including the terms hurdle rate, high-water mark, and claw back".
Consider an example where a fund of hedge funds divides its money equally between 10
hedge funds. All hedge funds charge 2 plus 20% and the fund of hedge funds charges 1 plus
10%. While it may seem that the investor pays 3 plus 30%, the actual fee can be even
higher. Consider a scenario where five of the hedge funds lose 40% before fees and the
other five make 40% before fees.
Each of the profitable hedge funds would collect an incentive fee of 20% of the profit of 38%:
0.2 × (40 – 2) = 7.6 %
The total incentive fee is (7.6% × 5)/10 = 3.8% of the funds invested.
Considering the 2% annual fee paid to the hedge funds and 1% annual fee paid to the fund
of funds, the net return of the investor is – (3+ 3.8) = –6.8% of the amount invested. This
means the return after fee is 6.8% less than the return on the underlying assets before fees.
Incentive fee from profitable hedge fund should be 7.6*5, why are we dividing it with 10? Why are we finding total incentive fee?
It should be Incentive fee of 7.6*5 =38%
service fee 3*5= 15
total 53%
total earned my investor 40*5=200%
remaining after all fees 200-53= 147%
even if my calculation seems to be wrong but I feel something is wrong in calculation done in notes. The calculation is present on Page 42 under the learning objective "Calculate the return on a hedge fund investment and explain the incentive fee structure of a hedge fund including the terms hurdle rate, high-water mark, and claw back".
Last edited by a moderator:
Hi @Jaskarn That's Hull's actual example (in the text) but, to check it, I input the assumptions in the same model used for his EOC Question 4.17 (because it inspired my similar question T3.706.1 @ https://forum.bionicturtle.com/threads/p1-t3-706-hedge-funds-hull.10293/ ) and please note the text does seem to be correct, to the extent it inherits Hull's assumptions (for example, he charges the 2.0% management fee as a percentage of beginning assets). Here is my XLS at https://www.dropbox.com/s/ndisful2rid460q/0814-hfof-fees.xlsx?dl=0 but hopefully the screenshot below will be sufficient. Please note:
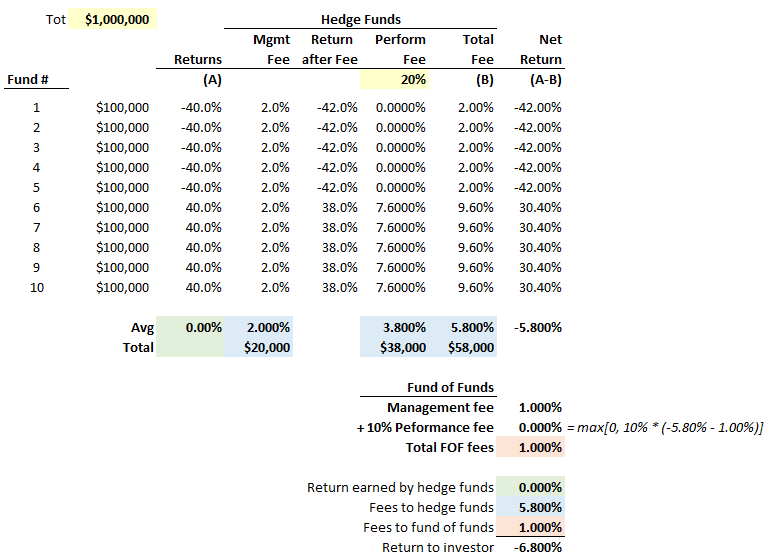
- The 3.8% refers the percentage of all 10 invested funds; e.g., if $1.0 million is the overall allocation equally to 10 funds, then 7.6% to 5 of them is $38,000 in performance fees, which is 3.8% of $1.0 (all ten funds), so it's "weighted average" (of invested) performance fee.
Hi David,
reading 19. Page 146:
"If the stock rises significantly, the call option will not be exercised; however, we have the underlying to protect ourselves from that scenario."
What I know is that when the price increased, the call holder will have an incentive to exercise --- call payoff= max(spot price - strike price, 0). However, in the note is the opposite! It says that it will NOT be exercised.
Am I missing something?
Thanks
reading 19. Page 146:
"If the stock rises significantly, the call option will not be exercised; however, we have the underlying to protect ourselves from that scenario."
What I know is that when the price increased, the call holder will have an incentive to exercise --- call payoff= max(spot price - strike price, 0). However, in the note is the opposite! It says that it will NOT be exercised.
Am I missing something?
Thanks
Hi @must854 No you are missing nothing. Sorry, it's just a blatant typo. Thank you for paying attention. In addition to the typo, on reflection, I think both paragraphs here are muddled and can be improved. (cc: @Nicole Seaman non-urgent revision, as this is a long document, would prefer to wait for next batch revision). Propose Page 146:
From current two bullets (incl. mistake) :
"This trade reflects a neutral to bullish outlook.
"This trade reflects a neutral to bullish outlook.
From current two bullets (incl. mistake) :
"This trade reflects a neutral to bullish outlook.
- "If the stock rises significantly, the call option will not be exercised; however, we have the underlying to protect ourselves from that scenario.
- On the other hand, by writing a call option we collect the premium. Thus, if the stock price stays about the same or rises modestly, we collect the premium. We can then use this strategy to roll over the options each month, collecting our premium"
"This trade reflects a neutral to bullish outlook.
- "If the stock price increases modestly (assuming the strike exceeds the current price), the covered call writer enjoys the interim capital appreciation supplemented by the option premium. If the stock price hovers or rises modestly, this strategy can be rolled over (e.g., buy to close original options and sell new options at higher price/later expiration), generating a stream of income."
- If the stock increases significantly, the strike price will be breached and the written call option will be exercised such that the trade forfeits further profits. This strategy enhances income but limits the upside.
- On the other hand, if the stock price decreases then the option will not be exercised, and the collected option premium provides a modest hedge to the stock's capital depreciation. As Hull notes, put-call parity shows that the price exposure from writing a covered call is the same as the exposure from writing a naked put."
Last edited by a moderator:
ziminli1228
Member
In the study notes of Hull chapter 4 p10, the chart is a long call not a long put as stated in the questions?
Hi @ziminli1228 We previously did have a typo on page 10, but it has already been fixed: the "Assumptions" table now reads: Put option strike, K: $27.50 ... to reflect that the hedge is put options ... and the associated text reads:
.... so the payoff of the put itself is not displayed, but rather what is displayed here is the net position of [stock + put] in the green, as the net position. I trust you'll see this if you download the latest version of the Hull note. Thanks!
Hi David,
Can you help me reconcile your example in the Hull pdf on Eurodollars against the below Investopedia example which states that short position gains when the quote decreases. The example from Investopedia makes intuitive sense to me, that the short gains by selling the contract at higher quote (lower rate) and then covering it at settlement when the quote is lower (higher rate). That is sell high (short position) cover later when price decline -->short profits.
In your example, the investor buys at 99.725 and it settles at 99.615 but you say that the long profits.
Example (pg .93 out of 182 in pdf): "In May 2013, an investor buys a Eurodollar contract at quote of ...
The difference between this initial and final contract price is the gain of $275 on this long position : 999,312.5 - 990,037.5 = $275"
vs this example from investopedia :
(https://www.investopedia.com/articl...4/introduction-trading-eurodollar-futures.asp)
"For example: Say a company knows in September that it will need to borrow $8 million in December to make a purchase. Recall that each eurodollar futures contract represents a $1,000,000 time deposit with a three month maturity. The company can hedge against an adverse move in interest rates during that three month period by short selling 8 December Eurodollar futures contracts, representing the $8 million needed for the purchase.
The price of eurodollar futures reflect the anticipated London Interbank Offered Rate (LIBOR) at the time of settlement, in this case, December. By short selling the December contract, the company profits from upward movement in interest rates, reflected in correspondingly lower December eurodollar futures prices.
Let's assume that on September 1, the December eurodollar futures contract price was exactly $96.00, implying an interest rate of 4.0%, and that at the expiry in December the final closing price is $95.00, reflecting a higher interest rate of 5.0%. If the company had sold 8 December Eurodollar contracts at $96.00 in September, it would have profited by 100 basis points (100 x $25 = $2,500) on 8 contracts, equaling $20,000 ($2,500 x 8) when it covered the short position.
In this way, the company was able to offset the rise in interest rates, effectively locking in the anticipated LIBOR for December as it was reflected in the price of the December Eurodollar contract at the time it made the short sale in September."
Can you help me reconcile your example in the Hull pdf on Eurodollars against the below Investopedia example which states that short position gains when the quote decreases. The example from Investopedia makes intuitive sense to me, that the short gains by selling the contract at higher quote (lower rate) and then covering it at settlement when the quote is lower (higher rate). That is sell high (short position) cover later when price decline -->short profits.
In your example, the investor buys at 99.725 and it settles at 99.615 but you say that the long profits.
Example (pg .93 out of 182 in pdf): "In May 2013, an investor buys a Eurodollar contract at quote of ...
The difference between this initial and final contract price is the gain of $275 on this long position : 999,312.5 - 990,037.5 = $275"
vs this example from investopedia :
(https://www.investopedia.com/articl...4/introduction-trading-eurodollar-futures.asp)
"For example: Say a company knows in September that it will need to borrow $8 million in December to make a purchase. Recall that each eurodollar futures contract represents a $1,000,000 time deposit with a three month maturity. The company can hedge against an adverse move in interest rates during that three month period by short selling 8 December Eurodollar futures contracts, representing the $8 million needed for the purchase.
The price of eurodollar futures reflect the anticipated London Interbank Offered Rate (LIBOR) at the time of settlement, in this case, December. By short selling the December contract, the company profits from upward movement in interest rates, reflected in correspondingly lower December eurodollar futures prices.
Let's assume that on September 1, the December eurodollar futures contract price was exactly $96.00, implying an interest rate of 4.0%, and that at the expiry in December the final closing price is $95.00, reflecting a higher interest rate of 5.0%. If the company had sold 8 December Eurodollar contracts at $96.00 in September, it would have profited by 100 basis points (100 x $25 = $2,500) on 8 contracts, equaling $20,000 ($2,500 x 8) when it covered the short position.
In this way, the company was able to offset the rise in interest rates, effectively locking in the anticipated LIBOR for December as it was reflected in the price of the December Eurodollar contract at the time it made the short sale in September."
Last edited:
Hi @lRRAngle Thank you, I do apologize but our text has a mistake. The numbers look okay, but the May purchase at 99.725 is met with a decline to 99.615 in June, so the study note should read: "The difference between this initial and final contract price is the loss of $275 on this long position: 990,037.50 - 999,312.50 = -$275.00" (even the second number is incorrect in the text).
I can see that we have a new exhibit (see below) staged for the next revision, which correctly shows the loss associated with the price (and quote) decline.
So Investopedia is correct. Eurodollar futures contracts are like bonds. Price (which includes both the Quote and the Contract Price) is inversely related to Rate (in the case of quote by Quote = 1 - Rate) such that, like a bond, the buyer profits on a rate decline (i.e., as the quote/price increase), and a short profits on a rate increase (as the quote/price decreases). Sorry for the confusion. We will clean this up in the next revision. Thank you!
FIXED IN PDF v9
I can see that we have a new exhibit (see below) staged for the next revision, which correctly shows the loss associated with the price (and quote) decline.
So Investopedia is correct. Eurodollar futures contracts are like bonds. Price (which includes both the Quote and the Contract Price) is inversely related to Rate (in the case of quote by Quote = 1 - Rate) such that, like a bond, the buyer profits on a rate decline (i.e., as the quote/price increase), and a short profits on a rate increase (as the quote/price decreases). Sorry for the confusion. We will clean this up in the next revision. Thank you!
FIXED IN PDF v9
Last edited by a moderator:
@David Harper CFA FRM: I dont like to pester about the error in the notes which might be highlighted by many more people in the forum. If you look at page no. 55 of Hull study notes you will find that the last calculation of page 55 is not converting given CC rates to Semi Annual rates,but it is directly taking CC rates and calculating Semi Annual Forward rate. I think the calculation should first convert the CC rates to Semi Annual rates, after this conversion we should use the given formula to calculate the semi annual forward rate. I am pasting precisely which calculation I am talking about below.
Thanks
Abhishek
Thanks
Abhishek
@Abchaudh
Please note that i moved your post to this thread, which we created specifically for our members to report errors in the study notes. There is a thread like this under each topic section in the forum. We appreciate our members pointing out any errors that they find, so please do not ever hesitate to point them out to us. It helps us out a lot, as we take pride in making sure that our study materials are accurate and free of errors.
Thank you,
Nicole
Hi @David Harper CFA FRM, @Nicole Seaman,
On page no. 138, the formula for the convexity term is ΔP/P = ... 0.5*C*Δy^2; in this case, 0.5*30^2*0.0035 = 0.55125%. I think you have to correct the formula in notes as well. The formula should read "0.5*30^2*0.0035^2 = 0.55125%" in the notes. The formula has been corrected by David in forum answers but the Notes version is still showing the incorrect calculation.
On page no. 138, the formula for the convexity term is ΔP/P = ... 0.5*C*Δy^2; in this case, 0.5*30^2*0.0035 = 0.55125%. I think you have to correct the formula in notes as well. The formula should read "0.5*30^2*0.0035^2 = 0.55125%" in the notes. The formula has been corrected by David in forum answers but the Notes version is still showing the incorrect calculation.
Hello @Abchaudh
I found the error you are referring to on page 138 of the practice question set, but I am not seeing this in the study notes (I may be missing it??). The error is already tagged for revision here for the practice question set: https://forum.bionicturtle.com/thre...term-structure-theories-hull-chapter-4.10596/. Note that we tag non-urgent revisions, as we cannot update all of them immediately. Please let me know if you are seeing this in the study notes, and I can add it to my list of revisions for the notes as well.
Thank you,
Nicole
@Nicole Seaman Agreed, I just checked R19 Hull Study Note to see if we used PQ T3.715.2 in the Notes, but we didn't so neither do I understand the reference to the notes. Thanks,
Hi @David Harper CFA FRM , @Nicole Seaman,it is my bad the error is in question set of Hull page no.138. Sorry I wrongly tagged it as a error in notes but it is in Question set. If you look at the highlighted text in the below document it says 0.5*30^2*0.0035 = 0.55125% . But it should read "0.5*30^2*0.0035^2 = 0.55125%".
Sorry once again for my mistake to give the reference of the document.
Thanks,
Abhishek
Sorry once again for my mistake to give the reference of the document.
Thanks,
Abhishek
Last edited by a moderator:
- Status
Similar threads
- Sticky
- Sticky
- Sticky
- Sticky
