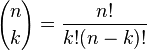Swarnendu Pathak
New Member
Hi,
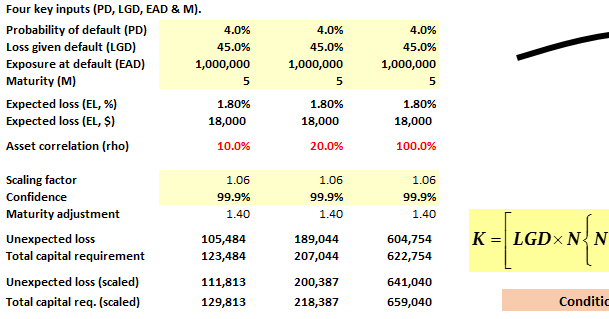
In the chapter Portfolio credit Risk (Allan M Malz) regarding the CVaR it is mentioned that when the PD is less then the significance level, then CVaR would be (-) ve or there would be a gain instead of loss as extreme loss is Zero, and if PD is more than significance level then CVaR is EAD*(1-Recovery Rate) minus LDG*PD (expected loss). Please explain when PD is less than significance level why extreme LGD is zero??
Thanks
In the chapter Portfolio credit Risk (Allan M Malz) regarding the CVaR it is mentioned that when the PD is less then the significance level, then CVaR would be (-) ve or there would be a gain instead of loss as extreme loss is Zero, and if PD is more than significance level then CVaR is EAD*(1-Recovery Rate) minus LDG*PD (expected loss). Please explain when PD is less than significance level why extreme LGD is zero??
Thanks