Kavita.bhangdia
Active Member
Hi David,
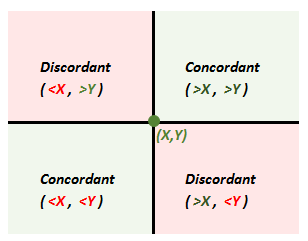
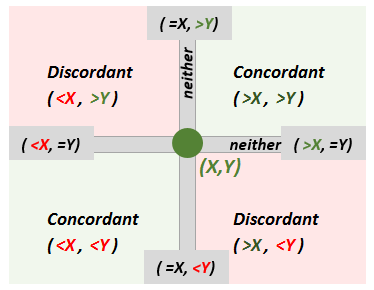
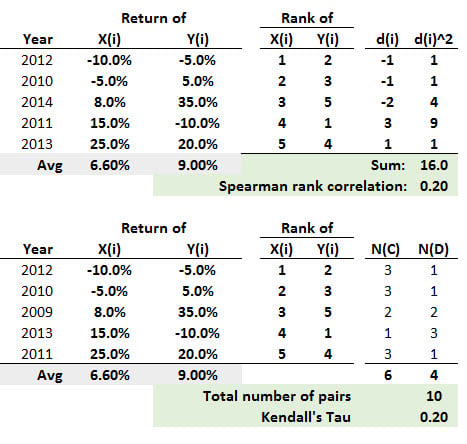
Please can you explain me concordant and discordant values in English..? I mean how to look for those values.. In the meisener reading you have represented it mathematically and I am not able to understand that.
Thanks
Kavita
Please can you explain me concordant and discordant values in English..? I mean how to look for those values.. In the meisener reading you have represented it mathematically and I am not able to understand that.
Thanks
Kavita

 )
)