Hi All,
The following is the question:
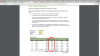
203.2. The cash prices of six-month and one-year Treasury bills are $99.00 and $98.00, respectively. An eighteen month (1.5 year) bond that will pay $1.00 coupon every six months (i.e., coupon rate of 2.0% per annum payable semi-annually) currently sells for $97.00. Which is nearest to the 1.5 year zero rate with continuous compounding? (variation on Hull 4.23)
a) 2.84% b) 3.75% c) 4.06% d) 5.39%
Could you help me understand the flaw in my logic? In order to replicate a 1.5 Y zero coupon bond, you could buy the 18M bonds, as well as sell 1% of the two treasuries. This results in zero cash flow at each of the treasury maturities (pay out 1 for the treasury maturing, receive 1 for the coupon) and a final cashflow of $100.
The cost of this set up is $97 - $.99 - $.98 = $95.03
Therefore 95.03e^(1.5*r)=100
e = 3.4%
Which is clearly wrong, but I can't quite figure out what I did wrong. Is this method incorrect, or did I make a mistake along the way?
The following is the question:
203.2. The cash prices of six-month and one-year Treasury bills are $99.00 and $98.00, respectively. An eighteen month (1.5 year) bond that will pay $1.00 coupon every six months (i.e., coupon rate of 2.0% per annum payable semi-annually) currently sells for $97.00. Which is nearest to the 1.5 year zero rate with continuous compounding? (variation on Hull 4.23)
a) 2.84% b) 3.75% c) 4.06% d) 5.39%
Could you help me understand the flaw in my logic? In order to replicate a 1.5 Y zero coupon bond, you could buy the 18M bonds, as well as sell 1% of the two treasuries. This results in zero cash flow at each of the treasury maturities (pay out 1 for the treasury maturing, receive 1 for the coupon) and a final cashflow of $100.
The cost of this set up is $97 - $.99 - $.98 = $95.03
Therefore 95.03e^(1.5*r)=100
e = 3.4%
Which is clearly wrong, but I can't quite figure out what I did wrong. Is this method incorrect, or did I make a mistake along the way?

 !
!