Binomial Options Pricing Model and Futures

[nikogeorgiev]

Hi David

I am not quite sure why when calculating the risk neutral probability we do not use the risk free rate. Would you please shortly explain that? I note that it costs nothing to take short or long position in a futures contract but that just confuses me even more...

Apologies for the basic question.

Kind regards
Niko

 

[David Harper CFA FRM]

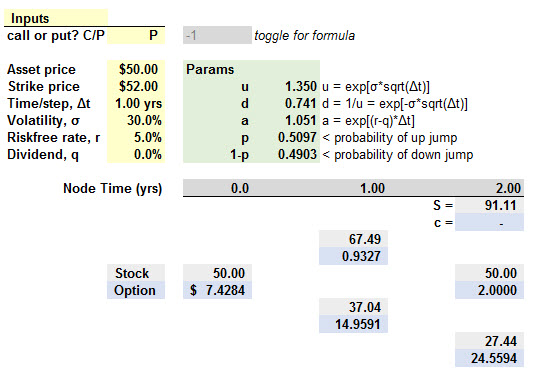
Hi @nikogeorgiev No worries, it's not basic, the risk-neutral probability concept is difficult. But can you illustrate with an example? Only because I'm not sure exactly what you mean, sorry The reason I say that is that, at least in the context of the binomial option pricing model (per your title) the risk-neutral probability, by definition, does use the risk-free rate to discount. Just to use Hull's Figure 13.9 as an example (our version of the same is below); this is a 2-year American put where S = 50, K = 52, σ = 30% and Rf = 5.0%. This model derives (u) and (d) as functions of the volatility; in Hull's phrasing, this "matches" volatility with (u) and (d). The p (probability of an up movement) is a function of these (u) and (d) values. In this way, there can be properly called risk-neutral probabilities because it is the probability that the asset will jump up (u) in a risk-neutral world (the world called "imaginary" by Tuckman) where investors are not risk averse such that assets do not require a risk premium. In this world (i.e., under the assumption of these probabilities) we are consistent to discount at the risk-free rate. This is why I am suggesting that risk-neutral probabilities do utilize the risk-free rate. (As Hull briefly mentions, it is consistent with the real-world because in the real-world a higher expected return is offset by a higher discount rate). Now, the next usage of risk-neutral probabilities is discussed more in Tuckman than Hull (but still consistent with Hull): that is where the probabilities in the tree (u and d) are adjusted so that the expected discounted value matches the observed market price (aka, arbitrage price); this informs the risk-neutral probabilities with the market's consensus view of the option's actual risk. But note, these probabilities inform future notes that continue to discounted at the risk-free rate. I think sometimes the more difficult question for new students, in this context is: why are we discounting risky instruments at the risk-free rate? Let me know if that's helpful? Thanks!