Head on over to our Facebook page to enter our Trivia Contest! You will be entered to win a $15 gift card of your choice from Starbucks, Amazon or iTunes (iTunes is US only)!
If you do not have Facebook, you can enter right here in our forum. Just answer the following questions:
(Note: All participants will be entered into our random drawing regardless of correct or incorrect answers. There will be two winners drawn at random.)
Question 1
The prices of five bonds, with maturities from six months to 2.5 years, that all pay 4.0% semi-annual coupons, allow us to infer the spot rate curve:
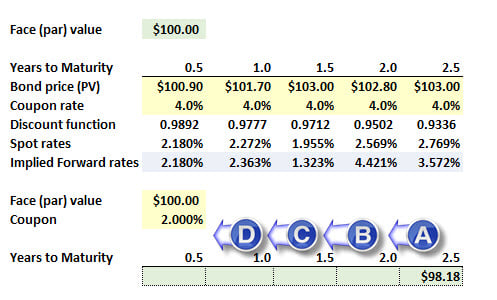
A 2.5-year 2.0% semi-annual coupon bond has a price of $98.18. Assume the spot rate curve is static (unchanged). As the bond approaches maturity, when does its price decrease?
a. From 2.5 to 2.0 years
b. From 2.0 to 1.5 years
c. From 1.5 to 1.0 years
d. From 1.0 to 0.5 years
Question 2
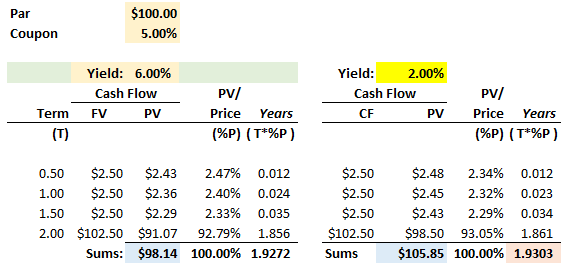
Consider a 5.0% semi-annual coupon bond with a price of $98.14 based on a yield (YTM) of 6.0%:
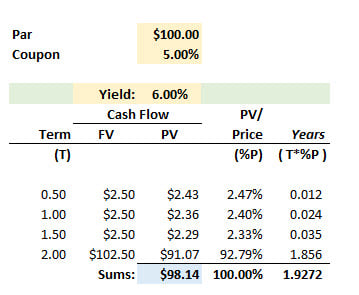
What happens to the bond's duration if the yield decreases to 2.0%?
a. Decreases
b. Unchanged
c. Increases
d. Unclear
Question 3
Consider a 6.0% semi-annual coupon bond with a price of $94.62 based on a yield (YTM) of 6.0%:
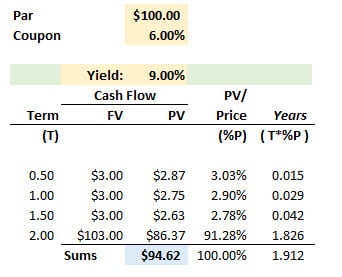
Which is nearest to the bond's DV01?
a. $0.0173
b. $0.0181
c. $0.0240
d. $0.0329
Question 4
Consider a zero-coupon bond with a yield of 5.0% at various maturities?
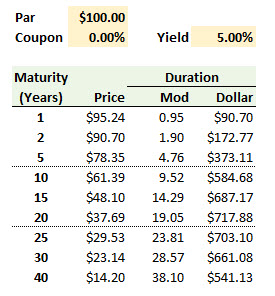
At which displayed maturity is the bond's DV01 the highest? (bonus: under continuous compounding, find the formula for maturity with peak DV01.)
a. One (1) year
b. Ten (10) years
c. Twenty (20) years
d. Forty (40) years
Question 5
Consider a 5.0% annual coupon bond with a price of $84.44 based on a yield (YTM) of 9.0%:
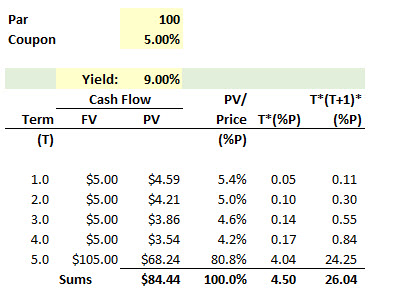
Which is nearest to the bond's convexity?
a. 4.5 years^2
b. 17.3 years^2
c. 21.9 years^2
d. 26.0 years^2
If you do not have Facebook, you can enter right here in our forum. Just answer the following questions:
(Note: All participants will be entered into our random drawing regardless of correct or incorrect answers. There will be two winners drawn at random.)
Question 1
The prices of five bonds, with maturities from six months to 2.5 years, that all pay 4.0% semi-annual coupons, allow us to infer the spot rate curve:

A 2.5-year 2.0% semi-annual coupon bond has a price of $98.18. Assume the spot rate curve is static (unchanged). As the bond approaches maturity, when does its price decrease?
a. From 2.5 to 2.0 years
b. From 2.0 to 1.5 years
c. From 1.5 to 1.0 years
d. From 1.0 to 0.5 years
Question 2
Consider a 5.0% semi-annual coupon bond with a price of $98.14 based on a yield (YTM) of 6.0%:

What happens to the bond's duration if the yield decreases to 2.0%?
a. Decreases
b. Unchanged
c. Increases
d. Unclear
Question 3
Consider a 6.0% semi-annual coupon bond with a price of $94.62 based on a yield (YTM) of 6.0%:

Which is nearest to the bond's DV01?
a. $0.0173
b. $0.0181
c. $0.0240
d. $0.0329
Question 4
Consider a zero-coupon bond with a yield of 5.0% at various maturities?

At which displayed maturity is the bond's DV01 the highest? (bonus: under continuous compounding, find the formula for maturity with peak DV01.)
a. One (1) year
b. Ten (10) years
c. Twenty (20) years
d. Forty (40) years
Question 5
Consider a 5.0% annual coupon bond with a price of $84.44 based on a yield (YTM) of 9.0%:

Which is nearest to the bond's convexity?
a. 4.5 years^2
b. 17.3 years^2
c. 21.9 years^2
d. 26.0 years^2


 You are totally correct, it is the derivative with respect to time (not maturity)! We are looking for the maximum DV01, so we want the d[DV01]/dT. But your expression is even better perfect (because DV01 is a just a scaled dollar duration, by constant 1/10,000, and your statement is exactly true: "We would probably have to take the derivative of $Dur [i.e., dollar duration] with respect to time to get the local maximum. The answer is worked out here, but try it yourself before peeking!
You are totally correct, it is the derivative with respect to time (not maturity)! We are looking for the maximum DV01, so we want the d[DV01]/dT. But your expression is even better perfect (because DV01 is a just a scaled dollar duration, by constant 1/10,000, and your statement is exactly true: "We would probably have to take the derivative of $Dur [i.e., dollar duration] with respect to time to get the local maximum. The answer is worked out here, but try it yourself before peeking!