Upper and lower bounds on options

[wrongsaidfred]

Hi David,

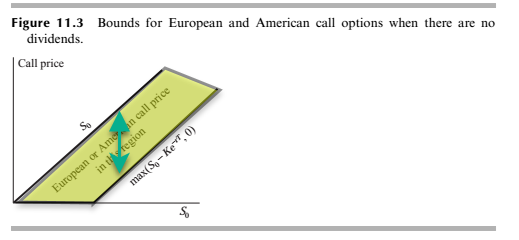
According to Hull, dividends play a role in determining the lower bounds of options prices, but that does not appear in your video. If a question like this arises and the stock provides dividends, should we go by what Hull says?

I also have notes from a class I took that says dividends play a role in the upper bounds of option prices.

It states c (or C) < S-D and p<X*e^-rt+D and P<X+D.

Are these valid or necessary to use on the exam?

Thanks,
Mike

 

[David Harper CFA FRM]

Hi Mike,

Maybe they should be in my video (dividend's impact is assigned: "Discuss the effects dividends have on the put‐call parity, the bounds of put and call option prices, and on the early exercise feature of American options."). Actually, I wish i did have the dividends impact in put-call parity, that really should be in the videos.

It is good to know. Again, "necessary" is impossible to answer given GARP's approach (many AIMs will never be asked). But I regret not addressing this AIM more specifically as this could be tested

I've got:

lower bounds (Hull 9.5 & 9.6)
European c >= S - D - K*exp(-rT)
European p>= D + K*exp(-rT) - S

Put call parity: c + D + K *exp(-rT) = p + S (Hull 9.7)

(
Hull 9.8 is very low testability and not worth the trouble, IMO:
American style with dividends put call parity:
S - D - K <= C- P < S - K *exp(-rT)
)

Thanks, David

 

[wrongsaidfred]

That looks about right.

Can I scrap the upper bound effects since I do not see them in Hull anywhere?

I know that there is no way for you to know what is "necessary". All I meant is that it is an extra step that may or may not be necessary in order to get the correct answer.

Thanks again,
Mike

 

[David Harper CFA FRM]

Hi Mike,

Oh, right, sorry, you have upper bounds. Yes, I agree that upper bounds, with dividends, aren't useful (they have no direct reference in the assigned Hull).

Re: as an extra step: I think what is "in bounds" is the a dividend-paying stock. While low probability exam-wise with respect to lower bound, it has appeared in put call parity (pretty sure that was a question last year or the year before) and possibly with BSM (pricing).

You may note that in all of this cases you can pretty much use this rule: dividends tend to reduce the stock price. So, if you replace (S) with (S-D):

works for lower bound Euro call: replace S - K * exp(-rT) with (S-D) - K*exp(-rT) --> c >= (S-D - K*exp(-rT)
works for lower bound Euro put: replace K * exp(-rT) - S with K*exp(-rT) - (S-D) --> p >= K*exp(-rT) - S +D
put call parity: replace c + K*exp(-rT) = p + S with c + K*exp(-rT) = p + (S - D) --> c + D + K*exp(-rT) = p + D

I hope that helps, David

 

[bpdulog]

Hi,

We are not provided with lower bounds on American options in the study notes so I'd like to know what they are?

 

[David Harper CFA FRM]

Hi @bpdulog See below (from John Hull's OFOD), outlined in red. I agree that I should include this in the next revision. Thanks!

 

[luccacerf]

Hi @David Harper CFA FRM the upper bound of european put is p<=Xe(-rt) not p<=X. I've noticed this trick error on your notes because there is a question on frm pratice exame (P1.82) that requires this knowledge.

 

[gprisby]

Thought I would add to this thread, as I don't see anything on American lower bounds. Question 180.5 also quizzes the American lower bound and I applied the Euro calculation and got it wrong. Let me provide some screenshots to detail my confusion.


Notes I have don't provide lower bound on American options. I also couldn't readily find it in the GARP text book I have...



Question:

180.5. What is the lower bound for the price of a nine (9)-month American PUT option on a non-dividend-paying stock when the stock price is $14.00, the strike price is $20.00, and the risk-free interest rate is 4.0% per annum?

a) zero (0)
b) $5.22
c) $5.41
d) $6.00


Answer.... I discounted the strike and got it wrong!

180.5. D. $6.00
For a European put, the lower bound is p >= K*exp(-rT) - S(0), but
For an American put, the lower bound is P >= K - S(0). In this case, P >= 20 - 14 = $6.00

 

[Flashback]

The meaning of variable X is also not defined.

X is usually denoted as Option Strike (an Exercise price). In FRM curriculum, K variable is used for same meaning.

 

[gprisby]

So, if the lower bound of an American put is: P >= K - S(0)

Does this mean the lower bound of an American call is: C >= S(0) - K ?

 

[Flashback]

I think that The lower bound of not dividend stock AO Call is C >= (S0 - X exp (-r x t).

 

[gprisby]

Hi @Flashback That's where my confusion lies! So if you look at the practice problem I posted above, the American put does not discount K. So, wouldn't that imply the American call does not discount K as well? Just can't find it in the GARP books or my BT notes to confirm one way or another. @David Harper CFA FRM am I going crazy or do I need more coffee?

 

[Flashback]

I fully agree that is a bit confusing but some reasons must be under such approach. I would like that David or someone else to explain such equation clearly.

 

[David Harper CFA FRM]

Hi @gprisby and @Flashback I can see that we can do a better job in the notes explaining this (Hull does explain it, I think, but it's maybe not focused on the explicit comparison between call versus put of the American lower bound). A way to think about the European lower bond is via put call parity, c + K*exp(-rT) = S(0) + p, which I like to recall as "call + discounted cash = protective put." The strike price is cash. Then for European lower bound:

• c = S(0) + p - K*exp(-rT); but as p > 0, the call's value must be at least as great as (setting p = 0 for its lower bound): c > S(0) + 0 - K*exp(-rT) --> c > S(0) - K*exp(-rT); i.e., stock price minus discounted strike
• p = c + K*(-rT) - S(0); but as c > 0, the put's value must be at least as great as (setting c = 0 for its lower bound): p > 0 + K*(-rT) - S --> p > K(-rT) - S(0). The Euro lower bounds do resemble each other. Note: of course, I'm omitting the max(.) function, it's really max(0, ...).

With respect to the American options, the apparent inconsistency is simply due to the fact that K > K*exp(-rT). This is not merely mathematical but speaks to Hull's conclusions about respective optimality of early exercise, K is cash: if you are going to spend a fixed amount of cash (per the call), ceteris paribus you would prefer to defer the spend; if you are going to receive a fixed amount of cash (which is the situation for the put!), you would prefer to receive immediately.

• For the call, c > S(0) - K*exp(-rT); but K > K*exp(-rT) such that [S(0) - K*exp(-rT)] is greater than [S(0) - K] for any r>0, T>0 such that it does not "replace" the lower bound. Hence, C = c > S(0) - K*exp(-rT). And this is also why Hull says, the American call is not worth exercising early: why pay the fixed strike, when you can defer it?!
• But for the put, p > K(-rT) - S(0); and K > K*exp(-rT) such that [K*exp(-rT) - S(0)] is less than [K-S(0)] for any r>0, T>0 such that it does replace the lower bound. Hence, while Euro p > S(0) - K*exp(-rT), American P > S(0) - K. And this is also why Hull says the American call may be worth an early exercise. I hope that's helpful (will make sure this gets into the next revision)!

 

[Flashback]

Great! I will backup this. Thanks!

 

[gprisby]

Cool, thanks David. This makes it more clear in my head. Especially the part about deferring the cash flow when we are paying and wanting it immediately when on the receiving end!

 

[San955]

Hi, can someone explain me the bounds ? Why the upper bound is below the lower bound on the graph?

 

[David Harper CFA FRM]

HI @San955 I think maybe you are misreading Hull's Figure. See below. Yellow is the option value region. At any given stock price, S(0), we can imagine a vertical line slicing through the region. The upper bound is the higher S(0), while the lower bound is the lower max[S0 - K*exp(-rt), 0]. For example, if S0 = $30, then the upper bound on a call option is $30.00 (hardly informative per se: an option cannot be worth more than the underlying stock!). The lower bound, if we assume at-the-money, 2- year term and 3.0% Rf, is max[30 - 30*exp(-3%*2), 0] = $1.74. The way i remember that, btw, is: if $30 grows at Rf, then FV of option will be S*exp(rT) - K; then if we discount that to PV, the PV is PV[FV] = PV[S*exp(rT) - K] = [S*exp(rT) - K]*exp(-rT) = S - K*expr(-rT); i.e., the min value is the PV if the stock grows at the Rf rate. I hope that's helpful,

 

[San955]

Hi @David Harper CFA FRM thank you for your answer! I found out that I should not read the vertical lines from left to right, because at first sight it seems confusing why upper bound is on the left and the lower bound is on the right. Could you give some logic explanation? Also, could I add up one more question why American call option is not optimal to be exercised if interest rates are zero (Hull solution is not much understandable?)

 

[David Harper CFA FRM]

Hi @San955 Sorry, I don't follow you re the bounds. I drew the green arrow to emphasize a vertical perspective: if you pick a stock price, that is S(0) on the X-axis, then look at the vertical line, the upper bound S(0) is higher than the lower bound. Per the math.

In regard to, why American call option is not optimal to be exercised if interest rates are zero? I'm tired (very long day) so maybe I'm mistaken, but this is not true. Please see https://forum.bionicturtle.com/threads/lower-bounds-on-dividend-paying-options.10667/post-52014 It is desirable to early exercise if the PV of dividend is greater than the value of waiting given by K-K*exp(-rT); if the Rf = 0, then the value of waiting is zero; the strike price is the same tomorrow/later as today. For any given dividend (which entices the EE), zero Rf makes early exercise more desirable. Thanks,