Tuckman: Model 2: Simulation

[CarlosB]

Hi David. A question from reading reading 40 on P2.T5, page 42. The table regarding Model 2; Simulation, the annual drift is converted into a monthly drift by dividing by 12. However, the annual volatility is not converted into monthly volatility. Page 43 is saying that the annual volatility should be multiplied by SQRT (1/12) to get the monthly volatility. Is this because the dw is already time-scaled?

 

[ShaktiRathore]

Hi
When annual volatility is multiplied by SQRT (1/12) to get the monthly volatility its equivalent to saying that the annual volatility is converted into a monthly volatility by dividing by SQRT(12).So we time scale the annual volatility by SQRT (1/12) to get monthly volatility.
thanks

 

[David Harper CFA FRM]

Hi @CarlosB Yes, dw is random standard normal but it is already time-scaled. If you'd like to see it in action, here is the underlylng XLS (simulations will differ of course): https://www.dropbox.com/s/x77nvpb0tblxrv1/1102-tuckman-model-2.xlsx?dl=0
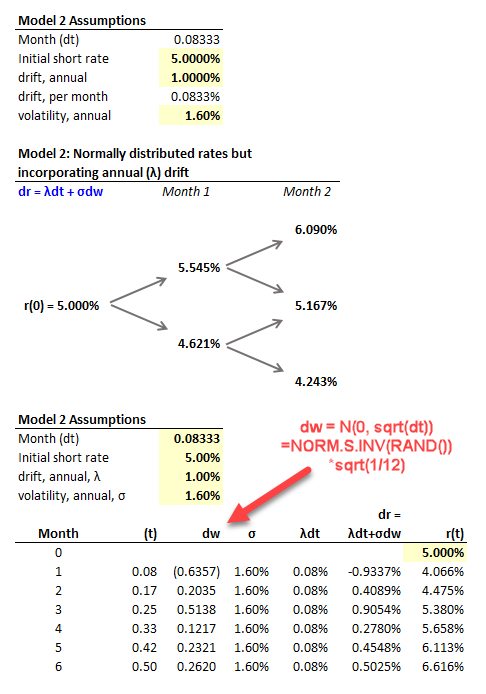
(see snapshot below also)

And from Tuckman (emphasis mine):

"The particularly simple model of this section will be called Model 1. The continuously compounded, instantaneous rate r(t) is assumed to evolve according to the following equation: (9.1)

dr = σ *dw

The quantity dr denotes the change in the rate over a small time interval, dt, measured in years; σ denotes the annual basis-point volatility of rate changes; and dw denotes a normally distributed random variable with a mean of zero and a standard deviation of sqrt(dt)." -- Tuckman, Bruce; Serrat, Angel. Fixed Income Securities: Tools for Today's Markets (Wiley Finance) (p. 251). Wiley. Kindle Edition.