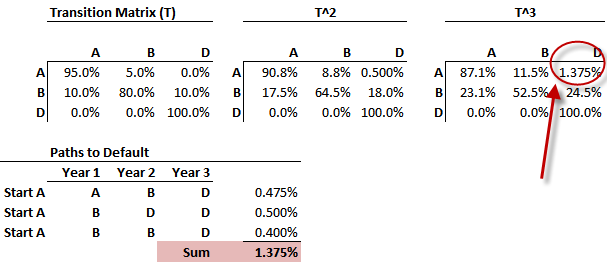
Hi @David Harper CFA FRM , suppose that matrix A is a transition matrix (I.e one with Markov property). Suppose we have calculated two and three year transition matrix by taking the square and cube of matrix A. How do we get the cumulative PD from the example in this link:
https://learn.bionicturtle.com/images/forum/e1.41b.png
I have read through certain papers where they add up the confusing term “marginal pd” which I wish to avoid using to get cumulative pd. But from what I understand, transition matrix is a conditional pd. Why does adding up conditional pd give us a cumulative pd? Adding conditional pd might results in probability exceeding 1.
Thanks!

https://learn.bionicturtle.com/images/forum/e1.41b.png
I have read through certain papers where they add up the confusing term “marginal pd” which I wish to avoid using to get cumulative pd. But from what I understand, transition matrix is a conditional pd. Why does adding up conditional pd give us a cumulative pd? Adding conditional pd might results in probability exceeding 1.
Thanks!
