Theta of ITM Put option

[sm@23]

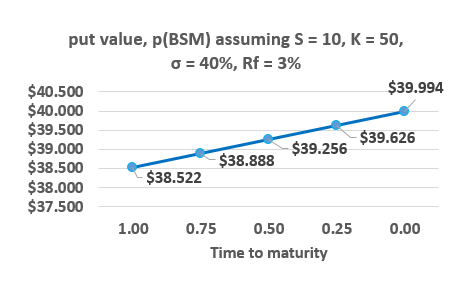
Hi @David Harper CFA FRM I found in your earlier post that intuition for theta to be +ve for ITM Put option as below:
"if you hold a deep ITM put, with asset price near zero, more "waiting time" to the Euro expiration is not a good thing, asset price can't go below zero. Volatility has asymmetric effect: mostly works against you to increase asset price/decrease put. You want to expire as soon as possible"

I'm still not getting why put value will increase i.e theta is +ve as we approach option expiry here?

Is there any illustration you can refer please ?

 

[David Harper CFA FRM]

Hi @sm@23, I can try with a quick illustration, but admittedly I think this is a counterintuive idea because, as you suggest, this is an exception to the general rule that an option will experience "time decay;" i.e., its value will decrease as maturity approaches. To generate the exception, all I need to do is price the put option when it is deeply in-the-money. So, in this example, the prices are genuine Black-Scholes-Merton (here is the XLS at https://www.dropbox.com/s/ppu83yebn6a7tq2/0821-theta-put.xlsx?dl=0) and the assumptions are:

• S = 10, K = 50 so this is deeply in the money
• Volatility, σ = 40%; although somewhat (also) counterintutively this is an exceptional case where volatility doesn't much matter; put another way, vega is nearly zero for deeply in- or out-of-money options
• Rf = 3.0%
• The Term varies: {1.0 year, 0.75 years, 0.50 years, 0.25 and 1/250 is the last value which represents only one day until maturity}

Note that, per this exception, put option value is actually increasing as maturity decreases. Mathematically, we can explain this: these values are effectively the minimum option values, given by K*exp(-rT) - S0. For example, with a one year maturity, the put value is effectively the minimum value of $50.00*exp(-3%*1.0) - $10.00 = $38.522. In this way, notice the value is really just increasing because as a function of the discounted strike price!

At the same time, the intuition that you cite, the way that I look at this is: Imagine you hold the one-year European put option when S = 10.00 and K = 50.00. This is a volatile stock (40%). The intrinsic value is $40.00. If it were American style, don't you want to exercise now and collect the $40.00? Compare this to the scenario where the stock is steady at $10.00 for the entire year: true, you will get the same $40.00, but it will be one year later, so your PV is lower. On the other hand, volatility represents the possibility of "random diffusion" in either direction. Unlike the call option (which has theoretically unlimited upside), this put can never be exercised for more than $50.00 because the stock can never fall below zero. Don't you want to exercise now also because the volatility has "more time to reduce your intrinsic value" than it "has room" to increase your value (it only has + 10 more that is even possible). I hope that's helpful!

 

[sm@23]

Thanks a ton @David Harper CFA FRM

 

[WhizzKidd]

this put can never be exercised for more than $50.00 because the stock can never fall below zero. Don't you want to exercise now also because the volatility has "more time to reduce your intrinsic value" than it "has room" to increase your value (it only has + 10 more that is even possible)?

Hi @David Harper CFA FRM, from the above statement I don't see why Theta would be positive? Since time and vol could push the put option out the money. Is the reason here for why a Deep ITM put will have positive Theta is that as time passes, volatility would be nearly negligible and that as it gets closer to maturity it becomes more likely to be exercised?

Side Q: Have you made a spreadsheet on how the greeks move for calls and puts? Like something, one can change and see the effects either graphically or numerically. I am piggybacking here as you spreadsheets are really easy to use

 

[David Harper CFA FRM]

HI @WhizzKidd to check for the associated spreadsheet, you can always try the SP. We did recently publish an updated version for R27 which includes a fully updated set of Greek option calculations and exhibits, which took many many hours but I'm happy with it, please see https://learn.bionicturtle.com/topic/hull-chapters-13-15-19/

Re: your question, I'm not sure how to further elaborate on my illustration and discussion above. But please note we are talking about an already deeply in the money put. You are absolutely correct that "time and vol could push the put option out the money." In fact, contrary to say an ATM call option or ATM put option where you'd prefer more time and greater volatility in order to find yourself in the money, in this case where you are already deeply in the money, more time and greater volatility are working against you! You would prefer to exercise immediately, but it's European and you must wait. Positive theta, which is unusual, means that the option value is increasing with shorter time to expiration (i.e., as time goes forward naturally); it's positive in this case of deep ITM put because you are getting close to the point where you can exercise for the gain and, in a manner of speaking, "there is less and less time for volatility to work against you and jolt this thing into out of the money." I hope that's helpful!