Table 5.2: Key Rate DV01s and Durations of 30-year C-STRIP

[ankit4685]

Hi,
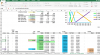
In the given table, can you help me to understand the logic of calculation we have used to derive Shock and D(t)
I'm not very much sure what is generic forumula / theory used to calcualte the =I30-($G$30/($A$36-$A$30)) [ Snippet 1 ]
And while calcualting D(t) why we used "2" ---> =(2-J28*SUM($K$27:K27))/(2+J28) [ Snippet 2 ]



[ Snippet 2 ]

 

[ankit4685]

Hi @David Harper CFA FRM
can you share your view on this

 

[David Harper CFA FRM]

HI @ankit4685

The first calculation (the shock value in green) is a simple linear interpolation between the "peak" shift of 0.010% (i.e., one basis point) at 2.0 years (i.e., the 4th period) and zero basis points at 10 years (the 5th period). The shocks are graphed in the upper right corner of your image; the graph plots these shock values. The formula, in your highlighted case, is just subtracting the fraction of one basis point equal to the (number of periods from the 4th period)/(six periods between 4 and 10) so that you have a line dropping by 1/6th a basis point each period until you get to the 10th period.

The second formula is solving for the discount factor as a function of the current par yield and the (prior) discount function (i.e., discount factors for the term structure up to the current point). This requires a full understanding of the par yield, which (unlike the spot/zero rate) is necessarily a function of prior discount factors. Please see my videos below for help as needed with the par yield. Then starting with the definition of the par yield, I solved for the current discount factor, d(T):

• C(T)/2*A(T) + d(T) = 1.0, where A(T) is the annuity factor; i..e, the sum of discount factors. We need to strip out d(T), where A(T) = d(T) + A(T-1):
• C(T)/2*[A(T-1) + d(T)] + d(T) = 1.0, and
• C(T)/2*A(T-1) + C(T)/2*d(T) + d(T) = 1.0, and
• C(T)/2*A(T-1) + d(T)*[C(T)/2 + 1.0] = 1.0, and
• d(T) = [ 1.0 - C(T)/2*A(T-1) ] / [C(T)/2 + 1.0], so that d(T) is given by:
• d(T) = [ 2.0 - C(T)*A(T-1) / [C(T) + 2.0]. I hope that's helpful!

Par yields are swap rates

Fixed Income: Infer discount factors, spot, forwards and par rates from swap rate curve (FRM T4-25)