gargi.adhikari
Active Member
In reference to R19.P1.T3.FIN_PRODS_HULL_Ch6_Topic: Euro$ FuturesContracts_Convexity_Adjustments
Hi,
I am trying to understand why the Futures Rate is = ( 100 - the Euro$ Futures Price) = (100-95) = 5 % ...?
Why is : Euro$ Futures- Quote= ( 100 - The 3-month Euro$ Futures- Interest Rate)
=> Q% = (100% - R%) ...?
Did I miss this caveat /knowledge point in any of the chapters...? :-( :-(
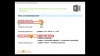
Hi,
I am trying to understand why the Futures Rate is = ( 100 - the Euro$ Futures Price) = (100-95) = 5 % ...?
Why is : Euro$ Futures- Quote= ( 100 - The 3-month Euro$ Futures- Interest Rate)
=> Q% = (100% - R%) ...?
Did I miss this caveat /knowledge point in any of the chapters...? :-( :-(

Last edited: