gargi.adhikari
Active Member
In reference to R19.P1.T3.FIN_PRODS_HULL_Ch26_ExoticOptions_Topic: UP-and-OUT Barrier-Options:-
Are UP-and-OUT Barrier-Options = PUT Options ? or can they be CALL Options too ..?
If they are CALL Options, why would one want to limit the Payoff and Profit by putting a cap on the upward movement of the price..? Is it to lower the premium of the option ?
Much gratitude for the insight on this.
Are UP-and-OUT Barrier-Options = PUT Options ? or can they be CALL Options too ..?
If they are CALL Options, why would one want to limit the Payoff and Profit by putting a cap on the upward movement of the price..? Is it to lower the premium of the option ?
Much gratitude for the insight on this.


 at this stuff !!!!!!!!!!!!!!!!!!!
at this stuff !!!!!!!!!!!!!!!!!!! 

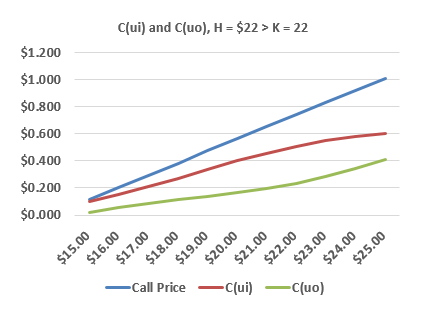
 Imagine that I am your counterparty and I will write you an one-year barrier option when the stock price is $100.00. The strike price is also $100.00, but we agree the barrier, H = $108.00. If this a knock-out (aka, up and out) barrier option, then it will cease to exist if the stock price breaches $108.00. You would prefer to test less frequently and I would prefer to test more frequently: the more frequently we observe whether S > H, then the more likely this option will be knocked-out. In extremis, we'd only test once at the end of the year; in this case, it might breach several times in the meantime, but still not get knocked out. Maybe we test monthly, but it could still breach on many days and survive. Daily observations, by comparison, decrease the value of this option to you (and the price I can charge you for it). Hence, for the knock-out barrier, an increase in frequency implies a decrease in value.
Imagine that I am your counterparty and I will write you an one-year barrier option when the stock price is $100.00. The strike price is also $100.00, but we agree the barrier, H = $108.00. If this a knock-out (aka, up and out) barrier option, then it will cease to exist if the stock price breaches $108.00. You would prefer to test less frequently and I would prefer to test more frequently: the more frequently we observe whether S > H, then the more likely this option will be knocked-out. In extremis, we'd only test once at the end of the year; in this case, it might breach several times in the meantime, but still not get knocked out. Maybe we test monthly, but it could still breach on many days and survive. Daily observations, by comparison, decrease the value of this option to you (and the price I can charge you for it). Hence, for the knock-out barrier, an increase in frequency implies a decrease in value.