AIMs: Explain how Credit VaR of a portfolio is calculated using the single-factor model, and how correlation affects the distribution of loss severity for intermediate values between 0 and 1. Describe how Credit VaR can be calculated using a simulation of joint defaults with a copula.
Questions:
311.1. Employing a single-factor model for the Credit VaR of a portfolio, Malz relates the market factor return (m) to a stated level of portfolio loss, in this case 0.010:

(Source: Allan Malz, Financial Risk Management: Models, History, and Institutions (Hoboken, NJ: John Wiley & Sons, 2011))
If the default probability is 1.0%, such that k = N[-1](1.0%) = -2.33, and the correlation is 0.64, such that beta = 0.80, which is nearest to the probability that the the portfolio loss is 0.01 or worse; i.e., the probability that the market factor ends up at a quantile, or worse, associated with a portfolio loss of 0.01?
a. 1.75%
b. 5.83%
c. 12.24%
d. 29.00%
311.2. Hull also employs the same single-factor Credit VaR model, in this case X = 99.9% to signify a desired confidence level that happens to match the confidence level built-into Basel's internal ratings-based (IRB) Credit VaR formula:
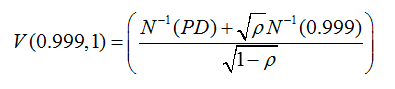
(Source: John C. Hull, Options, Futures, and Other Derivatives, 8th Edition (PrenticeHallPub; (2011))
This returns a worst-case default rate given an average default probability, PD, and copula correlation parameter, rho. The formula is essentially similar to Malz: the minus becomes a plus only because a positive deviate at 99.9% is used rather than the negative deviate at 0.10%; and as Malz discusses, correlation equals beta^squared.
If a bank holds a highly granular $100.0 million portfolio of retail exposures with average one-year default probability of 2.0% an average recovery rate of 50.0%, where the copula correlation parameter is estimated as 0.130, which is nearest is the 99.9% one-year credit VaR, per Malz definition which is an unexpected loss?
a. $4.030 million
b. $6.845 million
c. $9.720 million
d. $17.630 million
311.3. In regard to using copulas to estimate portfolio credit risk, Malz writes that "copulas are a very attractive modeling technique, since they permit the model to generate quite detailed results—the entire probability distribution of portfolio credit outcomes—with a very light theoretical apparatus and requiring the estimation of only one additional parameter, the correlation, beyond those used in single-credit modeling." (Source: Allan Malz, Financial Risk Management: Models, History, and Institutions (Hoboken, NJ: John Wiley & Sons, 2011))
However, he cites EACH of the following as a PITFALL (drawback) except for which is not?
a. The marginal distributions must be normal such that we are forced to accept a multivariate normal of defaults
b. The choice of copula is arbitrary and that we simply do not know enough to reliably estimate the copula correlation
c. It is difficult enough to estimate default correlations, and the copula correlation is only related to, not identical to it
d. Once a copula parameter value is assigned, it is tempting to rely on a wide range of consequently generated model results, but this is dangerous
Answers:
Questions:
311.1. Employing a single-factor model for the Credit VaR of a portfolio, Malz relates the market factor return (m) to a stated level of portfolio loss, in this case 0.010:

(Source: Allan Malz, Financial Risk Management: Models, History, and Institutions (Hoboken, NJ: John Wiley & Sons, 2011))
If the default probability is 1.0%, such that k = N[-1](1.0%) = -2.33, and the correlation is 0.64, such that beta = 0.80, which is nearest to the probability that the the portfolio loss is 0.01 or worse; i.e., the probability that the market factor ends up at a quantile, or worse, associated with a portfolio loss of 0.01?
a. 1.75%
b. 5.83%
c. 12.24%
d. 29.00%
311.2. Hull also employs the same single-factor Credit VaR model, in this case X = 99.9% to signify a desired confidence level that happens to match the confidence level built-into Basel's internal ratings-based (IRB) Credit VaR formula:
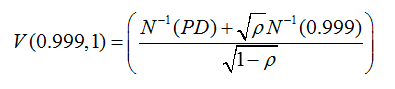
(Source: John C. Hull, Options, Futures, and Other Derivatives, 8th Edition (PrenticeHallPub; (2011))
This returns a worst-case default rate given an average default probability, PD, and copula correlation parameter, rho. The formula is essentially similar to Malz: the minus becomes a plus only because a positive deviate at 99.9% is used rather than the negative deviate at 0.10%; and as Malz discusses, correlation equals beta^squared.
If a bank holds a highly granular $100.0 million portfolio of retail exposures with average one-year default probability of 2.0% an average recovery rate of 50.0%, where the copula correlation parameter is estimated as 0.130, which is nearest is the 99.9% one-year credit VaR, per Malz definition which is an unexpected loss?
a. $4.030 million
b. $6.845 million
c. $9.720 million
d. $17.630 million
311.3. In regard to using copulas to estimate portfolio credit risk, Malz writes that "copulas are a very attractive modeling technique, since they permit the model to generate quite detailed results—the entire probability distribution of portfolio credit outcomes—with a very light theoretical apparatus and requiring the estimation of only one additional parameter, the correlation, beyond those used in single-credit modeling." (Source: Allan Malz, Financial Risk Management: Models, History, and Institutions (Hoboken, NJ: John Wiley & Sons, 2011))
However, he cites EACH of the following as a PITFALL (drawback) except for which is not?
a. The marginal distributions must be normal such that we are forced to accept a multivariate normal of defaults
b. The choice of copula is arbitrary and that we simply do not know enough to reliably estimate the copula correlation
c. It is difficult enough to estimate default correlations, and the copula correlation is only related to, not identical to it
d. Once a copula parameter value is assigned, it is tempting to rely on a wide range of consequently generated model results, but this is dangerous
Answers:

