Fran
Administrator
AIMs: Describe common frictions that arise during the creation of credit contracts. Define the following terms related to default and recovery: default events, probability of default, credit exposure, and loss given default. Calculate expected loss from recovery rates, the loss given default, and the probability of default.
Questions:
301.1. Each of the following describes a common friction that can arise during a credit contract EXCEPT which is incorrectly or imprecisely defined?
a. Asymmetric information: the borrower knows more than the lender about the the project to which the loan proceeds will be applied
b. Principal-agent: an investment manager is more concerned about fees and trading income than his investor's returns
c. Moral hazard: insurance reduces the incentive of the insured to avoid the insurable event
d. Externalities: equity investors (and managers with stock options) are motivated to increase the risk of the firm's assets, but debt holders do not benefit from this
301.2. The following is Malz’s definition of conditional default probability (Malz 7.2.4):
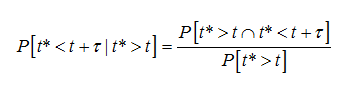
(Source: Allan Malz, Financial Risk Management: Models, History, and Institutions (Hoboken, NJ: John Wiley & Sons, 2011))
Each of the following is true about this formula's parameters and/or variables EXCEPT for which is incorrect? (bonus: which is the random variable?)
a. Small (t) is the time from which we are viewing default, it could be now or a future date
b. Greek tau (τ) is the time interval over which the default probability is measured
c. Small t with asterisk (t*) is when the default occurs
d. P[t* > t] refers to the probability of default before time (t); i.e., the unconditional default probability between (0,t)
301.3. Malz employs the following single-period relationship in order to summarize the minimum coupon spread (z) that should be expected by an investor as compensation for an expected loss:
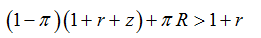
(Source: Allan Malz, Financial Risk Management: Models, History, and Institutions (Hoboken, NJ: John Wiley & Sons, 2011))
If the exposure is $1.0 million, the riskfree rate is 2.0%, the default probability (PD) is 3.0%, and the loss given default (LGD) is 60.0%, which is nearest to the implied minimum spread (z) required to compensate an investor (lender) for the expected loss?
a. 38 basis points
b. 84 basis points
c. 192 basis points
d. 235 basis points
Answers:
Questions:
301.1. Each of the following describes a common friction that can arise during a credit contract EXCEPT which is incorrectly or imprecisely defined?
a. Asymmetric information: the borrower knows more than the lender about the the project to which the loan proceeds will be applied
b. Principal-agent: an investment manager is more concerned about fees and trading income than his investor's returns
c. Moral hazard: insurance reduces the incentive of the insured to avoid the insurable event
d. Externalities: equity investors (and managers with stock options) are motivated to increase the risk of the firm's assets, but debt holders do not benefit from this
301.2. The following is Malz’s definition of conditional default probability (Malz 7.2.4):
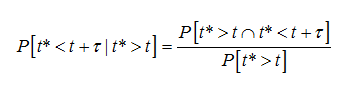
(Source: Allan Malz, Financial Risk Management: Models, History, and Institutions (Hoboken, NJ: John Wiley & Sons, 2011))
Each of the following is true about this formula's parameters and/or variables EXCEPT for which is incorrect? (bonus: which is the random variable?)
a. Small (t) is the time from which we are viewing default, it could be now or a future date
b. Greek tau (τ) is the time interval over which the default probability is measured
c. Small t with asterisk (t*) is when the default occurs
d. P[t* > t] refers to the probability of default before time (t); i.e., the unconditional default probability between (0,t)
301.3. Malz employs the following single-period relationship in order to summarize the minimum coupon spread (z) that should be expected by an investor as compensation for an expected loss:
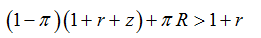
(Source: Allan Malz, Financial Risk Management: Models, History, and Institutions (Hoboken, NJ: John Wiley & Sons, 2011))
If the exposure is $1.0 million, the riskfree rate is 2.0%, the default probability (PD) is 3.0%, and the loss given default (LGD) is 60.0%, which is nearest to the implied minimum spread (z) required to compensate an investor (lender) for the expected loss?
a. 38 basis points
b. 84 basis points
c. 192 basis points
d. 235 basis points
Answers:
