Learning objectives: Describe a ratings transition matrix and explain its uses. Describe the process for and issues with building, calibrating, and backtesting an internal rating system. Identify and describe the biases that may affect a rating system.
919.1. In order to develop an internal rating system, Stronghouse Financial International is considering employing the Internal Rating Template below (which is based on de Servigny's Figure 2-8):

As Stronghouse evaluates the use of this template, it will find that each of the following statements is true EXCEPT which statement is inaccurate?
a. The choice of weights is very important, and these weights should be calibrated on a large sample and backtested often
b. The score can be mapped to an external credit rating (e.g., AAA) so that transition matrices can be used for portfolio management and to feed their economic or regulatory capital measures
c. The primary advantage of this go-forward internal template is that the resultant internal ratings can be linked to default probabilities without any need for a historical sample
d. If Stronghouse uses at-the-point (PIT) tools as the backbone to this internal rating template, the primary risk is providing highly unstable transition matrices and no guidance for the long term
919.2. Finlux International seeks to build an internal rating system for its considerable credit portfolio and assigns the project to a team including Alice, Bob, Chris, and Denise. In order to cast a wide net for ideas, each of the team members builds a mini-prototype:
I. Alice (A) developed an internal migration matrix based on a sample taken during a recession such that (related) her probabilities are not Markovian, yet to retrieve 5-year cumulative default probabilities she raises the matrix to the fifth power (ie., 5-year cumulative matrix = M^5) a calculation that might be valid if her probabilities were Markovian (scale bias)
II. Bob (B), in order to maximum the universe of rated bonds, combines ratings for all of the major agencies across industries, countries, asset classes (homogeneity bias)
III Chris (C) uses a method of polling the salespeople who originate the loans on the theory that these are the people with the best "on the ground" knowledge of credit risk (principal-agent bias)
IV. Denise (D) mixed at-the-point-in-time and through-the-cycle ratings because she was unaware of which methodological assumption applied to each sourced dataset
Which of the following accurately matches each team member to the bias that afflicts their approach?
a. Alice (A) = Scale bias; Bob (B) = Homogeneity bias; Chris (C) = Principal-Agent bias; Denise (D) = Time horizon bias
b. Alice (A) = Principal agent bias; Bob (B) = Scale bias; Chris (C) = Time horizon bias; Denise (D) = Homogeneity bias
c. Alice (A) = Criteria bias; Bob (B) = Principal agent bias; Chris (C) = Scale bias; Denise (D) = Bias arising from lack of backtesting
d. Alice (A) = Bias arising from lack of backtesting; Bob (B) = Information bias; Chris (C) = Criteria bias; Denise (D) = Principal agent bias
919.3. Consider the following one-year migration (aka, transition) matrix:
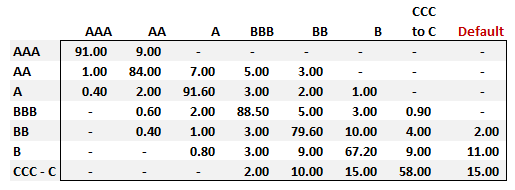
When is the soonest that an obligation rated AAA (the highest rating) can default?
a. During the first year (i.e., by the end of the first year) with a margin probability of 2.0%
b. During the second year (i.e., between the end of the first and second year) with a joint probability of 0.1879% (or 0.001879 or 1.879e-3)
c. During the third year (i.e., between the end of the second and third year) with an unconditional probability of 0.00540% (or 0.0000540 or 5.40e-5)
d. During the fourth year (i.e., between the end of the third and fourth year) with a conditional probability of 2.00%
Answers here:
919.1. In order to develop an internal rating system, Stronghouse Financial International is considering employing the Internal Rating Template below (which is based on de Servigny's Figure 2-8):

As Stronghouse evaluates the use of this template, it will find that each of the following statements is true EXCEPT which statement is inaccurate?
a. The choice of weights is very important, and these weights should be calibrated on a large sample and backtested often
b. The score can be mapped to an external credit rating (e.g., AAA) so that transition matrices can be used for portfolio management and to feed their economic or regulatory capital measures
c. The primary advantage of this go-forward internal template is that the resultant internal ratings can be linked to default probabilities without any need for a historical sample
d. If Stronghouse uses at-the-point (PIT) tools as the backbone to this internal rating template, the primary risk is providing highly unstable transition matrices and no guidance for the long term
919.2. Finlux International seeks to build an internal rating system for its considerable credit portfolio and assigns the project to a team including Alice, Bob, Chris, and Denise. In order to cast a wide net for ideas, each of the team members builds a mini-prototype:
I. Alice (A) developed an internal migration matrix based on a sample taken during a recession such that (related) her probabilities are not Markovian, yet to retrieve 5-year cumulative default probabilities she raises the matrix to the fifth power (ie., 5-year cumulative matrix = M^5) a calculation that might be valid if her probabilities were Markovian (scale bias)
II. Bob (B), in order to maximum the universe of rated bonds, combines ratings for all of the major agencies across industries, countries, asset classes (homogeneity bias)
III Chris (C) uses a method of polling the salespeople who originate the loans on the theory that these are the people with the best "on the ground" knowledge of credit risk (principal-agent bias)
IV. Denise (D) mixed at-the-point-in-time and through-the-cycle ratings because she was unaware of which methodological assumption applied to each sourced dataset
Which of the following accurately matches each team member to the bias that afflicts their approach?
a. Alice (A) = Scale bias; Bob (B) = Homogeneity bias; Chris (C) = Principal-Agent bias; Denise (D) = Time horizon bias
b. Alice (A) = Principal agent bias; Bob (B) = Scale bias; Chris (C) = Time horizon bias; Denise (D) = Homogeneity bias
c. Alice (A) = Criteria bias; Bob (B) = Principal agent bias; Chris (C) = Scale bias; Denise (D) = Bias arising from lack of backtesting
d. Alice (A) = Bias arising from lack of backtesting; Bob (B) = Information bias; Chris (C) = Criteria bias; Denise (D) = Principal agent bias
919.3. Consider the following one-year migration (aka, transition) matrix:

When is the soonest that an obligation rated AAA (the highest rating) can default?
a. During the first year (i.e., by the end of the first year) with a margin probability of 2.0%
b. During the second year (i.e., between the end of the first and second year) with a joint probability of 0.1879% (or 0.001879 or 1.879e-3)
c. During the third year (i.e., between the end of the second and third year) with an unconditional probability of 0.00540% (or 0.0000540 or 5.40e-5)
d. During the fourth year (i.e., between the end of the third and fourth year) with a conditional probability of 2.00%
Answers here:
Last edited by a moderator:
