Learning objectives: Define, calculate, and interpret key rate ’01 and key rate duration. Describe the key rate exposure technique in multi-factor hedging applications; summarize its advantages and disadvantages. Calculate the key rate exposures for a given security, and compute the appropriate hedging positions given a specific key rate exposure profile.
Questions:
912.1. The table below (which is modeled after Tuckman's Table 5.2) illustrates the key-rate DV01's for $1,000.00 face amount of a 30-year zero coupon bond. Just as with Tuckman's example, the key interest rate is a par yield:
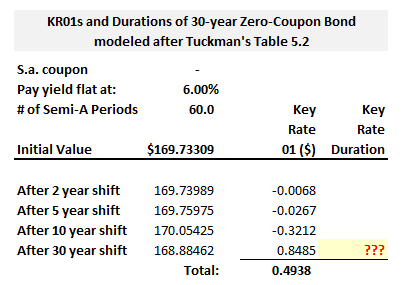
Source: Bruce Tuckman, Fixed Income Securities, 3rd Edition (Hoboken, NJ: John Wiley & Sons, 2011)
Which of the following is nearest the 30-year key rate duration (denoted "???" above)?
a. -18.9 years
b. +18.9 years
c. +28.5 years
d. +50.0 years
912.2. Assume we follow Tuckman's illustration and estimate the key rate exposures (i.e., KR01s and key rate durations) for a zero-coupon bond with 30 years to maturity; e.g., C-STRIP. The key interest rate is a par yield. The analysis selects four key rates: 2-year, 5-year, 10-year and 30-year. Further, as Tuckman explains about the rule for rates between neighbors, "each of the four shapes is called a key-rate shift. Each key rate affects par yields from the term of the previous key rate (or zero) to the term of the next key rate (or the last term). For example, the 10-year key rate affects par yields of terms 5 to 30 years only. Furthermore, the impact of each key rate is normalized to be one basis point at its own maturity and then assumed to decline linearly, reaching zero at the terms of the adjacent key rates. For the two-year shift at terms of less than 2 years and for the 30-year shift at terms greater than 30 years, however, the assumed change is constant at one basis point." Source: Bruce Tuckman, Fixed Income Securities, 3rd Edition (Hoboken, NJ: John Wiley & Sons, 2011)
About this key rate exposure technique, each of the following statements is true EXCEPT which is false?
a. It is possible for a 30-year zero coupon bond to have a non-zero key-rate with respect to the 10-year shift
b. Due to the linear decline between neighbors, we need to double (i.e., multiply by 2) the sum of the four KR01s to approximate the DV01 of the security
c. The shift in the 10-year par yield (as the key rate) implies a shift of greater than one basis point in the 10-year spot rate and a negative shift in the 30-year spot rate
d. If the key-rate '01 with respect to the 5-year shift is -0.0035, KR01(5.0) = -0.0035, then the security's price increases by $0.0035 per $100 face value amount for a positive one-basis point shift in the five-year key rate
912.3. Below is replicated Tuckman's Table 5.3 and Table 5.4. The situation concerns a Trader with four positions (aka, Initial Position):
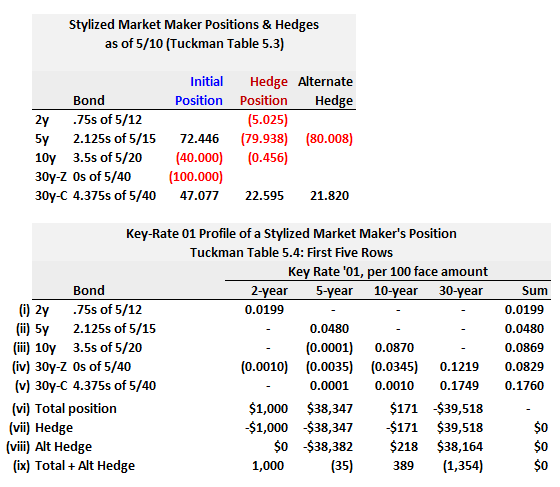
Source: Bruce Tuckman, Fixed Income Securities, 3rd Edition (Hoboken, NJ: John Wiley & Sons, 2011)
Which of the following statements is TRUE about this situation?
a. The Initial Position has a flat key-rate profile
b. The Initial Position has significant DV01 exposure
c. The Hedge Position is a portfolio that contains four bonds
d. The Initial Position is a substantial 5s-30s flattener: the Trader profits if 5-year yields increase more than 30-year yields
Answers here:
Questions:
912.1. The table below (which is modeled after Tuckman's Table 5.2) illustrates the key-rate DV01's for $1,000.00 face amount of a 30-year zero coupon bond. Just as with Tuckman's example, the key interest rate is a par yield:

Source: Bruce Tuckman, Fixed Income Securities, 3rd Edition (Hoboken, NJ: John Wiley & Sons, 2011)
Which of the following is nearest the 30-year key rate duration (denoted "???" above)?
a. -18.9 years
b. +18.9 years
c. +28.5 years
d. +50.0 years
912.2. Assume we follow Tuckman's illustration and estimate the key rate exposures (i.e., KR01s and key rate durations) for a zero-coupon bond with 30 years to maturity; e.g., C-STRIP. The key interest rate is a par yield. The analysis selects four key rates: 2-year, 5-year, 10-year and 30-year. Further, as Tuckman explains about the rule for rates between neighbors, "each of the four shapes is called a key-rate shift. Each key rate affects par yields from the term of the previous key rate (or zero) to the term of the next key rate (or the last term). For example, the 10-year key rate affects par yields of terms 5 to 30 years only. Furthermore, the impact of each key rate is normalized to be one basis point at its own maturity and then assumed to decline linearly, reaching zero at the terms of the adjacent key rates. For the two-year shift at terms of less than 2 years and for the 30-year shift at terms greater than 30 years, however, the assumed change is constant at one basis point." Source: Bruce Tuckman, Fixed Income Securities, 3rd Edition (Hoboken, NJ: John Wiley & Sons, 2011)
About this key rate exposure technique, each of the following statements is true EXCEPT which is false?
a. It is possible for a 30-year zero coupon bond to have a non-zero key-rate with respect to the 10-year shift
b. Due to the linear decline between neighbors, we need to double (i.e., multiply by 2) the sum of the four KR01s to approximate the DV01 of the security
c. The shift in the 10-year par yield (as the key rate) implies a shift of greater than one basis point in the 10-year spot rate and a negative shift in the 30-year spot rate
d. If the key-rate '01 with respect to the 5-year shift is -0.0035, KR01(5.0) = -0.0035, then the security's price increases by $0.0035 per $100 face value amount for a positive one-basis point shift in the five-year key rate
912.3. Below is replicated Tuckman's Table 5.3 and Table 5.4. The situation concerns a Trader with four positions (aka, Initial Position):
- The Trader shorted $100.0 million face amount of a 30-year STRIPS to a customer, buying about $47.0 million face of the 30-year bond to hedge the resulting interest rate risk. Additionally,
- The Trader facilitated a customer 5s-10s curve trade by shorting $40.0 million face of the 10-year note and buying about $72.0 million of the 5-year note

Source: Bruce Tuckman, Fixed Income Securities, 3rd Edition (Hoboken, NJ: John Wiley & Sons, 2011)
Which of the following statements is TRUE about this situation?
a. The Initial Position has a flat key-rate profile
b. The Initial Position has significant DV01 exposure
c. The Hedge Position is a portfolio that contains four bonds
d. The Initial Position is a substantial 5s-30s flattener: the Trader profits if 5-year yields increase more than 30-year yields
Answers here:
