Learning objectives: Compute the value of a European option using the Black-Scholes-Merton model on a non-dividend-paying stock. Compute the value of a warrant and identify the complications involving the valuation of warrants. Define implied volatilities and describe how to compute implied volatilities from market prices of options using the Black-Scholes-Merton model.
Questions:
815.1. Sally owns a non-dividend-paying stock that is currently trading at $60.00. As a hedge, she buys an out-of-the-money three-month European put option on the stock with a fixed strike price of $57.00, which is a 5.0% discount to the current stock price. The stock's volatility is 36.0% per annum. The riskfree rate is 4.0%. According to the Black-Scholes-Merton model, what is the value of the put? (here is a Z lookup table snippet that you can use http://learn.bionicturtle.com/images/2018/forum/082718-t4-815-1-zlookup.jpg).
a. $1.55
b. $2.63
c. $3.89
d. $4.40
815.2. A company’s stock price is $100.00 and 50.0 million shares are outstanding, so that its equity market capitalization is $5.0 billion. The company is considering granting 2.0 million at-the-money employee stock options (ESOs) because it has been advised that a grant equal to 4.0% of the outstanding is competitive. The ESOs have a 10 year maturity, and for analytical convenience we assume there are no vesting restrictions (an unrealistic assumption). Option exercises will be handled by issuing more shares. The stock price volatility is 26.0% per annum. The 10-year risk-free rate is 4.0%. The company has no plans to pay dividends. Which is nearest to the total cost of the warrant issue?
a. $31.2 million
b. $44.3 million
c. $88.5 million
d. $109.7 million
815.3. Mark the Risk Analyst is evaluating an at-the-money (ATM) call option on a non-dividend-paying stock when the stock's price is $30.00; i.e., S(0) = K = $30.00. For this option, he has the table shown below. The table displays option prices generated by the Black-Scholes-Merton option pricing model according to selected input variations of volatility and maturity. In other words, the table displays different values of c = BSM[S(0) = $30.00, K = $30.00, σ, Rf = 0.030, T].
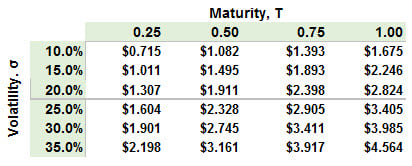
Mark's option actually expires in in eight months; T = 8/12. Further, he observes that the option's traded price (aka, observed market price) is $3.00. In this case, which of the following is nearest to the call option's implied volatility?
a. 24.5%
b. 28.0%
c. 31.5%
d. 35.0%
Answers:
Questions:
815.1. Sally owns a non-dividend-paying stock that is currently trading at $60.00. As a hedge, she buys an out-of-the-money three-month European put option on the stock with a fixed strike price of $57.00, which is a 5.0% discount to the current stock price. The stock's volatility is 36.0% per annum. The riskfree rate is 4.0%. According to the Black-Scholes-Merton model, what is the value of the put? (here is a Z lookup table snippet that you can use http://learn.bionicturtle.com/images/2018/forum/082718-t4-815-1-zlookup.jpg).
a. $1.55
b. $2.63
c. $3.89
d. $4.40
815.2. A company’s stock price is $100.00 and 50.0 million shares are outstanding, so that its equity market capitalization is $5.0 billion. The company is considering granting 2.0 million at-the-money employee stock options (ESOs) because it has been advised that a grant equal to 4.0% of the outstanding is competitive. The ESOs have a 10 year maturity, and for analytical convenience we assume there are no vesting restrictions (an unrealistic assumption). Option exercises will be handled by issuing more shares. The stock price volatility is 26.0% per annum. The 10-year risk-free rate is 4.0%. The company has no plans to pay dividends. Which is nearest to the total cost of the warrant issue?
a. $31.2 million
b. $44.3 million
c. $88.5 million
d. $109.7 million
815.3. Mark the Risk Analyst is evaluating an at-the-money (ATM) call option on a non-dividend-paying stock when the stock's price is $30.00; i.e., S(0) = K = $30.00. For this option, he has the table shown below. The table displays option prices generated by the Black-Scholes-Merton option pricing model according to selected input variations of volatility and maturity. In other words, the table displays different values of c = BSM[S(0) = $30.00, K = $30.00, σ, Rf = 0.030, T].

Mark's option actually expires in in eight months; T = 8/12. Further, he observes that the option's traded price (aka, observed market price) is $3.00. In this case, which of the following is nearest to the call option's implied volatility?
a. 24.5%
b. 28.0%
c. 31.5%
d. 35.0%
Answers:
