Learning objectives: Calculate the change in a bond’s price given its duration, its convexity, and a change in interest rates. Compare and contrast the major theories of the term structure of interest rates
Questions:
715.1. Consider the following continuously compounded zero (spot) rate curve and their associated discount factors; e.g., df(1.5) = exp(-0.0540*1.5) = 0.9222.
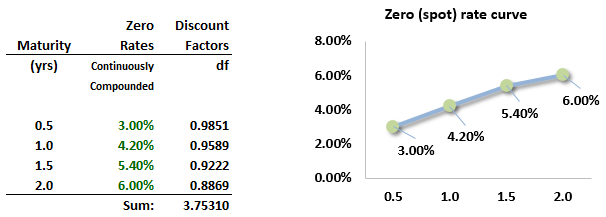
What is nearest to the per annum par yield, if we follow Hull and express the par yield with continuous compounding while the coupon is paid semi-annually? Hint: per Hull, the par yield, denoted by (c), must satisfy 1.0 = A*c/m + 1.0*d, where (d) is the present value of $1.0 received at the maturity of the bond, (A) is the value of an annuity that pays one dollar on each coupon payment date, and (m) is the number of coupon payments per year.
a. 3.7420%
b. 4.0330%
c. 5.1890%
d. 6.0270%
715.2. A 30-year zero-coupon bond with a face value of $100.00 has a yield of 5.0% per annum with continuous compounding. Peter the analyst wants to estimate the impact of a 35 basis point increase ("shock up") in the yield without fully re-pricing the bond. Using duration, he estimates the bond price will drop by 10.50% or $2.34287. This was easy to compute as the bond has a duration of 30.0 years and 30.0 * 0.00350 = 10.50%. However, this calculation forgets to includes a convexity term. Which is nearest to the additional impact of the convexity term only?
a. To reduce the estimated bond price drop by $0.1230 so that the total estimated drop is only $2.2199
b. To reduce the estimated bond price decrease by $0.2460 so that the total estimated drop is only $2.0969
c. To increase the estimated bond price decrease by $0.1674 so that the total estimated drop is $2.5102
d. To increase the estimated bond price decrease by $0.5690 so that the total estimated drop is $2.9118
715.3. Consider the following upward-sloping but smooth zero rate curve:
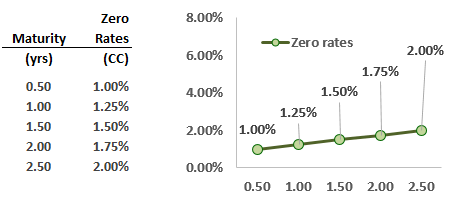
There are various theories that attempt to explain the factors that determine the shape of the zero rate curve. If the above zero curve is observed, each of the following theories is plausible EXCEPT which of the following theories is the LEAST LIKELY to be true, if only because it does not comport with the observed zero curve?
a. Pure expectations, the expected future six-month spot rate, E[S(2.0, 2.5)], is 3.00%
b. Under liquidity preference, the expected future six-month spot rate, E[S(2.0, 2.5)], is 3.00%
c. Under liquidity preference, the expected future six-month spot rate, E[S(2.0, 2.5)], is 2.40%
d. Under preferred habitat (market segmentation), the expected future six-month spot rate, E[S(2.0, 2.5)], could be 3.15%
Answers here:
Questions:
715.1. Consider the following continuously compounded zero (spot) rate curve and their associated discount factors; e.g., df(1.5) = exp(-0.0540*1.5) = 0.9222.

What is nearest to the per annum par yield, if we follow Hull and express the par yield with continuous compounding while the coupon is paid semi-annually? Hint: per Hull, the par yield, denoted by (c), must satisfy 1.0 = A*c/m + 1.0*d, where (d) is the present value of $1.0 received at the maturity of the bond, (A) is the value of an annuity that pays one dollar on each coupon payment date, and (m) is the number of coupon payments per year.
a. 3.7420%
b. 4.0330%
c. 5.1890%
d. 6.0270%
715.2. A 30-year zero-coupon bond with a face value of $100.00 has a yield of 5.0% per annum with continuous compounding. Peter the analyst wants to estimate the impact of a 35 basis point increase ("shock up") in the yield without fully re-pricing the bond. Using duration, he estimates the bond price will drop by 10.50% or $2.34287. This was easy to compute as the bond has a duration of 30.0 years and 30.0 * 0.00350 = 10.50%. However, this calculation forgets to includes a convexity term. Which is nearest to the additional impact of the convexity term only?
a. To reduce the estimated bond price drop by $0.1230 so that the total estimated drop is only $2.2199
b. To reduce the estimated bond price decrease by $0.2460 so that the total estimated drop is only $2.0969
c. To increase the estimated bond price decrease by $0.1674 so that the total estimated drop is $2.5102
d. To increase the estimated bond price decrease by $0.5690 so that the total estimated drop is $2.9118
715.3. Consider the following upward-sloping but smooth zero rate curve:

There are various theories that attempt to explain the factors that determine the shape of the zero rate curve. If the above zero curve is observed, each of the following theories is plausible EXCEPT which of the following theories is the LEAST LIKELY to be true, if only because it does not comport with the observed zero curve?
a. Pure expectations, the expected future six-month spot rate, E[S(2.0, 2.5)], is 3.00%
b. Under liquidity preference, the expected future six-month spot rate, E[S(2.0, 2.5)], is 3.00%
c. Under liquidity preference, the expected future six-month spot rate, E[S(2.0, 2.5)], is 2.40%
d. Under preferred habitat (market segmentation), the expected future six-month spot rate, E[S(2.0, 2.5)], could be 3.15%
Answers here:
