gargi.adhikari
Active Member
In Reference to R15.P1.T2.DIEBOLD_CH8_Topic: AR(p) Properties-COVARIANT-STATIONARY :-
Wanted to clarify if the AR(p) Property of Covariance Stationarity should include the conditions that the Mean and the variance be Stable/Constant ..?
The Inverse of the Roots of the Lag Operator is a Mandatory condition - so that has to be met for the Process to be Covar Stationary. But that does not not guarantee Covar Stationarity- so does it mean in addition, the Mean and the Variance has to be stable and Constant for the AR(p) process to be Covar Stationary..?

Very grateful for any insights on this.
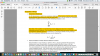
Wanted to clarify if the AR(p) Property of Covariance Stationarity should include the conditions that the Mean and the variance be Stable/Constant ..?
The Inverse of the Roots of the Lag Operator is a Mandatory condition - so that has to be met for the Process to be Covar Stationary. But that does not not guarantee Covar Stationarity- so does it mean in addition, the Mean and the Variance has to be stable and Constant for the AR(p) process to be Covar Stationary..?


Very grateful for any insights on this.


