Learning objectives: Describe and distinguish between continuous and discrete random variables. Define and distinguish between the probability density function, the cumulative distribution function, and the inverse cumulative distribution function. Calculate the probability of an event given a discrete probability function.
Questions:
708.1. Let f(x) represent a probability function (which is called a probability mass function, p.m.f., for discrete random variables and a probability density function, p.d.f., for continuous variables) and let F(x) represent the corresponding cumulative distribution function (CDF); in the case of the continuous variable, F(X) is the integral (aka, anti-derivative) of the pdf. Each of the following is true about these probability functions EXCEPT which is false?
a. The limits of a cumulative distribution function (CDF) must be zero and one; i.e., F(-∞) = 0 and F(+∞) = 1.0
b. For both discrete and random variables, the cumulative distribution function (CDF) is necessarily an increasing function
c. In the case of a continuous random variable, we cannot talk about the probability of a specific value occurring; e.g., Pr[R = +3.00%] is meaningless
d. Bayes Theorem can only be applied to discrete random variables, such that continuous random variables must be transformed into their discrete equivalents
708.2. Consider a binomial distribution with a probability of each success, p = 0.050, and that total number of trials, n = 30 trials. What is the inverse cumulative distribution function associated with a probability of 25.0%?
a. Zero successes
b. One successes
c. Two successes
d. Three successes
708.3. For a certain operational process, the frequency of major loss events during a one period year varies form zero to 5.0 and is characterized by the following discrete probability mass function (pmf) which is the exhaustive probability distribution and where (b) is a constant:
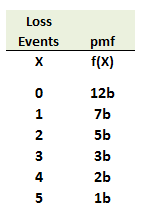
Which is nearest to the probability that next year LESS THAN two major loss events will happen?
a. 5.3%
b. 22.6%
c. 63.3%
d. 75.0%
Answers here:
Questions:
708.1. Let f(x) represent a probability function (which is called a probability mass function, p.m.f., for discrete random variables and a probability density function, p.d.f., for continuous variables) and let F(x) represent the corresponding cumulative distribution function (CDF); in the case of the continuous variable, F(X) is the integral (aka, anti-derivative) of the pdf. Each of the following is true about these probability functions EXCEPT which is false?
a. The limits of a cumulative distribution function (CDF) must be zero and one; i.e., F(-∞) = 0 and F(+∞) = 1.0
b. For both discrete and random variables, the cumulative distribution function (CDF) is necessarily an increasing function
c. In the case of a continuous random variable, we cannot talk about the probability of a specific value occurring; e.g., Pr[R = +3.00%] is meaningless
d. Bayes Theorem can only be applied to discrete random variables, such that continuous random variables must be transformed into their discrete equivalents
708.2. Consider a binomial distribution with a probability of each success, p = 0.050, and that total number of trials, n = 30 trials. What is the inverse cumulative distribution function associated with a probability of 25.0%?
a. Zero successes
b. One successes
c. Two successes
d. Three successes
708.3. For a certain operational process, the frequency of major loss events during a one period year varies form zero to 5.0 and is characterized by the following discrete probability mass function (pmf) which is the exhaustive probability distribution and where (b) is a constant:

Which is nearest to the probability that next year LESS THAN two major loss events will happen?
a. 5.3%
b. 22.6%
c. 63.3%
d. 75.0%
Answers here:
Last edited by a moderator:
