Learning objectives: Explain the differences between a probability mass function and a probability density function. Characterize the quantile function and quantile-based estimators. Explain the effect of a linear transformation of a random variable on the mean, variance, standard deviation, skewness, kurtosis, median and interquartile range.
Questions:
20.4.1. Consider the probability mass function (pmf) below. For example, Pr(X = -1.0) = 20.0%.
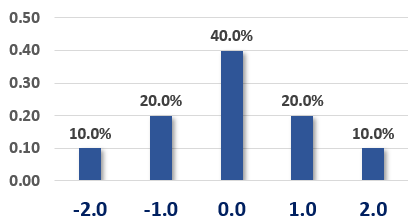
As we can see, this distribution is symmetrical so we know that its skewness is zero without performing any calculations. We are told the variance is 1.20 (although we can calculate the variance). What is this distribution's kurtosis?
a. Zero
b. 2.50
c. 3.60
d. 4.40
20.4.2. Peter decides that the payoff of his option straddle strategy can be approximated by the probability density function (pdf) illustrated below. The function is: f(x) = a*x^2 over the real domain {-3 < X < +3); i.e., {f(x) = a*x^2 | x ∈ -3.0 < X < 3.0}. He used x-squared because he wants the shape of a parabola, but how does he determine the value of the constant, a? It cannot be anything! Because this is a probability distribution, it must be true that the integral, a*x^3/3, when evaluated over [3, -3] equals one: the area under the pdf curve must equal one, and the integral of the pdf is the cumulative distribution function (CDF).
Given that a*3^3/3 - a*(-3)^3/3 = 1.0, we must have 9a - (-9a) = 1.0 such that a = 1/18. Therefore, a is 1/18 and his probability density function is f(x) = x^2/18. But is x^3/54 the integral? He notices that if F(x) = x^3/54 then F(3) = 27/54 = 0.5 and F(-3) = -0.5, but the CDF requires that F(3) = 1.0 and F(-3.0) = zero. Seeing this, he realizes that the indefinite integral includes a constant and is given by a*x^3 + c, or specifically, x^3/54 + c. Now it is possible for 3^3/54 + c = 1.0, if c = 1.0 - 0.5 = 0.5. Finally, he can specify the exact pdf, f(x) = x^2/18, as the derivative of the correct CDF, F(x) = x^3/54 + 0.5, so that F(3) = 1.0 and F(-3) = 0.
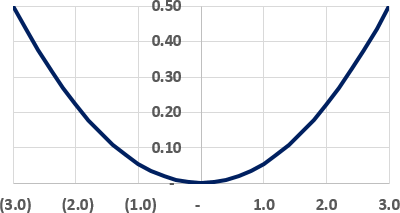
Value-at-risk (VaR) is the distributional quantile associated with a specified probability. The inverse CDF (aka, quantile function) returns the quantile implied by a probability, q = F^(-1)(p), whereas the CDF returns the probability associated with a quantile: p = F(q), or in this case, p = F(X). In this distribution, losses are on the left (negative payoffs), so the 95.0% VaR is the quantile implied by a 5.0% probability: X = F^(-1)(p) = F^(-1)(0.050). For this distribution, what is the 95.0% VaR, in this case, the 0.050 quantile?
a. -2.90
b. -2.70
c. -1.50
d. +0.33
20.4.3. Let Z be a random variable that is a linear function of random variables X and Y, where Z = 3*X + 7*Y? If the standard deviation of X and Y, respectively, are σ(X) = 4.0 and σ(Y) = 5.0 and the correlation between X and Y is ρ(X,Y) = 0.50, then what is the standard deviation of Z, σ(Z)?
a. 6.40
b. 7.81
c. 37.00
d. 42.30
Answers here:
Questions:
20.4.1. Consider the probability mass function (pmf) below. For example, Pr(X = -1.0) = 20.0%.

As we can see, this distribution is symmetrical so we know that its skewness is zero without performing any calculations. We are told the variance is 1.20 (although we can calculate the variance). What is this distribution's kurtosis?
a. Zero
b. 2.50
c. 3.60
d. 4.40
20.4.2. Peter decides that the payoff of his option straddle strategy can be approximated by the probability density function (pdf) illustrated below. The function is: f(x) = a*x^2 over the real domain {-3 < X < +3); i.e., {f(x) = a*x^2 | x ∈ -3.0 < X < 3.0}. He used x-squared because he wants the shape of a parabola, but how does he determine the value of the constant, a? It cannot be anything! Because this is a probability distribution, it must be true that the integral, a*x^3/3, when evaluated over [3, -3] equals one: the area under the pdf curve must equal one, and the integral of the pdf is the cumulative distribution function (CDF).
Given that a*3^3/3 - a*(-3)^3/3 = 1.0, we must have 9a - (-9a) = 1.0 such that a = 1/18. Therefore, a is 1/18 and his probability density function is f(x) = x^2/18. But is x^3/54 the integral? He notices that if F(x) = x^3/54 then F(3) = 27/54 = 0.5 and F(-3) = -0.5, but the CDF requires that F(3) = 1.0 and F(-3.0) = zero. Seeing this, he realizes that the indefinite integral includes a constant and is given by a*x^3 + c, or specifically, x^3/54 + c. Now it is possible for 3^3/54 + c = 1.0, if c = 1.0 - 0.5 = 0.5. Finally, he can specify the exact pdf, f(x) = x^2/18, as the derivative of the correct CDF, F(x) = x^3/54 + 0.5, so that F(3) = 1.0 and F(-3) = 0.

Value-at-risk (VaR) is the distributional quantile associated with a specified probability. The inverse CDF (aka, quantile function) returns the quantile implied by a probability, q = F^(-1)(p), whereas the CDF returns the probability associated with a quantile: p = F(q), or in this case, p = F(X). In this distribution, losses are on the left (negative payoffs), so the 95.0% VaR is the quantile implied by a 5.0% probability: X = F^(-1)(p) = F^(-1)(0.050). For this distribution, what is the 95.0% VaR, in this case, the 0.050 quantile?
a. -2.90
b. -2.70
c. -1.50
d. +0.33
20.4.3. Let Z be a random variable that is a linear function of random variables X and Y, where Z = 3*X + 7*Y? If the standard deviation of X and Y, respectively, are σ(X) = 4.0 and σ(Y) = 5.0 and the correlation between X and Y is ρ(X,Y) = 0.50, then what is the standard deviation of Z, σ(Z)?
a. 6.40
b. 7.81
c. 37.00
d. 42.30
Answers here:
