@David Harper CFA FRM
Hi,
Firstly I am posting this here because whenever I click on the links to threads in the study notes, I get the message "Oops, you do not have permission to access this thread. ". I am unsure what to do about that.
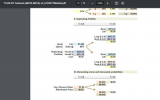
Anyways, my question is in Page 25 of the study notes Tuckman chapter-7 in market risk management. You mentioned the below -
"We already inferred the risk-neutral probabilities in the move from date 0 to date 1: the risk-neutral probability of an up move on date 0 is 80.24%; the risk-neutral probability of a down move on date 0 is 19.76%."
However this risk neutral probability is calculated for the previous example where the market value at time 0 is 950.423. In this example since there are three 6 month periods, as opposed to 2 in the previous example, we calculate the market value at time 0 to be 925.21. How are we using the same risk neutral probabilities as before since we calculate those probabilities by equating the market value to the value obtained from the time 1 values.
My second query is on how to intuitively think about a call option on a bond being replicated by a long position in 1 year bonds, and short position in 6 month bond. How are the cash flows same?
Thanks, and sorry for posting it here. (Kindly help me with these issues)
Hi,
Firstly I am posting this here because whenever I click on the links to threads in the study notes, I get the message "Oops, you do not have permission to access this thread. ". I am unsure what to do about that.
Anyways, my question is in Page 25 of the study notes Tuckman chapter-7 in market risk management. You mentioned the below -
"We already inferred the risk-neutral probabilities in the move from date 0 to date 1: the risk-neutral probability of an up move on date 0 is 80.24%; the risk-neutral probability of a down move on date 0 is 19.76%."
However this risk neutral probability is calculated for the previous example where the market value at time 0 is 950.423. In this example since there are three 6 month periods, as opposed to 2 in the previous example, we calculate the market value at time 0 to be 925.21. How are we using the same risk neutral probabilities as before since we calculate those probabilities by equating the market value to the value obtained from the time 1 values.
My second query is on how to intuitively think about a call option on a bond being replicated by a long position in 1 year bonds, and short position in 6 month bond. How are the cash flows same?
Thanks, and sorry for posting it here. (Kindly help me with these issues)