hull chapter 7 swaps questions clarification
- Thread starter puneet_
- Start date
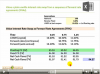
The forward rates are converted into semi-annual compounded rates, so that the interest amount can be calculated by the simple formula
CF = ForwardRate * Notional * DeltaT
Please note, that semi-annual compounding is chosen, because than the pay-frequency and compounding frequency are identical, this is also called simple interest rate.
Of course strictly speaking the conversion is not necessary, since the interest can be calculated directly from the cont. compounded rate by:
CF = Notional * ( exp(ForwardRate * DeltaT) - 1 )
But the Author decided to do this calculation in two steps instead, I guess for reasons of clarity.
Did that answer your question?
CF = ForwardRate * Notional * DeltaT
Please note, that semi-annual compounding is chosen, because than the pay-frequency and compounding frequency are identical, this is also called simple interest rate.
Of course strictly speaking the conversion is not necessary, since the interest can be calculated directly from the cont. compounded rate by:
CF = Notional * ( exp(ForwardRate * DeltaT) - 1 )
But the Author decided to do this calculation in two steps instead, I guess for reasons of clarity.
Did that answer your question?
Additionally to my remarks above, I think I found an error in the example.
If you look at the forward rates, than you see that they are calculated from the Libor rates with the formula for continuous compounding e.g.:
10.75 = (0.75 * 10.5 - 0.25 * 10) / 0.5
But the libor rates are not continuous compounded, they are given as simple interest. So the libor rates and forward rates shown are not compatible.
I see no reason to use continuous compounding at all in the calculation, but of course I judge solely from the screenshot. The rest of the video might offer explanation for that.
If you look at the forward rates, than you see that they are calculated from the Libor rates with the formula for continuous compounding e.g.:
10.75 = (0.75 * 10.5 - 0.25 * 10) / 0.5
But the libor rates are not continuous compounded, they are given as simple interest. So the libor rates and forward rates shown are not compatible.
I see no reason to use continuous compounding at all in the calculation, but of course I judge solely from the screenshot. The rest of the video might offer explanation for that.
@ami44 your first explanation is exactly correct. This is from Hull: his assumptions include LIBOR rates with continuous compounding (it's always the case with Hull that spot/zero rates are given with continuous compounding), but the swap's pay fixed rate is given with semi-annual compounding (i.e., to match the payment frequency). So, the assumptions do not match, as given, such that the conversion is necessary. Thanks!
Similar threads
