GARP T3 Chapter 16 Question 16.16

[ktrathen]

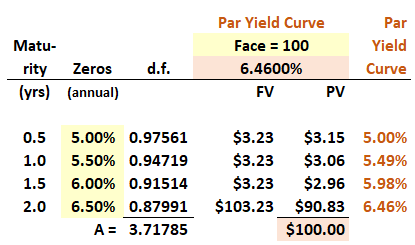
Question 16.16: The six-month, 12-month, 18-month, and 24-month zero rates are 5%, 5.5%, 6%, and 6.5% (all measured with semi-annual compounding) respectively. What is the two-year par yield for a bond paying coupons every six months?


For the par yield, I keep getting 6.41%, and A= 3.7465. Slightly different from the answers in the book.

Is this a mistake, or have I done something incorrect?

 

[David Harper CFA FRM]

Hi @ktrathen I agree with the solution. Par yield is something that is easily validated. Please see below (this XLS is here https://www.dropbox.com/s/76vxbf5nkbg4u4v/081620-sa-par-yield.xlsx?dl=0)

6.46% is correct because that is the per annum coupon rate that prices the bond exactly to par ($100.00). I was thinking it was maybe rounding, but it looks like you should be getting =((100-100*0.8799)*2)/3.7179 = 6.460636. In these situations, easily the most common causes of discrepancy are (i) compound frequency differences and related (ii) rounding. I hope that's helpful,

 

[ktrathen]

Thanks Dave.

I think I must have mistyped something minor on my calculator, as when I repeat the calculation this morning after some sleep, I get 3.7179 instead of something slightly different.

At least, in the process of working it out, I effectively derived the formula (c/m)A + 100d = 100

Is there a BAII Plus shortcut for this calculation?

 

[David Harper CFA FRM]

Sure thing. Re shortcut: no, not to my knowledge. But you'll notice that in this typically upward-sloping zero curve, the par yields are just below the zero rates; e.g., at 2 years, 6.46% versus 6.50%; at 1.5 years, 5.98% versus 6.0% For this reason, on a multiple-choice (exam), it's actually pretty easy to guess the par: it's just below the spot.