Forward rates
- Thread starter puneet_
- Start date
-
- Tags
- forward rates
Hi,
Yes i think it should be mentioned in the question whether the rate is cc or discrete.Its not safe to assume cc unless and otherwise mentioned,please do not assume anything unless and until mentioned in the question.It should be clearly inferred from the question whether cc or discrete compounding is to be used.
thanks
Yes i think it should be mentioned in the question whether the rate is cc or discrete.Its not safe to assume cc unless and otherwise mentioned,please do not assume anything unless and until mentioned in the question.It should be clearly inferred from the question whether cc or discrete compounding is to be used.
thanks
Forward rates are normally compounded in the frequency of the period length of the rate. E.g. a forward rate for the times 01.06.2017 to 01.01.2018 will normally be compounded with 0.5 years. I've also seen that called flat or simple rate or non-compounded.
The consequence is, that you can calculate the interest of a coupon by multiplying the forward rate with the nominal and period length.
Also it is what you get, when you calculate your forward rate from discount factors by
( DF(t-1) / DF(t) - 1 ) * 1/delta t
Me personally I've never seen forward rates in any other form, but of course i can not be sure for 100%
The consequence is, that you can calculate the interest of a coupon by multiplying the forward rate with the nominal and period length.
Also it is what you get, when you calculate your forward rate from discount factors by
( DF(t-1) / DF(t) - 1 ) * 1/delta t
Me personally I've never seen forward rates in any other form, but of course i can not be sure for 100%
Last edited:
The reason I agree with @ShaktiRathore is, with respect to the exam, we've seen continuous, annual and semi-annual. @ami44 I like using discount factors, for sure, as they embed the spot rate compound assumptions, but I don't understand your formula; e.g., if the rates which inform the df(.)s are annual rates, don't you want ( DF(t-1) / DF(t) - 1 )^(1/Δt) -1 ?
fwiw, i have a post here at https://forum.bionicturtle.com/threads/shortcut-to-forward-rates-if-you-have-bond-prices.4927/
I do notice that the 2106 P1 exam contains a forward rate question (#76) below. Notice it assumes annual compounding, but I think this is imprecise; also the explain has a typo, it should be 1.045^5/1.035^3^(1/2)-1 = 6.018%. Although please note, the question correctly says "is closest to" such that, for example, if you assumed continuous compounding such that your result is 6.00% = (5*4.5% - 3*3.5%)/(2-1), you would have no trouble. So i think this question is fair in the sense that it (i) asks for "nearest to" and (ii) gives choices that are not near each other. Still, I am commenting to GARP re: my view that I think the rate compound frequencies should all be specified (if only on the logic that a good question does not need the choices: a good question allows for the user to produce a single exact answer without reference to any choices). I hope that's interesting ...
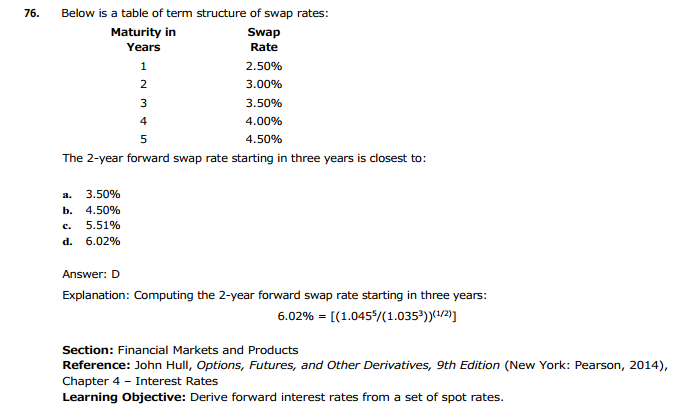
fwiw, i have a post here at https://forum.bionicturtle.com/threads/shortcut-to-forward-rates-if-you-have-bond-prices.4927/
I do notice that the 2106 P1 exam contains a forward rate question (#76) below. Notice it assumes annual compounding, but I think this is imprecise; also the explain has a typo, it should be 1.045^5/1.035^3^(1/2)-1 = 6.018%. Although please note, the question correctly says "is closest to" such that, for example, if you assumed continuous compounding such that your result is 6.00% = (5*4.5% - 3*3.5%)/(2-1), you would have no trouble. So i think this question is fair in the sense that it (i) asks for "nearest to" and (ii) gives choices that are not near each other. Still, I am commenting to GARP re: my view that I think the rate compound frequencies should all be specified (if only on the logic that a good question does not need the choices: a good question allows for the user to produce a single exact answer without reference to any choices). I hope that's interesting ...
Hi David,
now that you mention it, I remember that indeed forward rates with continuous compounding were mentioned somewhere and I studied them for the exam. Totally forgot about that, sorry. So I take back my statement above.
I guess continuous compounding can sometimes make the math easier. But apart from that, is any other compounding frequency really used for forwards, and if why?
Regarding the formula: Discountfactors are always the same, regardless what kind of rates have been used to calculate them. The formula is definately correct, i often use it, but I'm too tired right now for the math, sorry. Maybe tommorrow.
now that you mention it, I remember that indeed forward rates with continuous compounding were mentioned somewhere and I studied them for the exam. Totally forgot about that, sorry. So I take back my statement above.
I guess continuous compounding can sometimes make the math easier. But apart from that, is any other compounding frequency really used for forwards, and if why?
Regarding the formula: Discountfactors are always the same, regardless what kind of rates have been used to calculate them. The formula is definately correct, i often use it, but I'm too tired right now for the math, sorry. Maybe tommorrow.
I'm not sure anybody is still interested, but for closure here the derivation of my above mentioned formula for forward rates.
Consider two counterparties A and B which can both borrow at market rate (e.g. Libor). They enter into an Forward Rate Agreement that says, that A will lend B 1 USD at time t0 and B will pay it back at time t1. Also B will pay A at t1 the current market rate in interest for borrowing from t0 to t1.
The value of this contract is 0 at inception, because borrowing and lending at the market rate can not be worth anything for A and B.
Looking at the discounted cashflows:
0 = -DF(t0) + (1 + f * (t1 - t0)) * DF(t1)
With f being the (annualized) forward rate that makes the value of contract being zero. Also f is here choosen to be a simple interest rate.
Solving for f:
f = (DF(t0) / DF(t1) - 1) / (t1 - t0)
q.e.d.
I see this formula being used a lot in the wild. If I encounter a forward rate I would always assume that it is given as simple interest rate. But of course I can't speak for everybody everywhere.
Consider two counterparties A and B which can both borrow at market rate (e.g. Libor). They enter into an Forward Rate Agreement that says, that A will lend B 1 USD at time t0 and B will pay it back at time t1. Also B will pay A at t1 the current market rate in interest for borrowing from t0 to t1.
The value of this contract is 0 at inception, because borrowing and lending at the market rate can not be worth anything for A and B.
Looking at the discounted cashflows:
0 = -DF(t0) + (1 + f * (t1 - t0)) * DF(t1)
With f being the (annualized) forward rate that makes the value of contract being zero. Also f is here choosen to be a simple interest rate.
Solving for f:
f = (DF(t0) / DF(t1) - 1) / (t1 - t0)
q.e.d.
I see this formula being used a lot in the wild. If I encounter a forward rate I would always assume that it is given as simple interest rate. But of course I can't speak for everybody everywhere.
Last edited:
Similar threads
