Errors Found in Study Materials P1.T4. Valuation & Risk Models (OLD thread)

[Sixcarbs]

Hi David,

I hope this is the right place for this. There is an error in the video on Hull Chapter 15. You say things correctly but the slide you are pointing at is incorrect.

It is in Hull's example 15.3

Slide says:

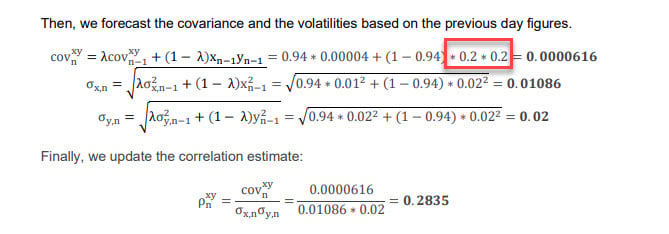
.17-(.2/2) = .15

.2 is the volatility, should be variance (.2)^2. Answer .15 is correct.

.17-[(.2)^2]/2= .15

Then below it slide says:

Standard deviation,
sqrt(.2/3)= 0.1155

Should be .2/sqrt(3)= 0.1155

Also, in Study notes, Hull Chapter 13, Binomial Trees. Page 6.

"The risk-neutral probability (= 0.6523) is found first."

I think it should be .5503, which it is in the spreadsheet below. I looked high and low for a way to come up with .6523 and could not calculate it or find a place for it.

I hope this helps.

Sixcarbs

 

[tattoo]

Hi david.
In page 6 of R27-P1-T4, it says "The risk-neutral probability p= 0.6523". However, according to



and



I came up with the result of p is 0.5503 which is consistent with the result of the spreadsheet showed below.



So where does "p= 0.6523" come from?
In addition, does the symbol "<" in the picture above(< probability of up jump) mean "less than"? If so, why is p less than probability of up jump? Many thanks.

 

[samuelfu9999]

Hi David,

For 818.1 of the Practice Questions of R27 (P.93), the d1 of rate of
change of the option price with respect to the futures price should be ln[F(0)/K] + σ^2*T/2 )/ [σ*sqrt(T)].

For 818.3, it is missing in the pdf.

 

[tattoo]

Hi david, in page 12 of R27-P1-T4, Hull's example 13.11:
the result from my side is like this

The figures in red are different with yours. But I cannot figure out why. Could you have a check? Thanks.

 

[David Harper CFA FRM]

@tattoo (cc: @Nicole Seaman )

1. You and @Sixcarbs are correct, thank you! There is a typo in the R27 Study Note, page 6, in the 3rd paragraph, instead of "(p = 0.6523)" it should read "(p = 0.5503)" to match the exhibit, as follows:

"The risk-neutral probability (p = 0.5503) is found first. The option prices at the final nodes are calculated as payoffs from the option. At the topmost node of time period two (T=0.5), the option value () is 3.2 (stock price of 24.2 minus strike price of 21). In the middle and lowermost nodes, the option is out of the money and so its value is zero (for and )."

2. In your binomial, it looks like you are discounting with exp[-(r-q)]; e.g., your 101.1160 = [(0.5126 * 189.3362) + (0.4874 * 10.000)] * exp[-(5.0% - 2.0%)*0.25] but you want [(0.5126 * 189.3362) + (0.4874 * 10.000)] * exp(-5.0%*0.25) = 100.6614; i.e., this is just a discounting function, you don't subtract the dividend yield here.

@samuelfu9999 Yes, totally agree thank you! (@Nicole Seaman it looks like 818.3 is missing ....)

 

[Nicole Seaman]

Hi David,

For 818.3, it is missing in the pdf.

Hello @samuelfu9999

Thank you for pointing out that 818.3 is missing. I've fixed it in the PDF and will upload the corrected version to the study planner. In the future, when you find any errors in the practice questions, please comment directly in the PQ forum thread, as this thread is just for study note and video errors. The PQ forum threads are on the answer pages in the PDF documents.

@David Harper CFA FRM

Can you also let me know if samuelfu9999 is correct about 818.1? There is some discussion about this in the original thread, but I want to make sure I'm understanding it correctly before I change anything.

For 818.1 of the Practice Questions of R27 (P.93), the d1 of rate of
change of the option price with respect to the futures price should be ln[F(0)/K] + σ^2*T/2 )/ [σ*sqrt(T)].

Thank you,

Nicole

 

[tattoo]

Hello David, in page 6 of R28-P1-T4: regarding the calculation of PV of 1 year bond

Why are there 2 "$100*0.75%/2*0.99658"? I think there's only 1 cash inflow of 100*0.75%/2 at time T=1.

 

[David Harper CFA FRM]

Hi @tattoo The final cash flow includes two components, the $100.00 par plus the coupon. The PV of the final cash flow is given by (100 + 100 × 0.75%/2) × 0.99135; i.e., the final (FV) cash flow is return of the $100.00 plus the coupon amount of 0.75%/2*100. However the formula nevertheless contains two typos (cc @Nicole Seaman this refers to R28 Study Notes page 6 ["Define the “law of one price”, explain it using an arbitrage argument, and describe how it can be applied to bond pricing"] such that the two indented (i.e., 2nd level) bullets should read:

• ($100.00 × 0.75%/2 × 0.99925) + ($100.00 × 0.75%/2 × 0.99648) + ($100.00 + $100.00 × 0.75%/2) × 0.99135 = $100.255, or
• [(0.75%/2 × 0.99925) + (0.75%/2 × 0.99648) + ((1 + 0.75%/2) × 0.99135)] * $100.00 = 1.00255 * $100.00 = $100.255

 

[tattoo]

In page 27 of R28-P1-T4, it says

However, the following example is

This means t=2.5, but the exponent is "2*0.5", which means t=0.5.

 

[David Harper CFA FRM]

@tattoo yea, it's confusing because the (t) has different roles: the exponent should be 2*Δt = 2*0.5 = 1.0, where Δt = 2.5 - 2.0; or the exponent can just be omitted altogether because it is just 1.0 in this case were we are retrieving a six-month forward rate under semi-annual compounding (our forward rate spans exactly one period). Although the logic isn't wrong. The second step is correct application if we understand the exponent, 2t, is really 2*Δt. I will modify this on later revision. Thanks,

 

[tattoo]

Hi David,
In page 7 of R31.P1.T4, there's an error in the following example.( PD=5.%, but PD in equation is 0.04)

 

[pbhalava]

Hi Team,
In Page 4 of P1.T4 (as shown in below image), won't the calculation of VAR be similar if we take P/L or L/P approach? In the example below we are getting different answers. If I am missing on the logic, please enlighten. Thank You!!

 

[David Harper CFA FRM]

Hi @pbhalava Yes, the use of L/P versus P/L should not change the VaR. In the pasted example above, the 95.0% 10-day absolute VaR = [-9.0%*(10/250) + 20.0%*sqrt(10/250)*1.645] * $100.00 = $6.22 regardless of L/P or P/L; in P/L, µ = 9.0% and in L/P, µ = -9.0% is the only input difference.

But as the text explains, the comparison above is between absolute VaR versus relative VaR: the relative VaR is 20.0%*sqrt(10/250)*1.645 * $100.00 = $6.58, which is greater (than absolute VaR) by exactly the drift because it omits the drift (and the relative VaR is just scaled volatility so it really doesn't care about L/P versus P/L). Relative VaR is the worst expected loss relative to the expected future value, and the expected future value is 9%*(10/250)*100 = $0.36 higher because that is the (positive) expected drift over the next ten days. The absolute VaR is less by the drift because it is the worst expected loss relative to today's position, so it's "giving credit" to the VaR for positive drift. The absolute VaR is the return-adjusted risk measure, is how I like to think about it; the relative VaR doesn't credit the drift (the return). So I don't see an error in the above, let me know if you still do? Thanks,

 

[pbhalava]

Hi @pbhalava Yes, the use of L/P versus P/L should not change the VaR. In the pasted example above, the 95.0% 10-day absolute VaR = [-9.0%*(10/250) + 20.0%*sqrt(10/250)*1.645] * $100.00 = $6.22 regardless of L/P or P/L; in P/L, µ = 9.0% and in L/P, µ = -9.0% is the only input difference.

But as the text explains, the comparison above is between absolute VaR versus relative VaR: the relative VaR is 20.0%*sqrt(10/250)*1.645 * $100.00 = $6.58, which is greater (than absolute VaR) by exactly the drift because it omits the drift (and the relative VaR is just scaled volatility so it really doesn't care about L/P versus P/L). Relative VaR is the worst expected loss relative to the expected future value, and the expected future value is 9%*(10/250)*100 = $0.36 higher because that is the (positive) expected drift over the next ten days. The absolute VaR is less by the drift because it is the worst expected loss relative to today's position, so it's "giving credit" to the VaR for positive drift. The absolute VaR is the return-adjusted risk measure, is how I like to think about it; the relative VaR doesn't credit the drift (the return). So I don't see an error in the above, let me know if you still do? Thanks,

Thank you for the explanation. I thought, the values coming in the green highlighted portion should be the same as calculated in the paragraph above. But yes, I got the gist of it.

 

[Elizabeth_Babalola]

Hi @David Harper CFA FRM ,

This was found on page 18, Chapter 1 of Topic 4- VRM study notes.

It seem more like a typo to me and should instead be: ES\[ \alpha \](X) + ES\[ \alpha \](Y).

Kindly reconfirm.

Thanks.

 

[Elizabeth_Babalola]

Hi @David Harper CFA FRM ,

This was found on page 18, Chapter 1 of Topic 4- VRM study notes.
View attachment 2690
It seem more like a typo to me and should instead be: ES\[ \alpha \](X) + ES\[ \alpha \](Y).

Kindly reconfirm.

Thanks.

Same as on page 19

 

[David Harper CFA FRM]

Hi @Elizabeth_Babalola Yes, you are correct, it should be: ES(X) + ES(Y) ≥ ES(X + Y). Thank you for spotting these typos! @Nicole Seaman I have saved correct version (in ROOT) to T4-VRM-1-Ch1-FR-Measures-v3.01 (but this can await next batch; i.e., let's without publish until we have more to edit)

 

[Elizabeth_Babalola]

Hi @David Harper CFA FRM ,

There seems to be some errors on page 32; Chapter 3 of the VRM note:




The return is 2% i.e 0.02 not 20% (0.2) as used in the calculations; kindly review.

Thanks.

Update: Fixed in v1.3

 

[Elizabeth_Babalola]

Also on page 9 of Topic 4 (same VRM). Taking a look at the below calculations, i am assuming that a negative sign was omitted the unconditional default probability formula.

 

[David Harper CFA FRM]

Hi @Elizabeth_Babalola Yes, you are correct about both mistakes. Thank you for your attention to detail. We will fix on revision. In regard to page 32 of VRM-3, the red circled (see below) 0.2s should be 0.020, just as you suggest. And in regard to page 9 of VRM-4, yes the negative sign was mistakenly omitted. Thank you again.