Roddefeller
New Member
Hi @David Harper CFA FRM,
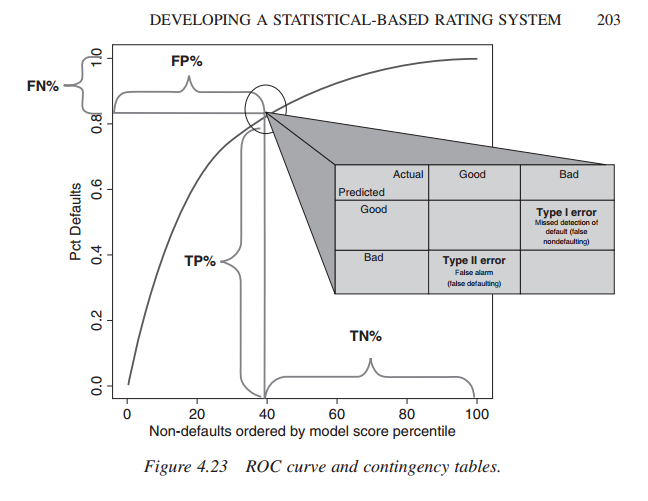
I was just reading through the "Validating Rating Models" Chapter and I'm left slightly confused with the definition De Laurentis uses for Type 1 and Type 2 errors with regard to the graph on p16 on the slides or p160 figure 10-5 in the original readings. What De Laurentis calls a Type 2 error (credit restriction for non-default), isn't this a clear case of a Type 1 error according to our understanding, as we reject a "good" one?
Many thanks for clarification.
Updated by Nicole to include graph that is being referenced:

I was just reading through the "Validating Rating Models" Chapter and I'm left slightly confused with the definition De Laurentis uses for Type 1 and Type 2 errors with regard to the graph on p16 on the slides or p160 figure 10-5 in the original readings. What De Laurentis calls a Type 2 error (credit restriction for non-default), isn't this a clear case of a Type 1 error according to our understanding, as we reject a "good" one?
Many thanks for clarification.
Updated by Nicole to include graph that is being referenced:

Last edited by a moderator: